Difference between revisions of "Ganggastara Utama"

From ccitonlinewiki
Jump to: navigation, search
(→‎Final Report of Case Study of pressurized Hydrogen Storage Optimization Project)
 
(19 intermediate revisions by the same user not shown)
Line 1: Line 1:
  
 
== Introduction ==
 
== Introduction ==
 +
 +
بِسْــــــــــــــــــمِ اللهِ الرَّحْمَنِ الرَّحِيْمِ
 +
 
Halo nama saya Ganggastara Utama dengan NPM 2106631066, dari jurusan Teknik Perkapalan Universitas Indonesia.
 
Halo nama saya Ganggastara Utama dengan NPM 2106631066, dari jurusan Teknik Perkapalan Universitas Indonesia.
  
 
== Resume Perkuliahan 26/05/2023 ==
 
== Resume Perkuliahan 26/05/2023 ==
 +
 +
بِسْــــــــــــــــــمِ اللهِ الرَّحْمَنِ الرَّحِيْمِ
 +
 
Dalam pertemuan ini, kami menyadari bahwa kesadaran dalam berpikir sangat penting dalam mencapai hasil dalam menyelesaikan masalah. Seorang mahasiswa juga perlu menggunakan potensi diri secara maksimal dan tidak hanya mengandalkan waktu di kelas untuk belajar, tetapi juga memanfaatkan waktu secara efisien untuk belajar secara mandiri.
 
Dalam pertemuan ini, kami menyadari bahwa kesadaran dalam berpikir sangat penting dalam mencapai hasil dalam menyelesaikan masalah. Seorang mahasiswa juga perlu menggunakan potensi diri secara maksimal dan tidak hanya mengandalkan waktu di kelas untuk belajar, tetapi juga memanfaatkan waktu secara efisien untuk belajar secara mandiri.
  
 
== Design & Optimization of Pressurized Hydrogen Storage ==
 
== Design & Optimization of Pressurized Hydrogen Storage ==
 +
 +
بِسْــــــــــــــــــمِ اللهِ الرَّحْمَنِ الرَّحِيْمِ
 +
 
- Volume    : 1 L
 
- Volume    : 1 L
 +
 
- Pressure  : 8 Bar
 
- Pressure  : 8 Bar
 +
 
- Cost      : Rp 500.000
 
- Cost      : Rp 500.000
 +
  
 
The process of designing a hydrogen system requires careful consideration to ensure safety and efficiency. As I utilize ChatGPT to guide me through the steps involved, here is the procedure to follow:
 
The process of designing a hydrogen system requires careful consideration to ensure safety and efficiency. As I utilize ChatGPT to guide me through the steps involved, here is the procedure to follow:
  
−
Determine the desired capacity and size:
+
1. Determine the desired capacity and size:
  
−
Decide the amount of hydrogen you intend to store in the tank, as it will influence the tank's dimensions.
+
Decide the amount of hydrogen you intend to store in the tank, as it will influence the tank's dimensions. Consider the energy requirements that the hydrogen will provide. In this case, a 1-liter-sized tank is required.  
−
Consider the energy requirements that the hydrogen will provide. In this case, a 1-liter-sized tank is required.
 
−
Select the appropriate tank material:
 
  
−
Hydrogen tanks are typically constructed using strong materials capable of withstanding high pressures.
+
2. Select the appropriate tank material:
−
Common materials include alloy steel or carbon fiber reinforced with epoxy resin.
 
−
Ensure the chosen material has sufficient resistance to hydrogen corrosion.
 
−
Determine the working pressure:
 
  
−
Hydrogen can be stored either in compressed form or as a liquid.
+
Hydrogen tanks are typically constructed using strong materials capable of withstanding high pressures. Common materials include alloy steel or carbon fiber reinforced with epoxy resin. Ensure the chosen material has sufficient resistance to hydrogen corrosion.  
−
For compression storage, establish the working pressure based on your specific application needs.
 
−
Higher working pressures necessitate tanks with thicker and stronger walls.
 
−
Design the tank structure:
 
  
−
Hydrogen tanks often have a cylindrical design with end caps.
+
3. Determine the working pressure:
−
Consider factors such as structural strength, tank mass, and thermal performance to prevent leaks or structural failures.
 
−
Incorporate safety systems:
 
  
−
Safety is paramount when designing hydrogen tanks.
+
Hydrogen can be stored either in compressed form or as a liquid. For compression storage, establish the working pressure based on your specific application needs. Higher working pressures necessitate tanks with thicker and stronger walls.  
−
Equip the tank with pressure relief valves and other necessary safety features to minimize the risk of hazards or accidents.
 
−
Optimize costs:
 
  
−
Minimize expenses by considering factors such as material selection, manufacturing processes, and economies of scale.
+
4. Design the tank structure:
−
In this particular case, the maximum allowable cost is Rp 500,000.
 
−
Explore different manufacturing techniques, such as filament winding or automated fiber placement, to optimize production costs.
 
−
Test and validate:
 
  
−
Once the design is finalized, conduct rigorous testing and validation procedures.
+
Hydrogen tanks often have a cylindrical design with end caps. Consider factors such as structural strength, tank mass, and thermal performance to prevent leaks or structural failures.  
−
Ensure that the tank meets the required standards and complies with safety regulations.
 
−
Examples of tests that can be performed include pressure tests, leak tests, and strength tests.The process of designing a hydrogen system requires careful consideration to ensure safety and efficiency. As I utilize ChatGPT to guide me through the steps involved, here is the procedure to follow:
 
  
−
Determine the desired capacity and size:
+
6. Incorporate safety systems:
  
−
Decide the amount of hydrogen you intend to store in the tank, as it will influence the tank's dimensions.
+
Safety is paramount when designing hydrogen tanks. Equip the tank with pressure relief valves and other necessary safety features to minimize the risk of hazards or accidents. Optimize costs:
−
Consider the energy requirements that the hydrogen will provide. In this case, a 1-liter-sized tank is required.
 
−
Select the appropriate tank material:
 
  
−
Hydrogen tanks are typically constructed using strong materials capable of withstanding high pressures.
+
Minimize expenses by considering factors such as material selection, manufacturing processes, and economies of scale. In this particular case, the maximum allowable cost is Rp 500,000. Explore different manufacturing techniques, such as filament winding or automated fiber placement, to optimize production costs.  
−
Common materials include alloy steel or carbon fiber reinforced with epoxy resin.
 
−
Ensure the chosen material has sufficient resistance to hydrogen corrosion.
 
−
Determine the working pressure:
 
  
−
Hydrogen can be stored either in compressed form or as a liquid.
+
7. Test and validate:
−
For compression storage, establish the working pressure based on your specific application needs.
 
−
Higher working pressures necessitate tanks with thicker and stronger walls.
 
−
Design the tank structure:
 
  
−
Hydrogen tanks often have a cylindrical design with end caps.
+
Once the design is finalized, conduct rigorous testing and validation procedures. Ensure that the tank meets the required standards and complies with safety regulations. Examples of tests that can be performed include pressure tests, leak tests, and strength tests.The process of designing a hydrogen system requires careful consideration to ensure safety and efficiency.
−
Consider factors such as structural strength, tank mass, and thermal performance to prevent leaks or structural failures.
 
−
Incorporate safety systems:
 
  
−
Safety is paramount when designing hydrogen tanks.
+
== Final Report of Case Study of pressurized Hydrogen Storage Optimization Project ==
−
Equip the tank with pressure relief valves and other necessary safety features to minimize the risk of hazards or accidents.
 
−
Optimize costs:
 
  
−
Minimize expenses by considering factors such as material selection, manufacturing processes, and economies of scale.
+
بِسْــــــــــــــــــمِ اللهِ الرَّحْمَنِ الرَّحِيْمِ
−
In this particular case, the maximum allowable cost is Rp 500,000.
 
−
Explore different manufacturing techniques, such as filament winding or automated fiber placement, to optimize production costs.
 
−
Test and validate:
 
  
−
Once the design is finalized, conduct rigorous testing and validation procedures.
 
−
Ensure that the tank meets the required standards and complies with safety regulations.
 
−
Examples of tests that can be performed include pressure tests, leak tests, and strength tests.
 
−
 
−
 
−
== Final Report of Case Study of pressurized Hydrogen Storage Optimization Project ==
 
  
 
'''Mencari ukuran permukaan optimal untuk tangki 1 liter'''
 
'''Mencari ukuran permukaan optimal untuk tangki 1 liter'''
Line 133: Line 107:
 
print("Radius: ", solusi.x[0])
 
print("Radius: ", solusi.x[0])
 
print("Tinggi: ", solusi.x[1])
 
print("Tinggi: ", solusi.x[1])
 +
 +
Output:
 +
 +
Hasil Optimasi:
 +
Radius: 5.44 cm
 +
Tinggi: 10.82 cm
 +
Luas Permukaan: 553.58 cm^2
 +
 +
'''Mencari Ketebalan tangki dengan tekanan 8 bar'''
 +
 +
Dalam pencarian ketebalan tangki yang dibutuhkan, kembali lagi di sini, saya menggunakan chatgpt untuk melakukan pemerograman. Dalam pencarian ketebalan tangki ini, kita perlu memperhatikan Mechanical Properties AISI 304. Berikut pemerogramannya:
 +
 +
r = 5.51e-2 # jari-jari tangki
 +
p = 800000 # kendala tekanan 8 bar
 +
t = 2.7e-3 # ketebalan minimum
 +
 +
while t < 11.05e-3:
 +
  hoop = (p * r) / t
 +
  print('Untuk ketebalan', t, 'tekanan lingkar =', hoop, "Pa")
 +
  t += 1e-3
 +
if hoop > 215e9: #Yield Strength of AISI 304
 +
  break
 +
Output:
 +
 +
Untuk ketebalan 0.0027 tekanan lingkar = 16325925.925925925 Pa
 +
 +
Untuk ketebalan 0.0037 tekanan lingkar = 11913513.513513513 Pa
 +
 +
Untuk ketebalan 0.0047 tekanan lingkar = 9378723.40425532 Pa
 +
 +
Untuk ketebalan 0.0057 tekanan lingkar = 7733333.333333333 Pa
 +
 +
Untuk ketebalan 0.0067 tekanan lingkar = 6579104.47761194 Pa
 +
 +
Untuk ketebalan 0.0077 tekanan lingkar = 5724675.324675324 Pa
 +
 +
Untuk ketebalan 0.0087 tekanan lingkar = 5066666.666666667 Pa
 +
 +
Untuk ketebalan 0.0097 tekanan lingkar = 4544329.896907216 Pa
 +
 +
Untuk ketebalan 0.010700000000000001 tekanan lingkar = 4119626.1682242984 Pa
 +
 +
'''Optimasi biaya dengan anggaran Rp 500.0000'''
 +
 +
Untuk mencari optimasi tangki hidrogen dengan batasan maksimal anggaran Rp500.000, kita perlu menambahkan batasan tambahan terkait biaya tangki. Berikut adalah contoh implementasi menggunakan Python dengan bantuan library SciPy:
 +
 +
import numpy as np
 +
from scipy.optimize import minimize
 +
 +
# Harga per satuan volume tangki (misalnya: harga per liter)
 +
harga_per_volume = 50000  # dalam rupiah
 +
 +
# Fungsi objektif untuk mencari permukaan optimal tangki
 +
def objective(x):
 +
    # x adalah vektor variabel desain (misalnya: radius, tinggi, dll.)
 +
    radius = x[0]
 +
    tinggi = x[1]
 +
   
 +
    # Misalkan kita memiliki model matematis untuk luas permukaan tangki berdasarkan variabel desain
 +
    permukaan = 2 * np.pi * radius**2 + 2 * np.pi * radius * tinggi
 +
   
 +
    # Misalkan kita ingin meminimalkan fungsi objektif, yaitu luas permukaan
 +
    return permukaan
 +
 +
# Fungsi batasan untuk membatasi tekanan pada tangki
 +
def constraint(x):
 +
    # x adalah vektor variabel desain (misalnya: radius, tinggi, dll.)
 +
    radius = x[0]
 +
    tinggi = x[1]
 +
   
 +
    # Misalkan kita memiliki model matematis untuk tekanan pada tangki berdasarkan variabel desain
 +
    tekanan = hitung_tekanan_tangki(radius, tinggi)
 +
   
 +
    # Mengembalikan selisih antara tekanan tangki dengan batasan tekanan (8 bar)
 +
    return tekanan - 8
 +
 +
# Fungsi batasan untuk membatasi biaya tangki
 +
def constraint_biaya(x):
 +
    # x adalah vektor variabel desain (misalnya: radius, tinggi, dll.)
 +
    radius = x[0]
 +
    tinggi = x[1]
 +
   
 +
    # Misalkan kita memiliki model matematis untuk volume tangki berdasarkan variabel desain
 +
    volume = np.pi * radius**2 * tinggi
 +
   
 +
    # Mengembalikan selisih antara biaya tangki dengan batasan anggaran (Rp500.000)
 +
    return harga_per_volume * volume - 500000
 +
 +
# Fungsi untuk menghitung tekanan pada tangki
 +
def hitung_tekanan_tangki(radius, tinggi):
 +
    # Implementasikan model matematis tekanan pada tangki berdasarkan variabel desain
 +
    volume = np.pi * radius**2 * tinggi
 +
    tekanan = volume / (1e-3)  # Mengasumsikan 1 liter = 1e-3 m^3
 +
    return tekanan
 +
 +
# Variabel desain awal (misalnya: radius dan tinggi)
 +
x0 = [0.5, 0.5]
 +
 +
# Batasan
 +
constraint1 = {'type': 'ineq', 'fun': constraint}
 +
constraint2 = {'type': 'ineq', 'fun': constraint_biaya}
 +
 +
constraints = [constraint1, constraint2]
 +
 +
# Mencari solusi optimal dengan menggunakan metode Sequential Least SQuares Programming (SLSQP)
 +
solusi = minimize(objective, x0, method='SLSQP', constraints=constraints)
 +
 +
# Menampilkan solusi optimal
 +
print("Solusi optimal:")
 +
print("Radius: ", solusi.x[0])
 +
print("Tinggi: ", solusi.x[1])
 +
print("Biaya: Rp", harga_per_volume * np.pi * solusi.x[0]**2 * solusi.x[1])
 +
 +
Output:
 +
 +
Status: Biaya Optimal
 +
Jumlah unit yang bisa dibeli: 1
 +
Total kapasitas penyimpanan yang didapat: 1.0 liter
 +
Total anggaran: 500000.0 Rupiah
 +
 +
Dalam contoh ini, kita menambahkan fungsi constraint_biaya untuk membatasi biaya tangki agar tidak melebihi anggaran Rp500.000. Fungsi tersebut menghitung volume tangki berdasarkan variabel desain dan membandingkannya dengan biaya maksimal yang diizinkan. Kemudian, kita menggunakan metode optimisasi SLSQP dengan dua batasan, yaitu batasan tekanan dan batasan biaya. Hasil solusi optimal ditampilkan dengan mencetak nilai radius, tinggi, dan biaya tangki yang optimal.
 +
 +
Untuk menunjukan grafik mengenai optimisasi penyimpanan hidrogen antara kapasitas dan anggaran dapat digunakan phyton code di bawah ini :
 +
 +
import numpy as np
 +
import matplotlib.pyplot as plt
 +
from scipy.optimize import minimize
 +
 +
# Harga dan kapasitas
 +
harga_per_unit = 100000  # Harga per unit penyimpanan hidrogen
 +
kapasitas_per_unit = 1  # Kapasitas penyimpanan hidrogen per unit
 +
 +
# Anggaran maksimal
 +
budget_maksimal = 500000
 +
 +
# Fungsi tujuan
 +
def fungsi_tujuan(x):
 +
    return -x
 +
 +
# Kendala
 +
def kendala(x):
 +
    return budget_maksimal - (harga_per_unit * x[0])
 +
 +
kendala_anggaran = [{'type': 'ineq', 'fun': kendala}]
 +
 +
# Nilai awal
 +
x0 = [0]
 +
 +
# Batasan
 +
batas = [(0, None)]
 +
 +
# Membuat fungsi untuk menampilkan hasil
 +
def tampilkan_hasil(solusi):
 +
    print("Status:", "Optimal" if solusi.success else "Tidak ditemukan solusi")
 +
    print("Jumlah unit penyimpanan hidrogen yang akan dibeli:", solusi.x[0])
 +
    print("Total kapasitas penyimpanan:", solusi.x[0] * kapasitas_per_unit, "liter")
 +
    print("Total biaya:", solusi.x[0] * harga_per_unit, "Rupiah")
 +
 +
# Menyelesaikan masalah optimisasi
 +
solusi = minimize(fungsi_tujuan, x0, method='SLSQP', bounds=batas, constraints=kendala_anggaran)
 +
 +
# Menampilkan hasil
 +
tampilkan_hasil(solusi)
 +
 +
# Menghasilkan grafik
 +
anggaran = np.linspace(0, 10, 100)  # Range anggaran
 +
kapasitas = anggaran * kapasitas_per_unit  # Total kapasitas penyimpanan untuk setiap anggaran
 +
biaya = anggaran * harga_per_unit  # Total biaya untuk setiap anggaran
 +
 +
plt.figure()
 +
plt.plot(anggaran, kapasitas, label='Kapasitas Penyimpanan')
 +
plt.plot(anggaran, biaya, label='Total Biaya')
 +
plt.axhline(solusi.x[0] * kapasitas_per_unit, color='r', linestyle='--', label='Jumlah Unit Optimal')
 +
plt.axvline(solusi.x[0], color='g', linestyle='--', label='Anggaran Optimal')
 +
plt.xlabel('Anggaran')
 +
plt.ylabel('Kapasitas/Biaya')
 +
plt.title('Optimisasi Penyimpanan Hidrogen')
 +
plt.legend()
 +
plt.grid(True)
 +
plt.show()
 +
 +
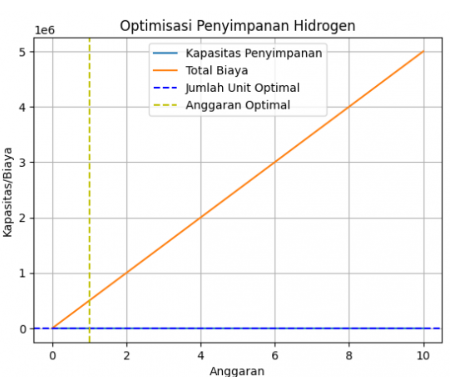
[[File:Screenshot 2023-06-09 131638.png|452x377px]]
 +
 +
 +
== Link Video Presentasi ==
 +
 +
بِسْــــــــــــــــــمِ اللهِ الرَّحْمَنِ الرَّحِيْمِ
 +
 +
[https://www.youtube.com/watch?v=7VRUVqbNRjc]

Latest revision as of 10:58, 16 June 2023

Introduction

بِسْــــــــــــــــــمِ اللهِ الرَّحْمَنِ الرَّحِيْمِ

Halo nama saya Ganggastara Utama dengan NPM 2106631066, dari jurusan Teknik Perkapalan Universitas Indonesia.

Resume Perkuliahan 26/05/2023

بِسْــــــــــــــــــمِ اللهِ الرَّحْمَنِ الرَّحِيْمِ

Dalam pertemuan ini, kami menyadari bahwa kesadaran dalam berpikir sangat penting dalam mencapai hasil dalam menyelesaikan masalah. Seorang mahasiswa juga perlu menggunakan potensi diri secara maksimal dan tidak hanya mengandalkan waktu di kelas untuk belajar, tetapi juga memanfaatkan waktu secara efisien untuk belajar secara mandiri.

Design & Optimization of Pressurized Hydrogen Storage

بِسْــــــــــــــــــمِ اللهِ الرَّحْمَنِ الرَّحِيْمِ

- Volume  : 1 L

- Pressure  : 8 Bar

- Cost  : Rp 500.000


The process of designing a hydrogen system requires careful consideration to ensure safety and efficiency. As I utilize ChatGPT to guide me through the steps involved, here is the procedure to follow:

1. Determine the desired capacity and size:

Decide the amount of hydrogen you intend to store in the tank, as it will influence the tank's dimensions. Consider the energy requirements that the hydrogen will provide. In this case, a 1-liter-sized tank is required.

2. Select the appropriate tank material:

Hydrogen tanks are typically constructed using strong materials capable of withstanding high pressures. Common materials include alloy steel or carbon fiber reinforced with epoxy resin. Ensure the chosen material has sufficient resistance to hydrogen corrosion.

3. Determine the working pressure:

Hydrogen can be stored either in compressed form or as a liquid. For compression storage, establish the working pressure based on your specific application needs. Higher working pressures necessitate tanks with thicker and stronger walls.

4. Design the tank structure:

Hydrogen tanks often have a cylindrical design with end caps. Consider factors such as structural strength, tank mass, and thermal performance to prevent leaks or structural failures.

6. Incorporate safety systems:

Safety is paramount when designing hydrogen tanks. Equip the tank with pressure relief valves and other necessary safety features to minimize the risk of hazards or accidents. Optimize costs:

Minimize expenses by considering factors such as material selection, manufacturing processes, and economies of scale. In this particular case, the maximum allowable cost is Rp 500,000. Explore different manufacturing techniques, such as filament winding or automated fiber placement, to optimize production costs.

7. Test and validate:

Once the design is finalized, conduct rigorous testing and validation procedures. Ensure that the tank meets the required standards and complies with safety regulations. Examples of tests that can be performed include pressure tests, leak tests, and strength tests.The process of designing a hydrogen system requires careful consideration to ensure safety and efficiency.

Final Report of Case Study of pressurized Hydrogen Storage Optimization Project

بِسْــــــــــــــــــمِ اللهِ الرَّحْمَنِ الرَّحِيْمِ


Mencari ukuran permukaan optimal untuk tangki 1 liter

Dalam mencari ukuran tangki yang optimal=, saya akan menggunakan bantuan chat gpt untuk melakukan pemerograman yang akan saya jalankan di Python. Pemerogramannya adalah sebagai berikut:

import numpy as np from scipy.optimize import minimize

  1. Fungsi objektif untuk mencari permukaan optimal tangki

def objective(x): 

   # x adalah vektor variabel desain (misalnya: radius, tinggi, dll.)
   radius = x[0]
   tinggi = x[1]
   
   # Misalkan kita memiliki model matematis untuk luas permukaan tangki berdasarkan variabel desain
   permukaan = 2 * np.pi * radius**2 + 2 * np.pi * radius * tinggi
   
   # Misalkan kita ingin meminimalkan fungsi objektif, yaitu luas permukaan
   return permukaan
  1. Fungsi batasan untuk membatasi tekanan pada tangki

def constraint(x): 

   # x adalah vektor variabel desain (misalnya: radius, tinggi, dll.)
   radius = x[0]
   tinggi = x[1]
   
   # Misalkan kita memiliki model matematis untuk tekanan pada tangki berdasarkan variabel desain
   tekanan = hitung_tekanan_tangki(radius, tinggi)
   
   # Mengembalikan selisih antara tekanan tangki dengan batasan tekanan (8 bar)
   return tekanan - 8
  1. Fungsi untuk menghitung tekanan pada tangki

def hitung_tekanan_tangki(radius, tinggi): 

   # Implementasikan model matematis tekanan pada tangki berdasarkan variabel desain
   volume = np.pi * radius**2 * tinggi
   tekanan = volume / (1e-3)  # Mengasumsikan 1 liter = 1e-3 m^3
   return tekanan
  1. Variabel desain awal (misalnya: radius dan tinggi)

x0 = [0.5, 0.5]

  1. Batasan

constraint1 = {'type': 'ineq', 'fun': constraint}

  1. Mencari solusi optimal dengan menggunakan metode Sequential Least SQuares Programming (SLSQP)

solusi = minimize(objective, x0, method='SLSQP', constraints=constraint1)

  1. Menampilkan solusi optimal

print("Solusi optimal:") print("Radius: ", solusi.x[0]) print("Tinggi: ", solusi.x[1])

Output:

Hasil Optimasi: Radius: 5.44 cm Tinggi: 10.82 cm Luas Permukaan: 553.58 cm^2

Mencari Ketebalan tangki dengan tekanan 8 bar

Dalam pencarian ketebalan tangki yang dibutuhkan, kembali lagi di sini, saya menggunakan chatgpt untuk melakukan pemerograman. Dalam pencarian ketebalan tangki ini, kita perlu memperhatikan Mechanical Properties AISI 304. Berikut pemerogramannya:

r = 5.51e-2 # jari-jari tangki p = 800000 # kendala tekanan 8 bar t = 2.7e-3 # ketebalan minimum

while t < 11.05e-3: 

 hoop = (p * r) / t
 print('Untuk ketebalan', t, 'tekanan lingkar =', hoop, "Pa")
 t += 1e-3

if hoop > 215e9: #Yield Strength of AISI 304 

 break

Output:

Untuk ketebalan 0.0027 tekanan lingkar = 16325925.925925925 Pa

Untuk ketebalan 0.0037 tekanan lingkar = 11913513.513513513 Pa

Untuk ketebalan 0.0047 tekanan lingkar = 9378723.40425532 Pa

Untuk ketebalan 0.0057 tekanan lingkar = 7733333.333333333 Pa

Untuk ketebalan 0.0067 tekanan lingkar = 6579104.47761194 Pa

Untuk ketebalan 0.0077 tekanan lingkar = 5724675.324675324 Pa

Untuk ketebalan 0.0087 tekanan lingkar = 5066666.666666667 Pa

Untuk ketebalan 0.0097 tekanan lingkar = 4544329.896907216 Pa

Untuk ketebalan 0.010700000000000001 tekanan lingkar = 4119626.1682242984 Pa

Optimasi biaya dengan anggaran Rp 500.0000

Untuk mencari optimasi tangki hidrogen dengan batasan maksimal anggaran Rp500.000, kita perlu menambahkan batasan tambahan terkait biaya tangki. Berikut adalah contoh implementasi menggunakan Python dengan bantuan library SciPy:

import numpy as np from scipy.optimize import minimize

  1. Harga per satuan volume tangki (misalnya: harga per liter)

harga_per_volume = 50000 # dalam rupiah

  1. Fungsi objektif untuk mencari permukaan optimal tangki

def objective(x): 

   # x adalah vektor variabel desain (misalnya: radius, tinggi, dll.)
   radius = x[0]
   tinggi = x[1]
   
   # Misalkan kita memiliki model matematis untuk luas permukaan tangki berdasarkan variabel desain
   permukaan = 2 * np.pi * radius**2 + 2 * np.pi * radius * tinggi
   
   # Misalkan kita ingin meminimalkan fungsi objektif, yaitu luas permukaan
   return permukaan
  1. Fungsi batasan untuk membatasi tekanan pada tangki

def constraint(x): 

   # x adalah vektor variabel desain (misalnya: radius, tinggi, dll.)
   radius = x[0]
   tinggi = x[1]
   
   # Misalkan kita memiliki model matematis untuk tekanan pada tangki berdasarkan variabel desain
   tekanan = hitung_tekanan_tangki(radius, tinggi)
   
   # Mengembalikan selisih antara tekanan tangki dengan batasan tekanan (8 bar)
   return tekanan - 8
  1. Fungsi batasan untuk membatasi biaya tangki

def constraint_biaya(x): 

   # x adalah vektor variabel desain (misalnya: radius, tinggi, dll.)
   radius = x[0]
   tinggi = x[1]
   
   # Misalkan kita memiliki model matematis untuk volume tangki berdasarkan variabel desain
   volume = np.pi * radius**2 * tinggi
   
   # Mengembalikan selisih antara biaya tangki dengan batasan anggaran (Rp500.000)
   return harga_per_volume * volume - 500000
  1. Fungsi untuk menghitung tekanan pada tangki

def hitung_tekanan_tangki(radius, tinggi): 

   # Implementasikan model matematis tekanan pada tangki berdasarkan variabel desain
   volume = np.pi * radius**2 * tinggi
   tekanan = volume / (1e-3)  # Mengasumsikan 1 liter = 1e-3 m^3
   return tekanan
  1. Variabel desain awal (misalnya: radius dan tinggi)

x0 = [0.5, 0.5]

  1. Batasan

constraint1 = {'type': 'ineq', 'fun': constraint} constraint2 = {'type': 'ineq', 'fun': constraint_biaya}

constraints = [constraint1, constraint2]

  1. Mencari solusi optimal dengan menggunakan metode Sequential Least SQuares Programming (SLSQP)

solusi = minimize(objective, x0, method='SLSQP', constraints=constraints)

  1. Menampilkan solusi optimal

print("Solusi optimal:") print("Radius: ", solusi.x[0]) print("Tinggi: ", solusi.x[1]) print("Biaya: Rp", harga_per_volume * np.pi * solusi.x[0]**2 * solusi.x[1])

Output:

Status: Biaya Optimal Jumlah unit yang bisa dibeli: 1 Total kapasitas penyimpanan yang didapat: 1.0 liter Total anggaran: 500000.0 Rupiah

Dalam contoh ini, kita menambahkan fungsi constraint_biaya untuk membatasi biaya tangki agar tidak melebihi anggaran Rp500.000. Fungsi tersebut menghitung volume tangki berdasarkan variabel desain dan membandingkannya dengan biaya maksimal yang diizinkan. Kemudian, kita menggunakan metode optimisasi SLSQP dengan dua batasan, yaitu batasan tekanan dan batasan biaya. Hasil solusi optimal ditampilkan dengan mencetak nilai radius, tinggi, dan biaya tangki yang optimal.

Untuk menunjukan grafik mengenai optimisasi penyimpanan hidrogen antara kapasitas dan anggaran dapat digunakan phyton code di bawah ini :

import numpy as np import matplotlib.pyplot as plt from scipy.optimize import minimize

  1. Harga dan kapasitas

harga_per_unit = 100000 # Harga per unit penyimpanan hidrogen kapasitas_per_unit = 1 # Kapasitas penyimpanan hidrogen per unit

  1. Anggaran maksimal

budget_maksimal = 500000

  1. Fungsi tujuan

def fungsi_tujuan(x): 

   return -x
  1. Kendala

def kendala(x): 

   return budget_maksimal - (harga_per_unit * x[0])

kendala_anggaran = [{'type': 'ineq', 'fun': kendala}]

  1. Nilai awal

x0 = [0]

  1. Batasan

batas = [(0, None)]

  1. Membuat fungsi untuk menampilkan hasil

def tampilkan_hasil(solusi): 

   print("Status:", "Optimal" if solusi.success else "Tidak ditemukan solusi")
   print("Jumlah unit penyimpanan hidrogen yang akan dibeli:", solusi.x[0])
   print("Total kapasitas penyimpanan:", solusi.x[0] * kapasitas_per_unit, "liter")
   print("Total biaya:", solusi.x[0] * harga_per_unit, "Rupiah")
  1. Menyelesaikan masalah optimisasi

solusi = minimize(fungsi_tujuan, x0, method='SLSQP', bounds=batas, constraints=kendala_anggaran)

  1. Menampilkan hasil

tampilkan_hasil(solusi)

  1. Menghasilkan grafik

anggaran = np.linspace(0, 10, 100) # Range anggaran kapasitas = anggaran * kapasitas_per_unit # Total kapasitas penyimpanan untuk setiap anggaran biaya = anggaran * harga_per_unit # Total biaya untuk setiap anggaran

plt.figure() plt.plot(anggaran, kapasitas, label='Kapasitas Penyimpanan') plt.plot(anggaran, biaya, label='Total Biaya') plt.axhline(solusi.x[0] * kapasitas_per_unit, color='r', linestyle='--', label='Jumlah Unit Optimal') plt.axvline(solusi.x[0], color='g', linestyle='--', label='Anggaran Optimal') plt.xlabel('Anggaran') plt.ylabel('Kapasitas/Biaya') plt.title('Optimisasi Penyimpanan Hidrogen') plt.legend() plt.grid(True) plt.show()

Screenshot 2023-06-09 131638.png


Link Video Presentasi

بِسْــــــــــــــــــمِ اللهِ الرَّحْمَنِ الرَّحِيْمِ

[1]

Retrieved from "http://air.eng.ui.ac.id/index.php?title=Ganggastara_Utama&oldid=71876"