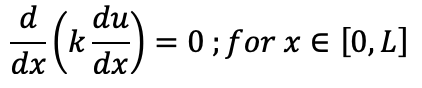Muhamad akbar fuad

Nama saya Muhamad Akbar Fuad, nama panggilannya Akbar Bidang peminatan yang diambil adalah Sistem Otomasi dan Manufaktur, dengan topik penelitian Additive Manufacturing Systems.

BELAJAR KOMPUTASI TEKNIK DENGAN CHAT GPT

DAI5

Conscious Thinking (Think about Reality and How to Solve it all) I(2) Intention I(3) Initial Thinking I(4) Idealization I(5) Instruction Set

JILID 1 : 1-Dimensional Partial Differential Equation (PDE)

Question 1: Did you know about DAI5?

The DAI5 Framework is a structured method designed to support systematic problem-solving, particularly in engineering and technical analysis. Developed by Dr. Ahmad Indra Siswantara, the DAI5 framework consists of five main steps: defining the problem, analyzing causes, ideating solutions, implementing, and evaluating outcomes. This approach is often applied in complex scenarios like numerical simulations, finite element analysis, and stress testing within engineering fields. By using DAI5, users aim to ensure each phase is rigorously analyzed to optimize solutions and outcomes, as demonstrated in various technical case studies on topics such as fluid dynamics and pipe stress analysis.

Question 2: Can you derive finite element equations from 1D PDE governing equations using weighted residual in a very simple way along with an example?

Certainly! Deriving the finite element equations from a 1D partial differential equation (PDE) using the weighted residual method is a great way to understand the basics of the finite element method (FEM). Let’s go through it step-by-step with a simple example.

Problem Setup: 1D PDE Example Consider the 1D steady-state heat conduction equation with no internal heat generation:

where: • u(x) is the temperature field, • k is the thermal conductivity (assumed constant for simplicity), • L is the length of the domain. Suppose we have the boundary conditions: 1. u(0)=u0 (a Dirichlet boundary condition), 2. du/dx = q at x = L (a Neumann boundary condition).

Step 1: Formulate the Weak Form (Weighted Residual Form) To derive the finite element equations, we start with the weak form of the governing equation. This involves: 1. Multiplying the differential equation by a test function v(x), 2. Integrating over the domain, 3. Applying integration by parts to reduce the order of derivatives on u. The weighted residual form is:

Question 1

How to scientifically justify that "conscious continuum" might suggest a continuous stream of awareness, free of interruption concept relevant to my work in consciousness and the DAI5 framework?

To scientifically justify the idea of a "conscious continuum" as a continuous, uninterrupted stream of awareness, particularly in relation to consciousness and the DAI5 framework, you might start with a few key points grounded in neuroscience, psychology, and philosophy:

1. **Neuroscientific Perspective on Continuous Perception**: Studies in neurobiology have shown that certain neural processes, particularly those involving the thalamus and cortex, operate in a way that appears to enable a continuous perception of the environment. This constant signaling aligns with a model of consciousness that isn’t strictly episodic but is rather fluid and ongoing. Research on gamma waves and the "binding problem" might support the view that brain processes naturally create a cohesive experience that feels uninterrupted.

2. **Philosophical Insights from Phenomenology**: Philosophers like Edmund Husserl have posited that consciousness is inherently a flowing experience, where even moments of pause or change in thought don’t break the underlying continuity. Husserl’s concept of the "stream of consciousness" supports the claim that awareness maintains a background consistency, even as specific focus shifts.

3. **The DAI5 Framework and Conscious States**: If the DAI5 framework considers different states or levels of consciousness, then the notion of continuity can be reinforced by viewing transitions between states not as interruptions, but as shifts within a single, cohesive framework. This would align with theories suggesting that even unconscious or subconscious processing contributes to an ongoing background awareness, providing a sort of seamless baseline.

4. **Psychological Research on Continuous Attention**: Experiments on sustained attention and working memory imply that humans have a baseline of uninterrupted processing. Even with distractions or lapses in specific focus, studies show a sort of persistent "attentional field" remains active. This suggests that conscious awareness, while sometimes distributed or partially engaged, might function as a continuous, adaptable field.

You could apply these ideas by examining how DAI5 interfaces with these concepts of continuity, particularly if DAI5 seeks to map or simulate continuous, holistic states. Drawing parallels with research in brain coherence, for example, might offer strong scientific support for the "conscious continuum" idea in your work.

Question 2

Can you give a practial example such as 1 Dimensional PDE problem?

We can think about the concept of a "conscious continuum" in the context of consciousness as analogous to how we model continuous phenomena in physics using Partial Differential Equations (PDEs).

Consider the **1-dimensional heat equation** as a practical example. This is a PDE that models the diffusion of heat (or any conserved quantity, like concentration) along a rod over time. Mathematically, it’s written as:

\[ \frac{\partial u}{\partial t} = \alpha \frac{\partial^2 u}{\partial x^2} \]

where: - \( u(x, t) \) represents the temperature at position \( x \) and time \( t \), - \( \alpha \) is the thermal diffusivity constant, - \( \frac{\partial u}{\partial t} \) is the rate of change of temperature over time, - \( \frac{\partial^2 u}{\partial x^2} \) is the spatial curvature of the temperature profile.

Analogy to Conscious Continuum In the context of a "conscious continuum," we could interpret this model as an analogy for how awareness or information "diffuses" across different states or parts of a conscious system:

1. **Spatial Domain as Conscious States**: If we view the spatial dimension \( x \) as representing different states or components within a conscious framework (like in the DAI5 model), then \( u(x, t) \) could represent the "level" of awareness or activation at each state.

2. **Temporal Evolution as Continuity of Awareness**: The equation suggests that awareness spreads out over time without interruption, smoothing out discrepancies and creating a cohesive state. This diffusion process would parallel how consciousness might "flow" or "spread" across different cognitive modules in a continuous manner.

3. **Boundary Conditions as Contextual Limits**: In a physical system, boundary conditions at the ends of the rod determine how the system behaves. Similarly, in a consciousness model, external inputs or internal constraints might define "boundaries" where the conscious experience changes or interacts with non-conscious processes.

Practical Example Imagine you want to model the focus of a conscious entity’s awareness as it shifts along a mental "axis" from one concept to another over time. The heat equation could be adapted to describe how this focus diffuses, creating a smooth transition in awareness without sudden breaks. In such a model: - \( u(x, t) \) could represent the level of focus on a given thought or concept at a particular time, gradually diffusing across adjacent concepts. - Any interruptions or drastic shifts in thought would be akin to introducing high gradients or sharp changes in the "awareness" field, requiring additional terms or boundary conditions.

This kind of 1D PDE framework could thus be useful for conceptualizing consciousness as a continuum that naturally evolves, smooths out, and sustains an ongoing "stream" of awareness.