Difference between revisions of "Jason Pandapotan Abraham (Metode Numerik)"
Panabraham (talk | contribs) (Created page with "Nama: Jason Pandapotan Abraham NPM: 2006523861 Hobi: Makan Saya adalah mahasiswa FTUI angkatan 2020 dari jurusan Teknik mesin dan saya adalah salah satu ciptaan terbaik da...") |
Panabraham (talk | contribs) |
||
| Line 43: | Line 43: | ||
| − | + | Berikut merupakan code optimasi tangki hidrogen dengan kapasitas 1 liter, dan biaya produksi maksimal Rp. 500.000 dengan material yang digunakan adalah AISI 316 austenitic stainless steel | |
| + | <syntaxhighlight lang=xml> | ||
| + | import numpy as np | ||
| + | from scipy.optimize import minimize | ||
| − | + | def objektif(x): | |
| − | + | # x[0] mewakili jari-jari, x[1] mewakili tinggi | |
| + | jari_jari = x[0] | ||
| + | tinggi = x[1] | ||
| − | + | # Hitung luas permukaan struktur silinder | |
| + | luas_permukaan = 2 * np.pi * jari_jari * (jari_jari + tinggi) | ||
| − | + | return luas_permukaan | |
| − | + | def konstrain(x): | |
| + | # x[0] mewakili jari-jari, x[1] mewakili tinggi | ||
| + | jari_jari = x[0] | ||
| + | tinggi = x[1] | ||
| − | + | # Hitung volume internal struktur silinder | |
| + | volume = np.pi * jari_jari**2 * tinggi | ||
| + | # Kembalikan selisih antara volume dan nilai yang diinginkan (1000 cm^3) | ||
| + | return volume - 1000 | ||
| + | # Menebak jari-jari dan tinggi | ||
| + | x0 = [1.0, 10.0] | ||
| − | + | # Membatasi variabel jari-jari dan tinggi | |
| + | batas = [(0, None), (0, None)] | ||
| − | + | # Menentukan konstrain dalam bentuk kamus | |
| − | + | konstrain_dict = {'type': 'eq', 'fun': konstrain} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | # Menggunakan fungsi minimize dari scipy untuk optimasi | |
| − | + | hasil = minimize(objektif, x0, method='SLSQP', bounds=batas, constraints=konstrain_dict) | |
| − | + | # Mencetak hasil yang telah dioptimasi | |
| − | + | print("Hasil Optimisasi:") | |
| + | print("Jari-jari: {:.2f} cm".format(hasil.x[0])) | ||
| + | print("Tinggi: {:.2f} cm".format(hasil.x[1])) | ||
| + | print("Luas Permukaan: {:.2f} cm^2".format(hasil.fun)) | ||
| − | + | </syntaxhighlight> | |
| − | |||
| − | + | Pertama mendefinisikan fungsi objektif yang menghitung luas permukaan struktur silinder berdasarkan variabel yang diberikan yakni tinggi dan jari-jari. | |
| − | + | Kemudian mendefinisikan fungsi konstrain yang memastikan volume internal struktur silinder memenuhi konstrain dengan selisih sebesar 1000 cm^3. | |
| + | Berikutnya, menebak tebakan awal untuk jari-jari dan tinggi (x0) serta batasan untuk variabel (batas). Dalam kasus ini, mengasumsikan jari-jari dan tinggi dapat memiliki nilai positif. | ||
| + | Selanjutnya, gunakan fungsi minimize dari scipy.optimize untuk menyelesaikan masalah optimisasi. Bemberikan fungsi objektif , metode (SLSQP), tebakan awal, konstrain, dan batasan. | ||
| + | Dari code tersebut didapat hasil: Tinggi: 10.84 cm, Jari-jari: 5.42 cm Luas Permukaan: 553.58 cm^2 | ||
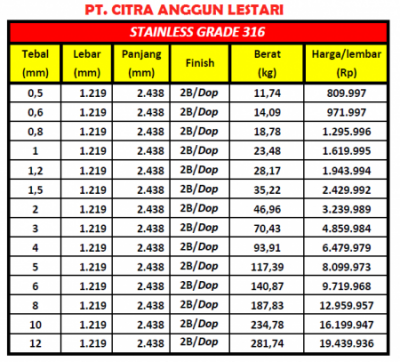
| − | + | [[File:400px-Pt_citra_anggun_kevin akbar.png]] | |
| − | + | Berdasarkan tabel diatas, kita dapat menghitung perkiraan harga dengan spesifikasi diatas (luas silinder = 571,88). Dengan membagi Harga dengan luas pelat, maka ditemukan biaya/satuan luas pelat. Setelah dihitung, dengan memaksimalkan budget, minimum berat yang masih masuk akal, dan strength masih wajar, saya memilih ketebalan 6mm untuk tank ini. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Revision as of 10:10, 6 June 2023
Nama: Jason Pandapotan Abraham
NPM: 2006523861
Hobi: Makan
Saya adalah mahasiswa FTUI angkatan 2020 dari jurusan Teknik mesin dan saya adalah salah satu ciptaan terbaik dari Tuhan yang Maha Esa karena pada prinsipnya Tuhan yang Maha Esa itu mendesain manusia dengan sebaik baiknya makhluk.
Contents
Tugas hydrogen storage Optimization
Hydrogen storage optimization involves maximizing the efficiency and capacity of hydrogen storage systems.We can solve that with :
1.Understand your storage requirements: Determine the specific needs for hydrogen storage, such as the required capacity, pressure, and temperature range. Consider the intended application and any constraints or limitations.
2.Choose the appropriate storage method: Evaluate different hydrogen storage methods, such as compressed gas, liquid hydrogen, metal hydrides, or chemical hydrides. Each method has its advantages and disadvantages in terms of capacity, energy density, safety, and cost.
3.Optimize storage system design: Consider factors such as storage vessel material, insulation, and pressure containment. Design the storage system to minimize leaks, ensure safety, and maximize capacity. Employ advanced materials and manufacturing techniques to enhance storage efficiency.
4.Optimize operating conditions: Explore the optimal pressure and temperature ranges for hydrogen storage based on the chosen storage method. Higher pressures and lower temperatures generally improve storage capacity, but they may have cost and safety implications. Conduct experiments or simulations to find the best operating conditions.
5.Improve storage materials: Research and develop new materials with higher hydrogen storage capacities and improved kinetics. For example, metal-organic frameworks (MOFs) and carbon-based materials like graphene have shown promise in enhancing hydrogen storage efficiency. Collaborate with researchers and experts in the field to explore cutting-edge materials.
6.Implement storage system monitoring and control: Install sensors and monitoring systems to ensure safe and efficient operation of the hydrogen storage system. Use real-time data to optimize storage conditions and detect any anomalies or leaks promptly.
7.Consider system integration: Account for the integration of hydrogen storage into larger systems, such as fuel cell vehicles or renewable energy storage systems. Optimize the interface between the storage system and the end-use application to maximize overall efficiency.
8.Conduct thorough safety assessments: Hydrogen storage optimization must prioritize safety. Perform rigorous safety assessments, including risk analysis, to identify potential hazards and implement appropriate safety measures. Adhere to industry standards and regulations to ensure safe storage and handling practices.
9.Continuously improve through research and development: Stay updated with advancements in hydrogen storage technologies and materials. Collaborate with researchers, universities, and industry experts to leverage their knowledge and expertise. Invest in research and development to continuously optimize and innovate hydrogen storage systems.
FINAL REPORT progress Case Study Optimization of Hydrogen Storage
Berikut merupakan code optimasi tangki hidrogen dengan kapasitas 1 liter, dan biaya produksi maksimal Rp. 500.000 dengan material yang digunakan adalah AISI 316 austenitic stainless steel
import numpy as np
from scipy.optimize import minimize
def objektif(x):
# x[0] mewakili jari-jari, x[1] mewakili tinggi
jari_jari = x[0]
tinggi = x[1]
# Hitung luas permukaan struktur silinder
luas_permukaan = 2 * np.pi * jari_jari * (jari_jari + tinggi)
return luas_permukaan
def konstrain(x):
# x[0] mewakili jari-jari, x[1] mewakili tinggi
jari_jari = x[0]
tinggi = x[1]
# Hitung volume internal struktur silinder
volume = np.pi * jari_jari**2 * tinggi
# Kembalikan selisih antara volume dan nilai yang diinginkan (1000 cm^3)
return volume - 1000
# Menebak jari-jari dan tinggi
x0 = [1.0, 10.0]
# Membatasi variabel jari-jari dan tinggi
batas = [(0, None), (0, None)]
# Menentukan konstrain dalam bentuk kamus
konstrain_dict = {'type': 'eq', 'fun': konstrain}
# Menggunakan fungsi minimize dari scipy untuk optimasi
hasil = minimize(objektif, x0, method='SLSQP', bounds=batas, constraints=konstrain_dict)
# Mencetak hasil yang telah dioptimasi
print("Hasil Optimisasi:")
print("Jari-jari: {:.2f} cm".format(hasil.x[0]))
print("Tinggi: {:.2f} cm".format(hasil.x[1]))
print("Luas Permukaan: {:.2f} cm^2".format(hasil.fun))
Pertama mendefinisikan fungsi objektif yang menghitung luas permukaan struktur silinder berdasarkan variabel yang diberikan yakni tinggi dan jari-jari.
Kemudian mendefinisikan fungsi konstrain yang memastikan volume internal struktur silinder memenuhi konstrain dengan selisih sebesar 1000 cm^3.
Berikutnya, menebak tebakan awal untuk jari-jari dan tinggi (x0) serta batasan untuk variabel (batas). Dalam kasus ini, mengasumsikan jari-jari dan tinggi dapat memiliki nilai positif.
Selanjutnya, gunakan fungsi minimize dari scipy.optimize untuk menyelesaikan masalah optimisasi. Bemberikan fungsi objektif , metode (SLSQP), tebakan awal, konstrain, dan batasan.
Dari code tersebut didapat hasil: Tinggi: 10.84 cm, Jari-jari: 5.42 cm Luas Permukaan: 553.58 cm^2
Berdasarkan tabel diatas, kita dapat menghitung perkiraan harga dengan spesifikasi diatas (luas silinder = 571,88). Dengan membagi Harga dengan luas pelat, maka ditemukan biaya/satuan luas pelat. Setelah dihitung, dengan memaksimalkan budget, minimum berat yang masih masuk akal, dan strength masih wajar, saya memilih ketebalan 6mm untuk tank ini.