Difference between revisions of "Fairuz Athaya Naim"
(→Final Report of Design & Optimization of Pressurized Hydrogen Storage) |
(→Final Report of Design & Optimization of Pressurized Hydrogen Storage) |
||
| Line 196: | Line 196: | ||
print(f"Optimal thickness: {best_thickness:.3f} mm") | print(f"Optimal thickness: {best_thickness:.3f} mm") | ||
| + | |||
print(f"Cost: Rp {best_cost}") | print(f"Cost: Rp {best_cost}") | ||
Revision as of 10:59, 9 June 2023
Contents
Introduction
Perkenalkan nama saya Fairuz Athaya Naim. Saya biasa dipanggil Aya. Saya lahir di Jakarta, 3 Desember 2002. Kini saya berdomisili di Depok. Saya mahasiswa kelas Metode Numerik-05.
Summary Pertemuan 1 (26/05/2023)
Pada pertemuan pertama mata kuliah Metode Numerik, saya mempelajari terkait pengertian Metode Numerik dan pengaplikasiannya dalam menyelesaikan permasalah matematika secara numerik. Kami juga mempelajari terkait consciousness dalam hidup, yaitu kesadaran manusia untuk menerima suatu permasalahan, memprosesnya, dan mencoba untuk mencari jalan keluar dari permasalahan tersebut. Di kelas kami juga mempelajari bahwa jawaban untuk hasil dari (X²-1)/(X-1) bila X = 1 adalah 2. Saat mensubstitusi langsung nilai x = 1 maka hasilnya akan 0/0 yang artinya jawaban tersebut tidak terdefinisikan. Tetapi bila dihitung menggunakan penjabaran dan penyederhanaan akan didapat hasil 2 meskipun hasil ini tidak belum absolut karena menggunakan nilai X = mendekati 1, hasil ini dapat didefinisikan dan tidak menimbulkan keraguan. Metode Numerik digunakan untuk melakukan perhitungan dan pendekatan yang akan menghasilkan nilai yang paling mendekati benar. Jawaban tersebut tidaklah eksak karena yang eksak hanyalah Tuhan Yang Maha Esa.
Design & Optimization of Pressurized Hydrogen Storage
Designing an optimizing pressurized hydrogen storage system with a 1-liter capacity and 8-bar pressure within a budget of Rp 500,000 requires careful consideration of materials and dimensions to optimize cost. I assembled the step-by-step of designing the storage with some help by ChatGPT
Selecting the Storage Tank Material
To optimize cost, it's important to choose an affordable and suitable material for the storage tank. One cost-effective option is using aluminum, as it offers good strength-to-weight ratio and corrosion resistance. Its dimensions will be determined in subsequent steps.
Determining the Tank Dimensions
To calculate the required dimensions, we need to consider the volume and pressure requirements. The given specifications are 1-liter capacity and 8-bar pressure. The formula to calculate the required tank volume is: Volume = Pressure * (1 liter / 8 bar) = 0.125 liters/bar.
Once the radius is calculated, the height can be determined using the 2:1 ratio: height = 2 * radius
Cost Optimization
To optimize costs, consider the following factors:
a. Material Cost: Aluminum is a cost-effective material for hydrogen storage tanks. Determine the cost of the required amount of aluminum based on current market prices.
b. Manufacturing Process: Opt for a simple manufacturing process that minimizes labor and machinery costs. Welding is commonly used for aluminum tanks, but consider the costs associated with different manufacturing methods.
c. Safety Measures: Ensure the tank design meets safety standards, minimizing the need for additional costly safety features.
d. Mass Production: If feasible, consider producing tanks in larger quantities, as it can reduce costs through economies of scale.
Cost Analysis
Once I have determined the material cost and considered the manufacturing and safety factors, analyze the overall cost. If the total cost exceeds the budget of Rp 500,000, I need to revisit the design and make adjustments to the dimensions, material, or manufacturing process to reduce costs.
Final Report of Design & Optimization of Pressurized Hydrogen Storage
Spesifikasi
Kapasitas: 1 Liter atau 1000 mL
Pressure: 8 bar
Biaya Maksimum: Rp 500,000
Material: Alumunium
Perhitungan Volume Tangki
1. Konversi Volume
1 Liter = 1000 cm^3
2. Konversi Tekanan
1 bar = 100,000 Pa
Tekanan Hidrogen = 8 x 100,000 Pa = 800,000 Pa
T = P = 800,000
A = 2πrh + πr^2
P = (2T) / A
sehingga
P = (2T) / (2πrh + πr^2)
800,000 = (2 x 800,000) / (2πrh + πr^2)
800,000 = 1,600,000 / (2πrh + πr^2)
800,000(2πrh + πr^2) = 1,600,000
2πrh + πr^2 = 2
2rh + r^2 = 2/π
Dengan h = 2r karena menyesuaikan dengan rasio 2:1
Untuk mencari nilai r, perlu dilakukan metode numerik Iterasi
Perhitungan Volume Tangki
Untuk perhitungan yang lebih sederhana
1000 = π x r^2 x h
Apabila mengasumsikan diameter 8.6 cm
1000 = π x 4.3^2 x h
1000/((π x 18.49))= h
Maka nilai h = 17.21 cm
Sehingga didapatkan untuk menampung 1 Liter Hydrogen pada tabung dengan diameter 8.6 cm diperlukan tinggi tabung sebesar 17.21 cm.
Untuk mencari luas permukaan menggunakan rumus
L = 2 x π x r (r + h)
L = 2 x π x 8.6 (8.6+17.21)
L = 1394.65 cm^2 = 13946 mm^2
Hasil perhitungan optimal karena telah memenuhi rasio perbandingan tinggi:radius sebesar 2:1
Pemilihan Material
Material yang digunakan untuk membuat tangki adalah Aluminium Alloy mempertimbangkan karakteristiknya yang fleksibel dan mudah dibentuk, serta tahan korosi sehingga tidak akan mencemari kemurnian hidrogen. Jenis yang akan digunakan adalah Aluminium Alloy 5052 dengan Yield Strength sebesar 193 MPa atau 27.99 ≈ 28 ksi. Allowable Strength didapat dari 2/3 dari Yield Strength yaitu 18.67 ksi atau 18670 psi
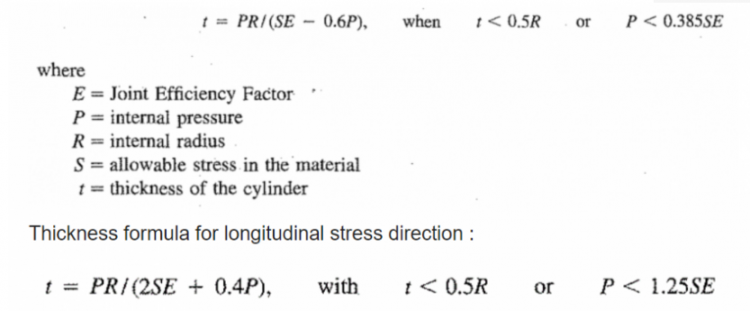
Ketebalan dari tabung dihitung melalui rumus:
E = 0.7
P = 8 bar = 116 psi
R = 8.6 cm = 3.385 inch
S = 18670 psi
Corrosion Allowance = 1 mm = 0.039 inch
t circumferential = 116 x (3.385 + 0.039) / (18670 x 0.7) - (0.6 x 116) = 0.0305 inch
0.0305 + corrosion allowance = 0.0305 + 0.039 = 0.0695 inch = 1.765 mm
t longitudinal = 116 x (3.385 + 0.039) / (2 x 18670 x 0.7) + (0.4 x 116) = 0.0152 Inch
0.0152 + corrosion allowance = 0.0152 + 0.039 = 0.0542 inch = 1.376 mm
Berdasarkan perhitungan diatas, maka minimal ketebalan bahannya adalah 1.765 mm
Cost Optimization
Melakukan cost optimization dengan phyton codes bila untuk membuat 1 liter aluminium tank with dengan radius 0.43 mm, tinggi 1.721 mm, lebar 1.765 mm, dan luas area 13946 mm^2. Harga Aluminium Alloy 5052 adalah is Rp 400 per 1000 mm^2 dan maximum cost sebesar Rp 500,000.
import math
def calculate_cost(thickness, area, price_per_area):
volume = area * thickness cost = math.ceil(volume / 1000) * price_per_area return cost
def optimize_cost(area, price_per_area, max_cost):
best_cost = float('inf')
best_thickness = 0.0
# Iterate over possible thickness values
for thickness in range(1, 100):
# Convert to millimeters
thickness_mm = thickness / 1000
# Calculate the cost for the current thickness
cost = calculate_cost(thickness_mm, area, price_per_area)
# Check if the cost is within the maximum allowed cost and update the best cost and thickness if necessary
if cost <= max_cost and cost < best_cost:
best_cost = cost
best_thickness = thickness_mm
return best_thickness, best_cost
- Given parameters
radius = 0.43
height = 1.721
width = 1.765
area = 13946
price_per_area = 400
max_cost = 500000
- Calculate the total area
total_area = 2 * math.pi * radius * height + math.pi * radius**2 + width * height
- Optimize the cost
best_thickness, best_cost = optimize_cost(total_area, price_per_area, max_cost)
print(f"Optimal thickness: {best_thickness:.3f} mm")
print(f"Cost: Rp {best_cost}")