Sasikirana Dania Putri

Introduction

My Name is Sasi and currently I'm very intreated in learning more about mechanical engineering !! ^O^/

Hydrogen Storage

In order to design and optimize a pressurized hydrogen tank (specifically an 8 bar storage with capacity of 1 liters and maximum budget of 500 thousand rupiah) there is a few things I've compiled in my head to do so, which includes;

1. Design the storage container with lightweight and high-strength materials, which are also strong and cost effective such as carbon fiber, aluminum, or other composite materials. This will help to reduce the weight and ensure that the container can withstand the pressure.

2. Consider using a pressure regulator to control the pressure of the hydrogen being stored. This will help to prevent over-pressurization and ensure safe storage. We'd also looks for affordable and yet reliable valves and pressure regulators that are suitable for hydrogen applications

3. Optimize the design of the valve and fitting system to ensure that they are compatible with the high-pressure hydrogen gas. This will help to prevent any leaks or other safety issues.

4. Use a pressure relief device to ensure that the container can release pressure in the event of an emergency. This is used for a safety feature, to make sure there is no burst when an over pressurization happens

5. Efficient Space Utilization: Design the storage system to fit in compact spaces, enabling easy integration into various applications, such as hydrogen-powered vehicles or portable energy systems.

6. Testing and Certification: Ensure that the storage system meets relevant safety standards and undergoes appropriate testing and certification processes to ensure its reliability and performance.

7. Cost-Effective Manufacturing: Explore cost-effective manufacturing methods, such as extrusion or casting, for the pressure vessel. These methods can help keep the production cost within the budget.

Calculating an Optimal Hydrogen Storage Tank

Beforehand, I’ve mentioned a few things we could focus on if we were to optimize a hydrogen tank; for instance I talked about the design of the container as well as the valve, manufacturing, materials, and encouraging users to use a few devices things such as a pressure regulator and relief. Now comes another question? As a mechanical engineer, we should not only be able to only think about how to optimize something, but to count the formulas as well as to design it.

When talking about a hydrogen storage tank, we first must think about the design; how large is it? How big is the length of the said tank? The optimal length and largeness, or volume, of a 1-liter hydrogen storage tank will depend on various factors, including the desired pressure rating, material strength, and safety considerations. In this case, with a maximum pressure of 8 bar, we can explore a general approach to estimating the tank dimensions.

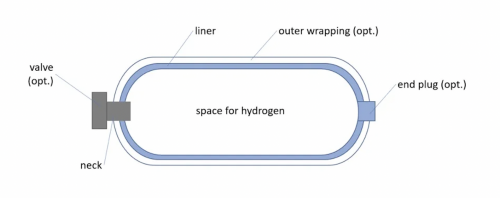
I've attached a picture below as an inspiration of what a hydrogen storage tank is commonly designed as

To begin, we need to determine the specific requirements for the tank's dimensions, such as the allowable stress in the tank material, safety factors, and design codes and standards. These factors will help ensure the tank's integrity and safety.

Assuming a cylindrical tank shape, we can use the following formula to estimate the required dimensions:

V = π * r^2 * h

where V is the desired volume (1 liter or 0.001 m³), π is a mathematical constant (approximately 3.14159), r is the radius, and h is the height of the tank.

To calculate the dimensions, we can rearrange the formula as follows:

h = V / (π * r^2)

Now, calculate the thickness of a hydrogen storage tank, you will need additional information, such as the material properties, safety factors, and design codes. However, the general outline of the calculation process would be the following;

1. Determine the desired volume: which is a 1-liter hydrogen storage tank.

2. Convert the pressure from bar to Pascals (Pa): 1 bar is approximately equal to 100,000 Pa. Therefore, the maximum pressure of 8 bar is equivalent to 800,000 Pa.

3. Determine the material properties: The specific material you choose for the tank will have a maximum allowable stress value (σ_allowable). This value represents the stress that the material can handle without experiencing permanent deformation or failure.

4. Apply safety factors: Safety factors are used to account for uncertainties and ensure the tank's structural integrity and safety. Typical safety factors range between 2 to 4, depending on the design standards and regulations.

5. Calculate the required thickness: Using the formula for the cylindrical pressure vessel's wall thickness, you can determine the thickness (t) needed to withstand the pressure:

t = (P * r) / (σ_allowable * SF)

where P is the pressure in Pascals, r is the radius of the tank, σ_allowable is the allowable stress of the material, and SF is the safety factor.