Difference between revisions of "Muhammad Hasfi Rizki Nur"
(→Pertemuan 4 (2 Desember 2020)) |
(→Pertemuan 4 (2 Desember 2020)) |
||
| Line 591: | Line 591: | ||
== Pertemuan 4 (2 Desember 2020) == | == Pertemuan 4 (2 Desember 2020) == | ||
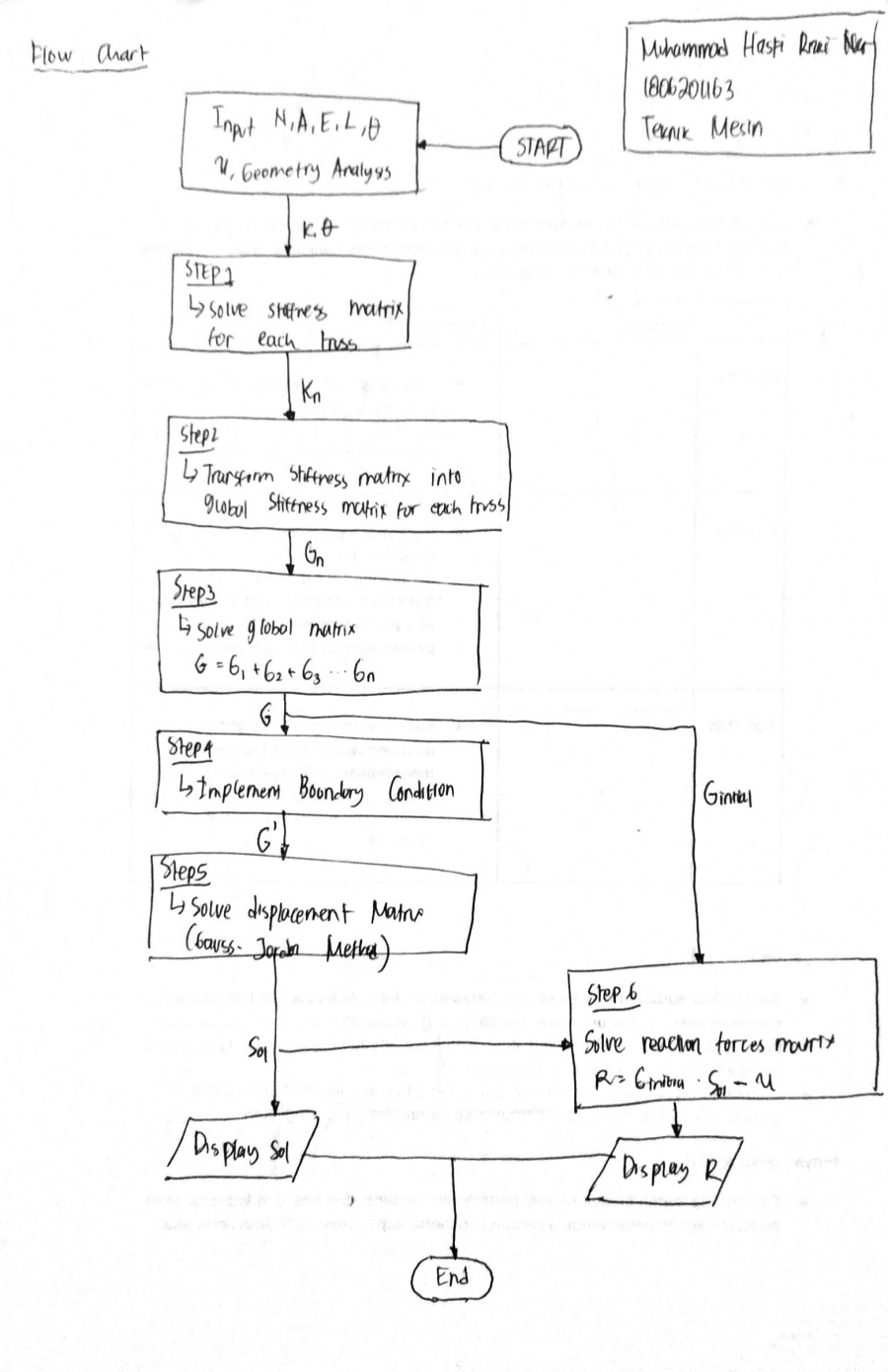
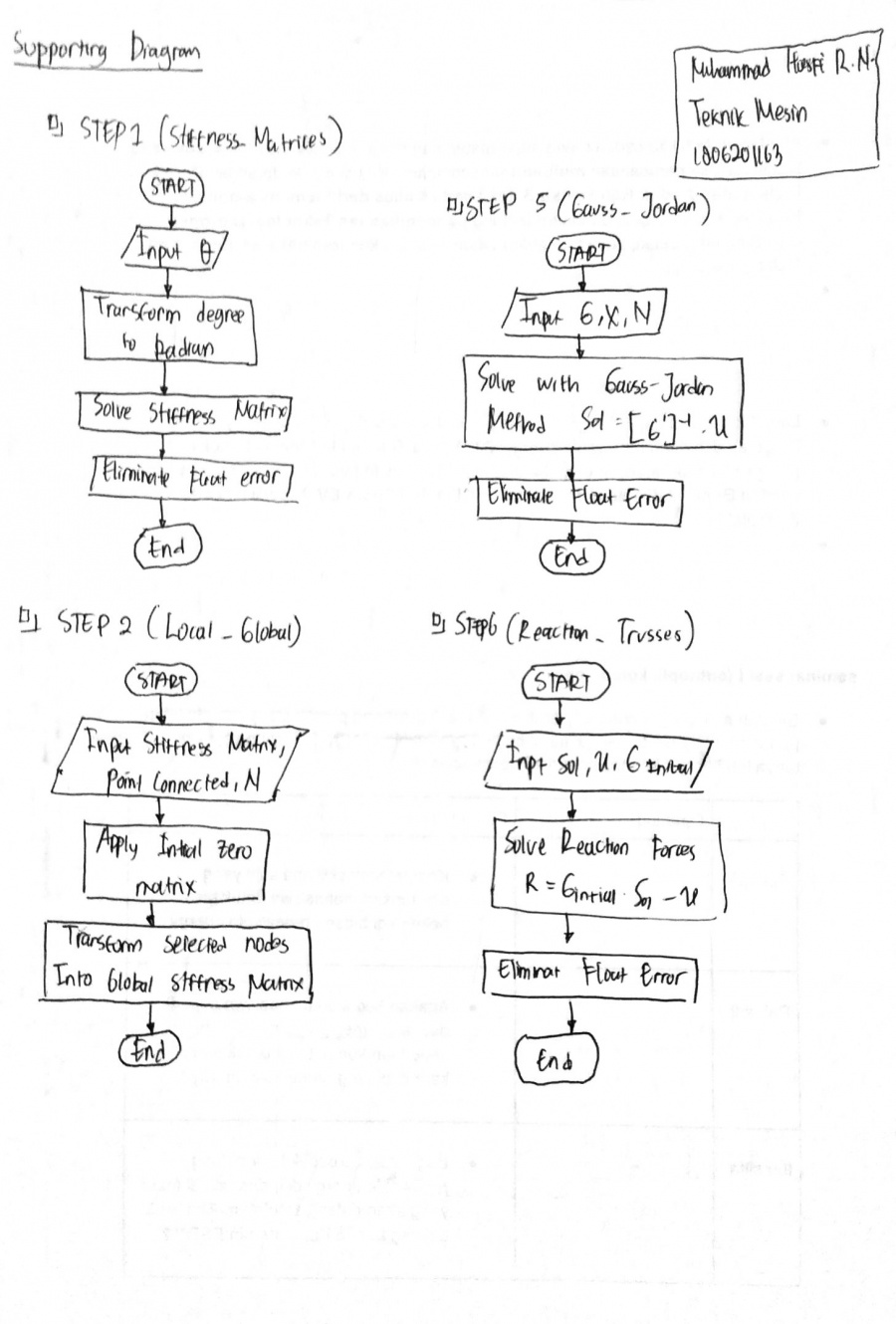
| − | '''Tugas''' | + | === '''Kuis: Membuat Flowchart dan Diagram''' === |
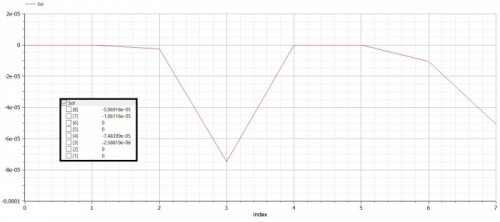
| + | [[File:hasfi_1_kuis.jpg|900px|left]] | ||
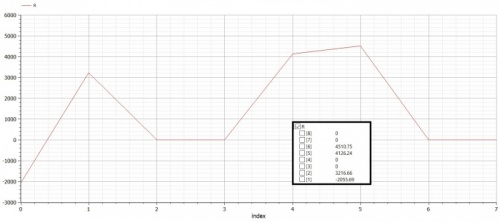
| + | [[File:hasfi_2_kuis.jpg|900px|left]] | ||
| + | [[File:hasfi_3_kuis.jpg|900px|left]] | ||
| + | |||
| + | === '''Tugas''' === | ||
[[File:hasfi_soal_4.jpg|500px|left]] | [[File:hasfi_soal_4.jpg|500px|left]] | ||
[[File:hasfi_1_p4.jpg|900px|left]] | [[File:hasfi_1_p4.jpg|900px|left]] | ||
Revision as of 16:43, 9 December 2020
بِسْمِ اللهِ الرَّحْمَنِ الرَّحِيْمِ
السَّلاَمُ عَلَيْكُمْ وَرَحْمَةُ اللهِ وَبَرَكَاتُ
Biodata Diri
Nama: Muhammad Hasfi Rizki Nur
NPM : 1806201163
TTL : Jakarta, 26 Oktober 2000
Saya mahasiswa prodi Teknik Mesin Universitas Indonesia angkatan 2018. Saat ini saya sedang menempuh kuliah pada semester kelima.
Alasan saya memilih prodi ini adalah saya tertarik belajar dan bekerja di bidang energi dan ingin memberikan dampak yang baik kepada perkembangan teknologi energi di Indonesia.
Contents
Pertemuan 1 (11 November 2020)
Materi pertemuan pertama
Pada pertemuan pertama, Bapak Ahmad Indra memberikan penjelasan bagaiman mengoperasikan situs wiki air dan memberikan 4 poin tujuan pembelajaran Metode Numerik saat ini. Keempat poin tersebut adalah:
- Mempelajari dan memahami konsep dasar dari pelajaran Metode Numerik.
- Mampu menerapkan pemahaman konsep kedalam permodelan numerik.
- Mengaplikasikan metode numerik dengan output yang berasal dari persoalan seputar Teknik Mesin.
- Mengevaluasi diri sendiri sehingga menjadi orang yang lebih beradab sesuai Sila kedua dari Pancasila.
Tugas Video
Diberikan tugas berupa menjelaskan perangkat lunak OpenModelica dan pengoperasikannya. Berikut video yang sudah saya buat:
Pertemuan 2 (18 November 2020)
Pada kegiatan pembelajaran mata kuliah metode numerik hari ini. Bapak Ahmad Indra mengawali dengan mereview tugas yang diberikan pada pertemuan minggu lalu. Review tersebut mengenai software Open Modelica dan perhitungan yang masing - masing mahasiswa lakukan.
Berikut ini adalah contoh penerapan aplikasi OpenModelica untuk membuat 4 algoritma metode numerik dalam mencari roots of equation (akar persamaan) dari:
f(x) = exp^(-x)-(x)
f'(x) = -exp^(-x)-1
error maksimum = 0.0000001
1) Newton Raphson (Terbuka)
model Newton_Raphson_Algorithm parameter Real g=1; //guess parameter Integer N=20; //max iteration parameter Real er=0.0000001; //error maximum Real a[N]; Real y[N];//function Real ER[N]; //error Real sol; //solution algorithm a[1]:=g; y[1]:=a[1]-(exp(-a[1])-a[1])/(-exp(-a[1])-1); ER[1]:=abs(1-a[1]/y[1]); for i in 2:N loop a[i]:=y[i-1]; y[i]:=a[i]-(exp(-a[i])-a[i])/(-exp(-a[i])-1); ER[i]:=abs(1-y[i-1]/y[i]); if ER[i]<er then sol:=y[i]; break; end if; end for; end Newton_Raphson_Algorithm;
2) Secant (Terbuka)
model Secant_Algorithm parameter Real a=0; //guess parameter Real b=1; //guess parameter Integer N=10; //max iteration parameter Real er=0.0000001; //error maximum Real A[N]; Real B[N]; Real y[N]; Real ER[N]; Real sol; //solution algorithm A[1]:=a; B[1]:=b; y[1]:=B[1]-(exp(-B[1])-B[1])*(A[1]-B[1])/((exp(-A[1])-A[1])-(exp(-B[1])-B[1])); ER[1]:=abs(1-B[1]/y[1]); for i in 2:N loop A[i]:=B[i-1]; B[i]:=y[i-1]; y[i]:=B[i]-(exp(-B[i])-B[i])*(A[i]-B[i])/((exp(-A[i])-A[i])-(exp(-B[i])-B[i])); ER[i]:=abs(1-y[i-1]/y[i]); if ER[i]<er then sol:=y[i]; break; end if; end for; end Secant_Algorithm;
3) Bisection (Tertutup)
model Bisection_Algorithm parameter Real a=0; //guess bawah parameter Real b=1; //guess atas parameter Integer N=50; //max iteration parameter Real er=0.0000001; //error maximum Real fa=(exp(-a)-a); Real fb=(exp(-b)-b); Real A[N]; Real B[N]; Real fy[N]; Real y[N]; Real ER[N]; Real sol; //solution algorithm if fa*fb<0 then A[1]:=a; B[1]:=b; y[1]:=(A[1]+B[1])/2; fy[1]:=exp(-y[1])-y[1]; ER[1]:=1; for i in 2:N loop if fy[i-1]>0 then A[i]:=y[i-1]; B[i]:=B[i-1]; else A[i]:=A[i-1]; B[i]:=y[i-1]; end if; y[i]:=(A[i]+ B[i])/2; fy[i]:=exp(-y[i])-y[i]; ER[i]:=abs(1-y[i-1]/y[i]); if ER[i]<er then sol:=y[i]; break; end if; end for; end if; end Bisection_Algorithm;
4) Regula Falsi (Tertutup)
model Regula_Falsi_Algorithm parameter Real a=0; //guess bawah parameter Real b=1; //guess atas parameter Integer N=20; //max iteration parameter Real er=0.0000001; //error maximum Real A[N]; Real B[N]; Real fa[N]; Real fb[N]; Real fy[N]; Real y[N]; Real ER[N]; Real sol; //solution algorithm A[1]:=a; B[1]:=b; fa[1]:=exp(-A[1])-A[1]; fb[1]:=exp(-B[1])-B[1]; if fa[1]*fb[1]<0 then y[1]:=(A[1]*fb[1]-B[1]*fa[1])/(fb[1]-fa[1]); fy[1]:=exp(-y[1])-y[1]; ER[1]:=1; for i in 2:N loop if fy[i-1]>0 then A[i]:=y[i-1]; B[i]:=B[i-1]; else A[i]:=A[i-1]; B[i]:=y[i-1]; end if; fa[i]:=exp(-A[i])-A[i]; fb[i]:=exp(-B[i])-B[i]; y[i]:=(A[i]*fb[i]-B[i]*fa[i])/(fb[i]-fa[i]); fy[i]:=exp(-y[i])-y[i]; ER[i]:=abs(1-y[i-1]/y[i]); if ER[i]<er then sol:=y[i]; break; end if; end for; end if; end Regula_Falsi_Algorithm;
Tugas Video
Berikut video yang sudah saya buat: https://youtu.be/Q8-usKi9NsM
Pertemuan 3 (25 November 2020)
Pada awal-awal Pak Dai memaparkan tiga aplikasi metode numerik yang sering digunakan dalam menyelesaikan permasalahan teknik, pertama ada Computation Fluid Dynamics (CFD), lalu FInite Element Analysis (FEA), dan Metode Stokastik. CFD dan FEA berbasis ilmu fisika, kemudian metode stokastik berbasis data dan statistik. Ada lima langkah yang Pak Dai paparkan dalam mengaplikasikan metode numerik ke permasalahan teknik :
- Riset masalah tekniknya terlebih dahulu
- Menganalisis masalah (mendefinisikan variabel yang mau dicari dan mencari parameter fisikanya)
- Membuat model matematika
- Membuat model numerik
- Setelah itu cari penyelesaian dengan bantuan komputer untuk mendapatkan output yang diinginkan
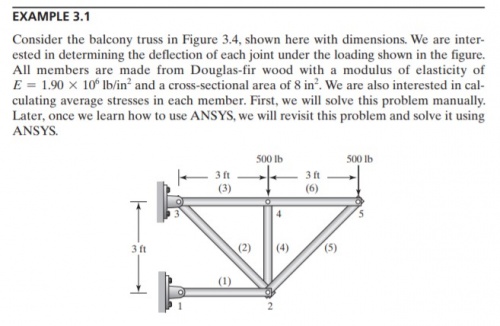
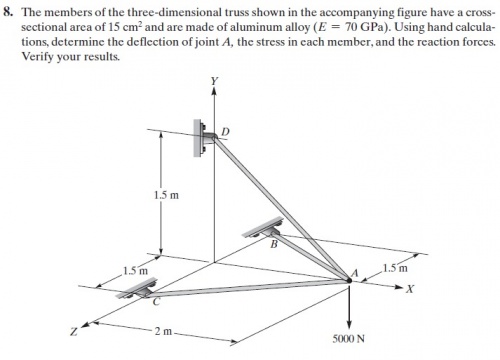
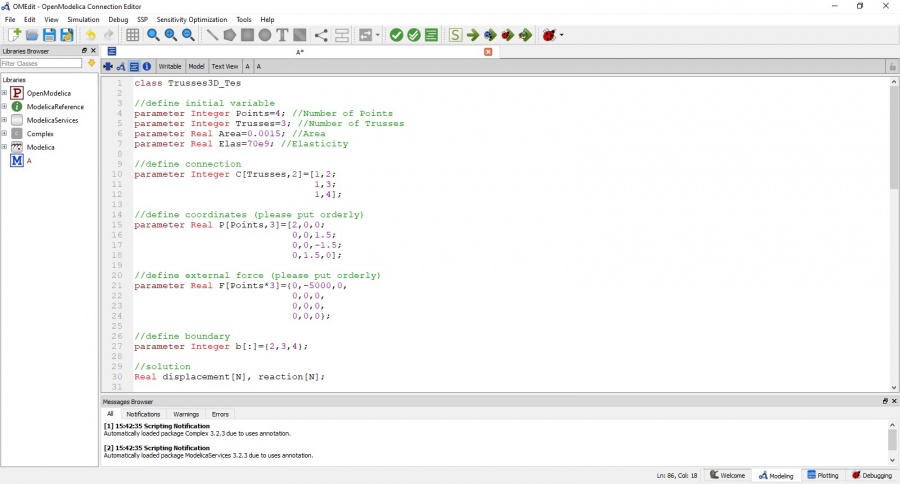
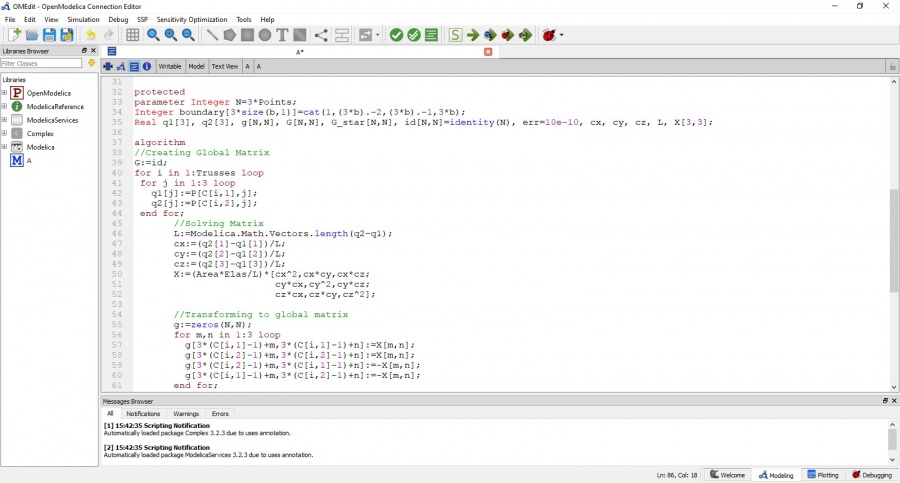
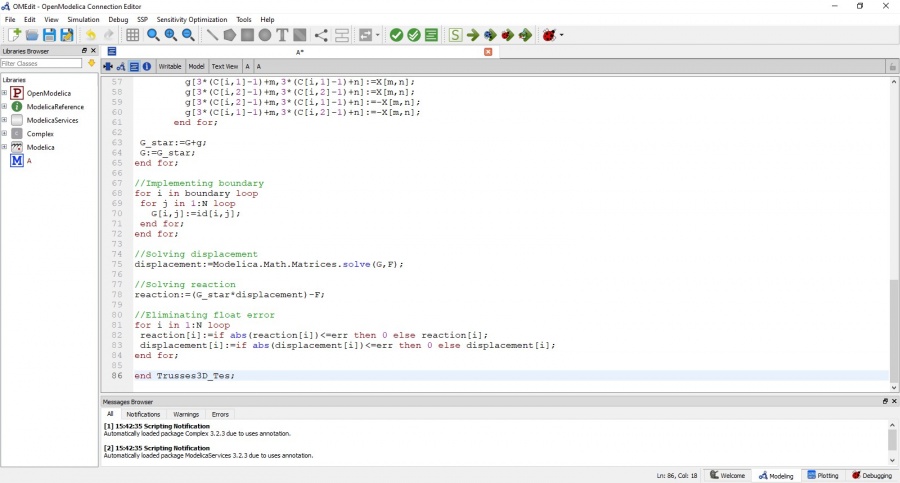
Soal Latihan Trusses Problem
Code
|
Persamaan model Trusses
parameter Integer N=10; //Global matrice = 2*points connected
parameter Real A=8;
parameter Real E=1.9e6;
Real G[N,N]; //global
Real Ginitial[N,N]; //global
Real Sol[N]; //global dispplacement
Real X[N]={0,0,0,0,0,0,0,-500,0,-500};
Real R[N]; //global reaction force
Real SolMat[N,1];
Real XMat[N,1];
//boundary coundition
Integer b1=1;
Integer b2=3;
//truss 1
parameter Real X1=0; //degree between truss
Real k1=A*E/36;
Real K1[4,4]; //stiffness matrice
Integer p1a=1;
Integer p1b=2;
Real G1[N,N];
//truss 2
parameter Real X2=135; //degree between truss
Real k2=A*E/50.912;
Real K2[4,4]; //stiffness matrice
Integer p2a=2;
Integer p2b=3;
Real G2[N,N];
//truss 3
parameter Real X3=0; //degree between truss
Real k3=A*E/36;
Real K3[4,4]; //stiffness matrice
Integer p3a=3;
Integer p3b=4;
Real G3[N,N];
//truss 4
parameter Real X4=90; //degree between truss
Real k4=A*E/36;
Real K4[4,4]; //stiffness matrice
Integer p4a=2;
Integer p4b=4;
Real G4[N,N];
//truss 5
parameter Real X5=45; //degree between truss
Real k5=A*E/50.912;
Real K5[4,4]; //stiffness matrice
Integer p5a=2;
Integer p5b=5;
Real G5[N,N];
//truss 6
parameter Real X6=0; //degree between truss
Real k6=A*E/36;
Real K6[4,4]; //stiffness matrice
Integer p6a=4;
Integer p6b=5;
Real G6[N,N];
/*
for each truss, please ensure pXa is lower then pXb (X represents truss element number)
*/
algorithm
//creating global matrice
K1:=Stiffness_Matrices(X1);
G1:=k1*Local_Global(K1,N,p1a,p1b);
K2:=Stiffness_Matrices(X2);
G2:=k2*Local_Global(K2,N,p2a,p2b);
K3:=Stiffness_Matrices(X3);
G3:=k3*Local_Global(K3,N,p3a,p3b);
K4:=Stiffness_Matrices(X4);
G4:=k4*Local_Global(K4,N,p4a,p4b);
K5:=Stiffness_Matrices(X5);
G5:=k5*Local_Global(K5,N,p5a,p5b);
K6:=Stiffness_Matrices(X6);
G6:=k6*Local_Global(K6,N,p6a,p6b);
G:=G1+G2+G3+G4+G5+G6;
Ginitial:=G;
//implementing boundary condition
for i in 1:N loop
G[2*b1-1,i]:=0;
G[2*b1,i]:=0;
G[2*b2-1,i]:=0;
G[2*b2,i]:=0;
end for;
G[2*b1-1,2*b1-1]:=1;
G[2*b1,2*b1]:=1;
G[2*b2-1,2*b2-1]:=1;
G[2*b2,2*b2]:=1;
//solving displacement
Sol:=Gauss_Jordan(N,G,X);
//solving reaction force
SolMat:=matrix(Sol);
XMat:=matrix(X);
R:=Reaction_Trusses(N,Ginitial,SolMat,XMat);
end Trusses;
|
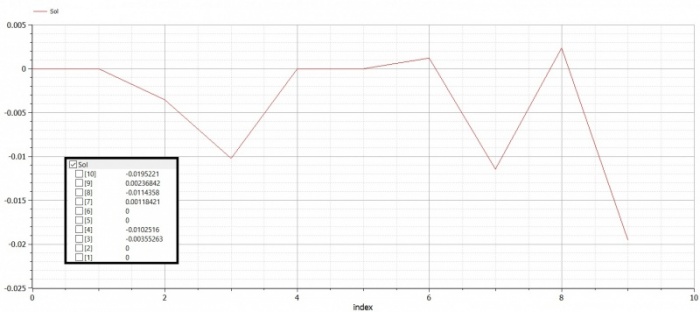
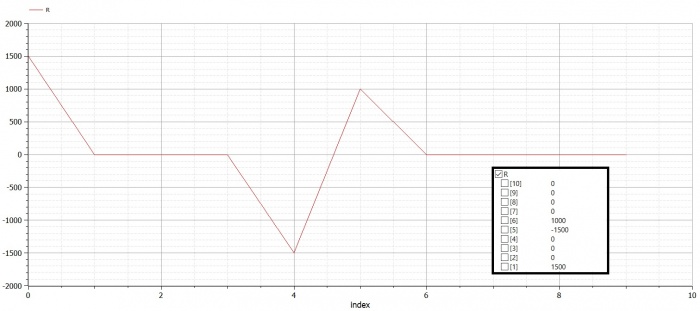
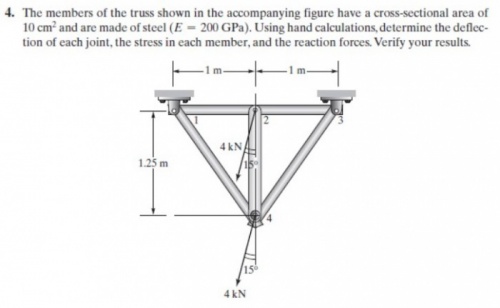
Tugas Trusses Problem
code
|
Persamaan class Trusses_HW
parameter Integer N=8; //Global matrice = 2*points connected
parameter Real A=0.001; //Area m2
parameter Real E=200e9; //Pa
Real G[N,N]; //global
Real Ginitial[N,N]; //global
Real Sol[N]; //global dispplacement
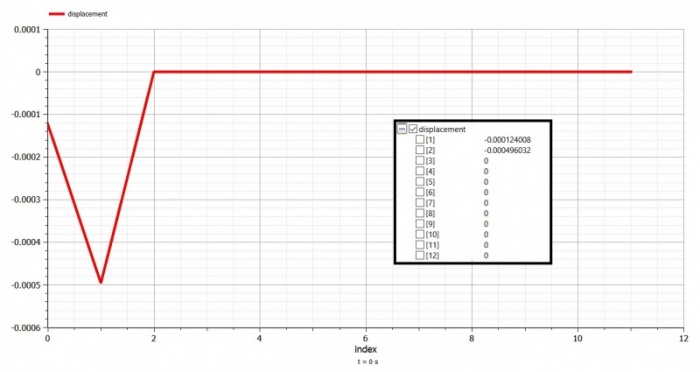
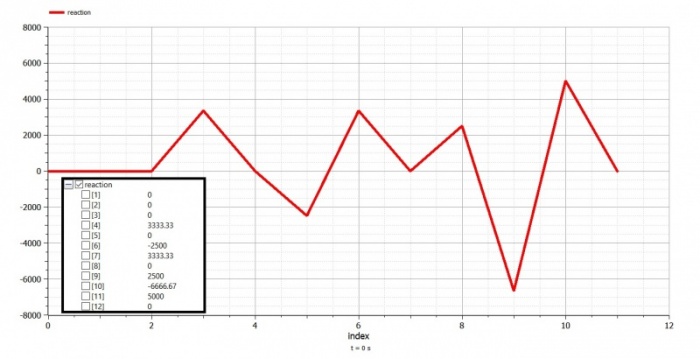
Real X[N]={0,0,-1035.2762,-3863.7033,0,0,-1035.2762,-3863.7033};
Real R[N]; //global reaction force
Real SolMat[N,1];
Real XMat[N,1];
//boundary condition
Integer b1=1;
Integer b2=3;
//truss 1
parameter Real X1=0; //degree between truss
Real k1=A*E/1;
Real K1[4,4]; //stiffness matrice
Integer p1a=1;
Integer p1b=2;
Real G1[N,N];
//truss 2
parameter Real X2=0; //degree between truss
Real k2=A*E/1;
Real K2[4,4]; //stiffness matrice
Integer p2a=2;
Integer p2b=3;
Real G2[N,N];
//truss 3
parameter Real X3=90; //degree between truss
Real k3=A*E/1.25;
Real K3[4,4]; //stiffness matrice
Integer p3a=2;
Integer p3b=4;
Real G3[N,N];
//truss 4
parameter Real X4=90+38.6598; //degree between truss
Real k4=A*E/1.6;
Real K4[4,4]; //stiffness matrice
Integer p4a=1;
Integer p4b=4;
Real G4[N,N];
//truss 5
parameter Real X5=90-38.6598; //degree between truss
Real k5=A*E/1.6;
Real K5[4,4]; //stiffness matrice
Integer p5a=3;
Integer p5b=4;
Real G5[N,N];
/*
for each truss, please ensure pXa is lower then pXb (X represents truss element number)
*/
algorithm
//creating global matrice
K1:=Stiffness_Matrices(X1);
G1:=k1*Local_Global(K1,N,p1a,p1b);
K2:=Stiffness_Matrices(X2);
G2:=k2*Local_Global(K2,N,p2a,p2b);
K3:=Stiffness_Matrices(X3);
G3:=k3*Local_Global(K3,N,p3a,p3b);
K4:=Stiffness_Matrices(X4);
G4:=k4*Local_Global(K4,N,p4a,p4b);
K5:=Stiffness_Matrices(X5);
G5:=k5*Local_Global(K5,N,p5a,p5b);
G:=G1+G2+G3+G4+G5;
Ginitial:=G;
//implementing boundary condition
for i in 1:N loop
G[2*b1-1,i]:=0;
G[2*b1,i]:=0;
G[2*b2-1,i]:=0;
G[2*b2,i]:=0;
end for;
G[2*b1-1,2*b1-1]:=1;
G[2*b1,2*b1]:=1;
G[2*b2-1,2*b2-1]:=1;
G[2*b2,2*b2]:=1;
//solving displacement
Sol:=Gauss_Jordan(N,G,X);
//solving reaction force
SolMat:=matrix(Sol);
XMat:=matrix(X);
R:=Reaction_Trusses(N,Ginitial,SolMat,XMat);
end Trusses_HW;
|
Fungsi Panggil
|
Matrice Transformation function Stiffness_Matrices
input Real A;
Real Y;
output Real X[4,4];
Real float_error = 10e-10;
final constant Real pi=2*Modelica.Math.asin(1.0);
algorithm
Y:=A/180*pi;
X:=[(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y);
Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2;
-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y);
-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2];
for i in 1:4 loop
for j in 1:4 loop
if abs(X[i,j]) <= float_error then
X[i,j] := 0;
end if;
end for;
end for;
end Stiffness_Matrices;
Global Element Matrice function Local_Global
input Real Y[4,4];
input Integer B;
input Integer p1;
input Integer p2;
output Real G[B,B];
algorithm
for i in 1:B loop
for j in 1:B loop
G[i,j]:=0;
end for;
end for;
G[2*p1,2*p1]:=Y[2,2];
G[2*p1-1,2*p1-1]:=Y[1,1];
G[2*p1,2*p1-1]:=Y[2,1];
G[2*p1-1,2*p1]:=Y[1,2];
G[2*p2,2*p2]:=Y[4,4];
G[2*p2-1,2*p2-1]:=Y[3,3];
G[2*p2,2*p2-1]:=Y[4,3];
G[2*p2-1,2*p2]:=Y[3,4];
G[2*p2,2*p1]:=Y[4,2];
G[2*p2-1,2*p1-1]:=Y[3,1];
G[2*p2,2*p1-1]:=Y[4,1];
G[2*p2-1,2*p1]:=Y[3,2];
G[2*p1,2*p2]:=Y[2,4];
G[2*p1-1,2*p2-1]:=Y[1,3];
G[2*p1,2*p2-1]:=Y[2,3];
G[2*p1-1,2*p2]:=Y[1,4];
end Local_Global;
Reaction Matrice Equation function Reaction_Trusses input Integer N; input Real A[N,N]; input Real B[N,1]; input Real C[N,1]; Real X[N,1]; output Real Sol[N]; Real float_error = 10e-10; algorithm X:=A*B-C; for i in 1:N loop if abs(X[i,1]) <= float_error then X[i,1] := 0; end if; end for; for i in 1:N loop Sol[i]:=X[i,1]; end for; end Reaction_Trusses; |