Difference between revisions of "Diagy Alwan Irsyad"

From ccitonlinewiki
Jump to: navigation, search
Line 46: Line 46:
 
==== Finite Element Method for Trusses ====
 
==== Finite Element Method for Trusses ====
  
−
[[File:SoalTrusses4 d29.png|700px|thumb|left]]
+
[[File:SoalTrusses4 d29.png|700px|center]]
 +
 
 
{| class="wikitable"
 
{| class="wikitable"
 
|-
 
|-
 
| style='border-style: none  none  solid  solid;' |  
 
| style='border-style: none  none  solid  solid;' |  
−
''Persamaan''
+
'''''Class'''''
  
 
  class Trusses_Tugas3_d29
 
  class Trusses_Tugas3_d29
Line 156: Line 157:
 
   
 
   
 
  end Trusses_Tugas3_d29;
 
  end Trusses_Tugas3_d29;
 +
|}
 +
 +
{| class="wikitable"
 +
|-
 +
| style='border-style: none  none  solid  solid;' |
 +
'''''Function Stiffness_Matrices'''''
 +
 +
function Stiffness_Matrices
 +
 +
input Real A;
 +
Real Y;
 +
output Real X[4,4];
 +
Real float_error = 10e-10;
 +
 +
final constant Real pi=2*Modelica.Math.asin(1.0);
 +
 +
algorithm
 +
 +
Y:=A/180*pi;
 +
   
 +
X:=[(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y);
 +
 +
Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2;
 +
 +
-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y);
 +
 +
-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2];
 +
 +
for i in 1:4 loop
 +
  for j in 1:4 loop
 +
    if abs(X[i,j]) <= float_error then
 +
      X[i,j] := 0;
 +
    end if;
 +
  end for;
 +
end for;
 +
 +
end Stiffness_Matrices;
 +
|}
 +
 +
{| class="wikitable"
 +
|-
 +
| style='border-style: none  none  solid  solid;' |
 +
'''''Function Gauss-Jordan'''''
 +
function Gauss_Jordan
 +
 +
input Integer N;
 +
input Real A[N,N];
 +
input Real B[N];
 +
output Real X[N];
 +
Real float_error = 10e-10;
 +
 +
algorithm
 +
X:=Modelica.Math.Matrices.solve(A,B);
 +
 +
for i in 1:N loop
 +
  if abs(X[i]) <= float_error then
 +
    X[i] := 0;
 +
  end if;
 +
end for;
 +
 +
end Gauss_Jordan;
 +
|}
 +
 +
{| class="wikitable"
 +
|-
 +
| style='border-style: none  none  solid  solid;' |
 +
'''''Function Reaction_Trusses'''''
 +
function Reaction_Trusses
 +
 +
input Integer N;
 +
input Real A[N,N];
 +
input Real B[N,1];
 +
input Real C[N,1];
 +
Real X[N,1];
 +
output Real Sol[N];
 +
Real float_error = 10e-10;
 +
 +
algorithm
 +
X:=A*B-C;
 +
 +
for i in 1:N loop
 +
  if abs(X[i,1]) <= float_error then
 +
    X[i,1] := 0;
 +
  end if;
 +
end for;
 +
 +
for i in 1:N loop
 +
  Sol[i]:=X[i,1];
 +
end for;
 +
 +
end Reaction_Trusses;
 +
|}
 +
 +
{| class="wikitable"
 +
|-
 +
| style='border-style: none  none  solid  solid;' |
 +
'''''Function Local_Global'''''
 +
function Local_Global
 +
 +
input Real Y[4,4];
 +
input Integer B;
 +
input Integer p1;
 +
input Integer p2;
 +
output Real G[B,B];
 +
 +
algorithm
 +
 +
for i in 1:B loop
 +
  for j in 1:B loop
 +
      G[i,j]:=0;
 +
  end for;
 +
end for;
 +
 +
G[2*p1,2*p1]:=Y[2,2];
 +
G[2*p1-1,2*p1-1]:=Y[1,1];
 +
G[2*p1,2*p1-1]:=Y[2,1];
 +
G[2*p1-1,2*p1]:=Y[1,2];
 +
 +
G[2*p2,2*p2]:=Y[4,4];
 +
G[2*p2-1,2*p2-1]:=Y[3,3];
 +
G[2*p2,2*p2-1]:=Y[4,3];
 +
G[2*p2-1,2*p2]:=Y[3,4];
 +
 +
G[2*p2,2*p1]:=Y[4,2];
 +
G[2*p2-1,2*p1-1]:=Y[3,1];
 +
G[2*p2,2*p1-1]:=Y[4,1];
 +
G[2*p2-1,2*p1]:=Y[3,2];
 +
 +
G[2*p1,2*p2]:=Y[2,4];
 +
G[2*p1-1,2*p2-1]:=Y[1,3];
 +
G[2*p1,2*p2-1]:=Y[2,3];
 +
G[2*p1-1,2*p2]:=Y[1,4];
 +
 +
end Local_Global;
 
|}
 
|}

Revision as of 15:16, 2 December 2020

Biodata

Diagy.JPG

Nama : Diagy Alwan Irsyad

NPM : 1906301154

Angkatan : 2019

Program Studi : Teknik Mesin, S1 Reguler

Tempat, tanggal lahir : Cepu, 29 April 2001

Jenis kelamin : Laki-laki

Alamat surel : diagya.irsyad29@gmail.com / diagy.alwan@ui.ac.id

Metode Numerik-02

Pada semester gasal 2020/2021, saya mengambil mata kuliah Metode Numerik, tepatnya di kelas Metode Numerik-02.

Pertemuan I (11 November 2020)

Pertemuan pertama bersama Pak Dai, saya dan mahasiswa lain diajak berpikir untuk bisa mengetahui hal-hal yang sudah kami ketahui dan yang belum. Dengan mengetahui hal yang belum diketahui, kami bisa mempelajari hal-hal tersebut.

Selain itu, kami juga membahas tentang pengenalan aplikasi OpenModelica. Di akhir kelas, kami diberi tugas untuk membuat video mengenai cara penggunaan OpenModelica.

Berikut adalah video yang saya buat:

Pertemuan II (18 November 2020)

Pertemuan kedua bersama Pak Dai, saya dan mahasiswa lain diminta untuk bisa menjadi orang yang beruntung. Yang dimaksud orang yang beruntung adalah orang yang mampu menjadi pribadi yang lebih baik dibandingkan kemarin. Pak Dai mengingatkan agar kami jangan menjadi orang yang merugi, yaitu orang yang tidak memiliki perkembangan antara hari ini dengan kemarin.

Setelah mendapatkan pelajaran hidup yang berharga, kami diminta untuk mempresentasikan tugas kami yang telah dibuat sebelumnya. Tujuan dari presentasi ini adalah untuk menilai kemampuan mahasiswa dalam pemahaman penggunaan aplikasi OpenModelica.

Materi yang diberikan pada pertemuan ini adalah penggunaan class untuk memanggil sebuah function. Function yang bisa dipanggil beragam jumlahnya, mulai dari penjumlahan sederhana sampai operasi eliminasi matriks.

Di akhir kelas, kami diberi tugas untuk membuat video mengenai cara menyelesaikan persamaan aljabar simultan dengan OpenModelica. Metode yang digunakan menggunakan class untuk memanggil sebuah function, seperti yang sudah diajarkan sebelumnya.

Berikut adalah video yang saya buat:

Pertemuan III (25 November 2020)

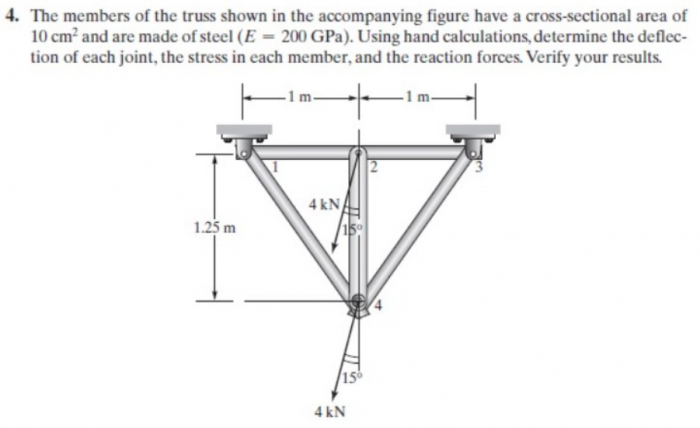
Finite Element Method for Trusses

SoalTrusses4 d29.png

Class 

class Trusses_Tugas3_d29

parameter Integer N=8; //Global matrice = 2*points connected
parameter Real A=0.001; //m^2
parameter Real E=200e9; //Pa
Real G[N,N]; //global
Real Ginitial[N,N]; //global
Real Sol[N]; //global displacement
Real X[N]={0,0,-1035.2762,-3863.7033,0,0,-1035.2762,-3863.7033};
Real R[N]; //global reaction force
Real SolMat[N,1];
Real XMat[N,1];

//boundary condition
Integer b1=1;
Integer b2=3;

//truss 1
parameter Real X1=0; //degree between trusses
Real k1=A*E/1;
Real K1[4,4]; //stiffness matrices
Integer p1a=1;
Integer p1b=2;
Real G1[N,N];

//truss 2
parameter Real X2=0; //degree between trusses
Real k2=A*E/1;
Real K2[4,4]; //stiffness matrices
Integer p2a=2;
Integer p2b=3;
Real G2[N,N];

//truss 3
parameter Real X3=90; //degree between trusses
Real k3=A*E/1.25;
Real K3[4,4]; //stiffness matrices
Integer p3a=2;
Integer p3b=4;
Real G3[N,N];
 
//truss 4
parameter Real X4=90+38.6598; //degree between trusses
Real k4=A*E/1.6;
Real K4[4,4]; //stiffness matrices
Integer p4a=1;
Integer p4b=4;
Real G4[N,N];

//truss 5
parameter Real X5=90-38.6598; //degree between trusses
Real k5=A*E/1.6;
Real K5[4,4]; //stiffness matrices
Integer p5a=3;
Integer p5b=4;
Real G5[N,N];

/*
for each truss, ensure pXa is lower then pXb (X represents truss element number)
*/

algorithm

//creating global matrice
K1:=Stiffness_Matrices(X1);
G1:=k1*Local_Global(K1,N,p1a,p1b);

K2:=Stiffness_Matrices(X2);
G2:=k2*Local_Global(K2,N,p2a,p2b);

K3:=Stiffness_Matrices(X3);
G3:=k3*Local_Global(K3,N,p3a,p3b);

K4:=Stiffness_Matrices(X4);
G4:=k4*Local_Global(K4,N,p4a,p4b);

K5:=Stiffness_Matrices(X5);
G5:=k5*Local_Global(K5,N,p5a,p5b);

G:=G1+G2+G3+G4+G5;
Ginitial:=G;

//implementing boundary condition
for i in 1:N loop
 G[2*b1-1,i]:=0;
 G[2*b1,i]:=0;
 G[2*b2-1,i]:=0;
 G[2*b2,i]:=0;
end for;

G[2*b1-1,2*b1-1]:=1;
G[2*b1,2*b1]:=1;
G[2*b2-1,2*b2-1]:=1;
G[2*b2,2*b2]:=1;

//solving displacement
Sol:=Gauss_Jordan(N,G,X);

//solving reaction force
SolMat:=matrix(Sol);
XMat:=matrix(X);
R:=Reaction_Trusses(N,Ginitial,SolMat,XMat);

end Trusses_Tugas3_d29;

Function Stiffness_Matrices 

function Stiffness_Matrices

input Real A;
Real Y;
output Real X[4,4];
Real float_error = 10e-10;

final constant Real pi=2*Modelica.Math.asin(1.0);

algorithm

Y:=A/180*pi;
    
X:=[(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y);

Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2;

-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y);

-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2];

for i in 1:4 loop
 for j in 1:4 loop
   if abs(X[i,j]) <= float_error then
     X[i,j] := 0;
   end if;
 end for;
end for;

end Stiffness_Matrices;

Function Gauss-Jordan 

function Gauss_Jordan

input Integer N;
input Real A[N,N];
input Real B[N];
output Real X[N];
Real float_error = 10e-10;

algorithm
X:=Modelica.Math.Matrices.solve(A,B);

for i in 1:N loop
  if abs(X[i]) <= float_error then
    X[i] := 0;
  end if;
end for;

end Gauss_Jordan;

Function Reaction_Trusses 

function Reaction_Trusses

input Integer N;
input Real A[N,N];
input Real B[N,1];
input Real C[N,1];
Real X[N,1];
output Real Sol[N];
Real float_error = 10e-10;

algorithm
X:=A*B-C;

for i in 1:N loop
 if abs(X[i,1]) <= float_error then
   X[i,1] := 0;
 end if;
end for;

for i in 1:N loop
 Sol[i]:=X[i,1];
end for;

end Reaction_Trusses;

Function Local_Global 

function Local_Global

input Real Y[4,4];
input Integer B;
input Integer p1;
input Integer p2;
output Real G[B,B];

algorithm

for i in 1:B loop
 for j in 1:B loop
     G[i,j]:=0;
 end for;
end for;

G[2*p1,2*p1]:=Y[2,2];
G[2*p1-1,2*p1-1]:=Y[1,1];
G[2*p1,2*p1-1]:=Y[2,1];
G[2*p1-1,2*p1]:=Y[1,2];

G[2*p2,2*p2]:=Y[4,4];
G[2*p2-1,2*p2-1]:=Y[3,3];
G[2*p2,2*p2-1]:=Y[4,3];
G[2*p2-1,2*p2]:=Y[3,4];

G[2*p2,2*p1]:=Y[4,2];
G[2*p2-1,2*p1-1]:=Y[3,1];
G[2*p2,2*p1-1]:=Y[4,1];
G[2*p2-1,2*p1]:=Y[3,2];

G[2*p1,2*p2]:=Y[2,4];
G[2*p1-1,2*p2-1]:=Y[1,3];
G[2*p1,2*p2-1]:=Y[2,3];
G[2*p1-1,2*p2]:=Y[1,4];

end Local_Global;
Retrieved from "http://air.eng.ui.ac.id/index.php?title=Diagy_Alwan_Irsyad&oldid=45417"