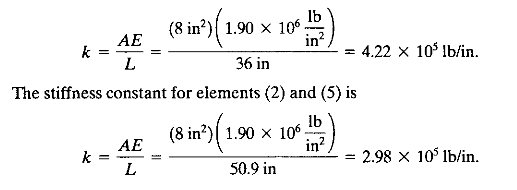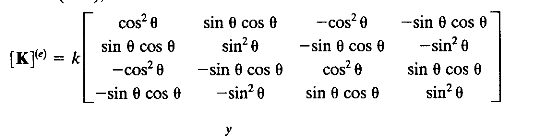Difference between revisions of "Metnum03-Iqbal Reza Alfikri"

From ccitonlinewiki
Jump to: navigation, search
(→‎Pertemuan 6)
(→‎UAS)
 
(8 intermediate revisions by the same user not shown)
Line 264: Line 264:
  
 
optimasi harga material yang paling murah
 
optimasi harga material yang paling murah
 +
 +
== Tugas BEsar ==
 +
 +
Optimasi pemilihan material dan luas penampang truss
 +
 +
[[File:Tugas Besar Metnum Geometri Jos.jpg]]
 +
 +
Asumsi :
 +
 +
1. Variasi Stiffness terikat dengan variabel area. Memvariasikan Elastisitas tergolong sulit karena setiap material memiliki range yang tidak teratur dan dalam satu material yang sejenis (struktur biaya tetap) tidak terjadi perubahan nilai elastisitas yang berbanding lurus dengan perubahan biaya.
 +
 +
2. Beban akan terdistribusi hanya pada point penghubung (karena bersifat truss)
 +
 +
3. Safety factor bernilai 2.
 +
 +
4. Batas displacement 0,001 m sebelum buckling(pada kolom paling atas)
 +
 +
Solusi :
 +
 +
'''Perhitungan stress'''
 +
 +
//define initial variable
 +
parameter Integer Points=16; //Number of Points
 +
parameter Integer Trusses=24; //Number of Trusses
 +
parameter Real Area=0.000224;  //Area L Profile (Dimension=0.03, Thickness=0,004)
 +
parameter Real Elas=193e9;    //Elasticity SS 304
 +
  //define connection
 +
  parameter Integer C[:,2]=[ 1,5;  // (1)
 +
                          2,6;  // (2)
 +
                          3,7;  // (3)
 +
                          4,8;  // (4)
 +
                          5,6;  // (5)
 +
                          6,7;  // (6)
 +
                          7,8;  // (7)
 +
                          5,8;  // (8)
 +
                          5,9;  // (9)
 +
                          6,10; // (10)
 +
                          7,11; // (11)
 +
                          8,12; // (12)
 +
                          9,10; // (13)
 +
                          10,11;// (14)
 +
                          11,12;// (15)
 +
                          9,12; // (16)
 +
                          9,13; // (17)
 +
                          10,14;// (18)
 +
                          11,15;// (19)
 +
                          12,16;// (20)
 +
                          13,14;// (21)
 +
                          14,15;// (22)
 +
                          15,16;// (23)
 +
                          13,16];//(24)
 +
//define coordinates (please put orderly)
 +
parameter Real P[:,6]=[    0  ,0  ,0,1,1,1;    //node 1
 +
                          0.75,0  ,0,1,1,1;    //node 2
 +
                          0.75,0.6,0,1,1,1;    //node 3
 +
                          0  ,0.6,0,1,1,1;      //node 4
 +
                         
 +
                          0  ,0  ,0.3,0,0,0;  //node 5
 +
                          0.75,0  ,0.3,0,0,0;  //node 6
 +
                          0.75,0.6,0.3,0,0,0;  //node 7
 +
                          0  ,0.6,0.3,0,0,0;    //node 8
 +
                         
 +
                          0  ,0  ,1.05,0,0,0;  //node 9
 +
                          0.75,0  ,1.05,0,0,0;  //node 10 
 +
                          0.75,0.6,1.05,0,0,0;  //node 11
 +
                          0  ,0.6,1.05,0,0,0;    //node 12
 +
                         
 +
                          0  ,0  ,1.8,0,0,0;  //node 13
 +
                          0.75,0  ,1.8,0,0,0;  //node 14
 +
                          0.75,0.6,1.8,0,0,0;  //node 15
 +
                          0  ,0.6,1.8,0,0,0];  //node 16
 +
                         
 +
//define external force (please put orderly)
 +
parameter Real F[Points*3]={0,0,0,
 +
                          0,0,0,
 +
                          0,0,0,
 +
                          0,0,0,
 +
                          0,0,0,
 +
                          0,0,0,
 +
                          0,0,0,
 +
                          0,0,0,
 +
                          0,0,0,
 +
                          0,0,0,
 +
                          0,0,0,
 +
                          0,0,0,
 +
                          0,0,-1000,
 +
                          0,0,-500,
 +
                          0,0,-500,
 +
                          0,0,-1000};
 +
//solution
 +
Real displacement[N], reaction[N];
 +
Real check[3];
 +
Real stress1[Trusses];
 +
Real safety[Trusses];
 +
Real dis[3];
 +
Real Str[3];
 +
protected
 +
parameter Integer N=3*Points;
 +
Real q1[3], q2[3], g[N,N], G[N,N], G_star[N,N], id[N,N]=identity(N), cx, cy, cz, L, X[3,3];
 +
Real err=10e-15, ers=10e-8;
 +
algorithm
 +
//Creating Global Matrix
 +
G:=id;
 +
for i in 1:Trusses loop
 +
for j in 1:3 loop
 +
  q1[j]:=P[C[i,1],j];
 +
  q2[j]:=P[C[i,2],j];
 +
end for;     
 +
  //Solving Matrix
 +
  L:=Modelica.Math.Vectors.length(q2-q1);
 +
  cx:=(q2[1]-q1[1])/L;
 +
  cy:=(q2[2]-q1[2])/L;
 +
  cz:=(q2[3]-q1[3])/L;
 +
  X:=(Area*Elas/L)*[cx^2,cx*cy,cx*cz;
 +
                    cy*cx,cy^2,cy*cz;
 +
                    cz*cx,cz*cy,cz^2];
 +
    //Transforming to global matrix
 +
  g:=zeros(N,N);
 +
  for m,n in 1:3 loop
 +
    g[3*(C[i,1]-1)+m,3*(C[i,1]-1)+n]:=X[m,n];
 +
    g[3*(C[i,2]-1)+m,3*(C[i,2]-1)+n]:=X[m,n];
 +
    g[3*(C[i,2]-1)+m,3*(C[i,1]-1)+n]:=-X[m,n];
 +
    g[3*(C[i,1]-1)+m,3*(C[i,2]-1)+n]:=-X[m,n];
 +
  end for; 
 +
G_star:=G+g;
 +
G:=G_star;
 +
end for;
 +
//Implementing boundary
 +
for x in 1:Points loop
 +
if P[x,4] <> 0 then
 +
  for a in 1:Points*3 loop
 +
    G[(x*3)-2,a]:=0;
 +
    G[(x*3)-2,(x*3)-2]:=1;
 +
  end for;
 +
end if;
 +
if P[x,5] <> 0 then
 +
  for a in 1:Points*3 loop
 +
    G[(x*3)-1,a]:=0;
 +
    G[(x*3)-1,(x*3)-1]:=1;
 +
  end for;
 +
end if;
 +
if P[x,6] <> 0 then
 +
  for a in 1:Points*3 loop
 +
    G[x*3,a]:=0;
 +
    G[x*3,x*3]:=1;
 +
  end for;
 +
  end if;
 +
end for;
 +
//Solving displacement
 +
displacement:=Modelica.Math.Matrices.solve(G,F);
 +
//Solving reaction
 +
reaction:=(G_star*displacement)-F;
 +
//Eliminating float error
 +
for i in 1:N loop
 +
  reaction[i]:=if abs(reaction[i])<=err then 0 else reaction[i];
 +
  displacement[i]:=if abs(displacement[i])<=err then 0 else displacement[i];
 +
end for;
 +
//Checking Force
 +
check[1]:=sum({reaction[i] for i in (1:3:(N-2))})+sum({F[i] for i in (1:3:(N-2))});
 +
check[2]:=sum({reaction[i] for i in (2:3:(N-1))})+sum({F[i] for i in (2:3:(N-1))});
 +
check[3]:=sum({reaction[i] for i in (3:3:N)})+sum({F[i] for i in (3:3:N)}); 
 +
for i in 1:3 loop
 +
check[i] := if abs(check[i])<=ers then 0 else check[i];
 +
end for;
 +
//Calculating stress in each truss
 +
for i in 1:Trusses loop
 +
for j in 1:3 loop
 +
  q1[j]:=P[C[i,1],j];
 +
  q2[j]:=P[C[i,2],j];
 +
  dis[j]:=abs(displacement[3*(C[i,1]-1)+j]-displacement[3*(C[i,2]-1)+j]);
 +
end for;     
 +
  //Solving Matrix
 +
  L:=Modelica.Math.Vectors.length(q2-q1);
 +
  cx:=(q2[1]-q1[1])/L;
 +
  cy:=(q2[2]-q1[2])/L;
 +
  cz:=(q2[3]-q1[3])/L;
 +
  X:=(Elas/L)*[cx^2,cx*cy,cx*cz;
 +
                cy*cx,cy^2,cy*cz;
 +
                cz*cx,cz*cy,cz^2];   
 +
  Str:=(X*dis);
 +
  stress1[i]:=Modelica.Math.Vectors.length(Str);
 +
end for;
 +
//Safety factor
 +
for i in 1:Trusses loop
 +
if stress1[i]>0 then
 +
  safety[i]:=Yield/stress1[i];
 +
else
 +
  safety[i]:=0;
 +
end if;
 +
end for;
 +
end Trusses_3D_Tugas_Besar_Safety;
 +
 +
 +
'''untuk bagian curve fitting'''
 +
 +
model callcurve
 +
  parameter Real [8] X={1.11e-4,1.41e-4,1.71e-4,2.31e-4,3.04e-4,3.75e-4,7.44e-4,8.64e-4};
 +
  parameter Real [8] Y={273700 ,318800 ,381200 ,512800 ,683700 ,838000 ,1663100,1986400};
 +
  Real [3] Coe;
 +
algorithm
 +
Coe:=Curve_Fitting(X,Y);
 +
end callcurve;
 +
 +
Function
 +
 +
function Curve_Fitting
 +
  input Real X[:];
 +
  input Real Y[size(X,1)];
 +
  input Integer order=2;
 +
  output Real Coe[order+1];
 +
protected
 +
  Real Z[size(X,1),order+1];
 +
  Real ZTr[order+1,size(X,1)];
 +
  Real A[order+1,order+1];
 +
  Real B[order+1];
 +
algorithm
 +
for i in 1:size(X,1) loop
 +
  for j in 1:(order+1) loop
 +
  Z[i,j]:=X[i]^(order+1-j);
 +
  end for;
 +
end for;
 +
ZTr:=transpose(Z);
 +
A:=ZTr*Z;
 +
B:=ZTr*Y;
 +
Coe:=Modelica.Math.Matrices.solve(A,B); //Coe:=fill(2,size(Coe,1));
 +
 +
 +
 +
'''Program untuk optimasi'''
 +
 +
model Opt_Gold
 +
parameter Real[3] y={-834.974,0.356007,2.39937e-5};
 +
parameter Real xlo=111e-6;
 +
parameter Real xhi=3.75e-4;
 +
parameter Integer N=10; // maximum iteration
 +
parameter Real es=0.0001; // maximum error
 +
Real f1[N], f2[N], x1[N], x2[N], ea[N];
 +
Real xopt,  fx;
 +
protected
 +
Real d, xl, xu, xint, R=(5^(1/2)-1)/2;
 +
algorithm
 +
xl := xlo;
 +
xu := xhi;
 +
for i in 1:N loop
 +
d:= R*(xu-xl);
 +
x1[i]:=xl+d;
 +
x2[i]:=xu-d;
 +
f1[i]:=y[1]*x1[i]^2+y[2]*x1[i]+y[3];
 +
f2[i]:=y[1]*x2[i]^2+y[2]*x2[i]+y[3];
 +
xint:=xu-xl;
 +
if f1[i]>f2[i] then
 +
  xl:=x2[i];
 +
  xopt:=x1[i];
 +
  fx:=f1[i];
 +
  else
 +
    xu:=x1[i];
 +
    xopt:=x2[i];
 +
    fx:=f2[i];
 +
end if;
 +
ea[i]:=(1-R)*abs((xint)/xopt);
 +
if ea[i]<es then
 +
  break;
 +
end if;
 +
end for; end Opt_Gold;
 +
 +
 +
== UAS ==
 +
 +
[[File:WhatsApp_Image_2021-01-13_at_4.26.47_PM_(1).jpeg]]
 +
 +
[[File:P_20210113_165107.jpg|720px]]
 +
 +
[[File:WhatsApp_Image_2021-01-13_at_5.05.42_PM.jpeg]]
 +
 +
[[File:WhatsApp_Image_2021-01-13_at_5.26.38_PM.jpeg]]
 +
 +
[[File:WhatsApp_Image_2021-01-13_at_5.26.47_PM.jpeg]]

Latest revision as of 17:28, 13 January 2021

Nama : Iqbal Reza Alfikri

NPM : 1706104243


Pertemuan 1

Pada pertemuan 1 Pak Dai menjelaskan tentang 4 poin indikator penilaian yaitu

1. Paham konsep

2. Dapat menerapkan konsep untuk memecahkan persamaan

3. Progress

4. Penilaian diri sendiri


Tugas Pertemuan 1

Hal yang sudah dipelajari sebelum UTS yaitu

1. Deret Mclaurin

2. Open method dan bracketing method

3. Regresi Linear

4. Turunan Numerik

Pada tugas 1 juga ditugaskan untuk membuat video tentang openmodelica

Untuk tugas 1 ini saya menggunakan referensi video ini untuk mensimulasikan ketinggian air pada gelas

https://www.youtube.com/watch?v=OsWNgD43Ib8&t=630s

Dan ini adalah Tugas saya

https://youtu.be/du8zcRfDARQ


Pertemuan 2

Pada pertemuan 2, diberikan latihan untuk mencari rata-rata menggunakan looping.

pembelajaran pertemuan 2 tentang class dan function. pada class kita menulis coding untuk di eksekusi kemudian pada fuction adalah tempat dimana memberi perintah dalam bentuk terstruktur seperti proses perhitungan matematik dilakukan di function. function nantinya dapat dipanggil dalam class dengan input yang ditentukan pada class.

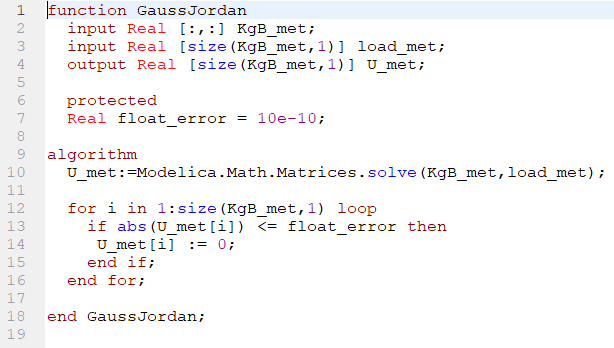
Metode looping juga digunakan untuk melakukan operasi eleminasi Gauss-Jordan

Tugas Pertemuan 2

Melalui Openmodelica rupanya tidak diperlukan metode looping untuk melakukan eleminasi Gauss-Jordan. Karena didalam Openmodelica sendiri sudah tersedia fungsi

Modelica.Math.Matrices.solve

yang merupakan fungsi untuk melakukan eleminasi Gauss-Jordan. Sumber laman : https://build.openmodelica.org/Documentation/Modelica.Math.Matrices.solve.html

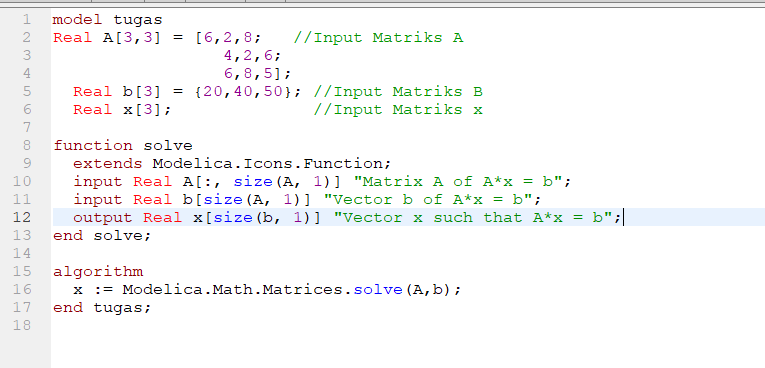
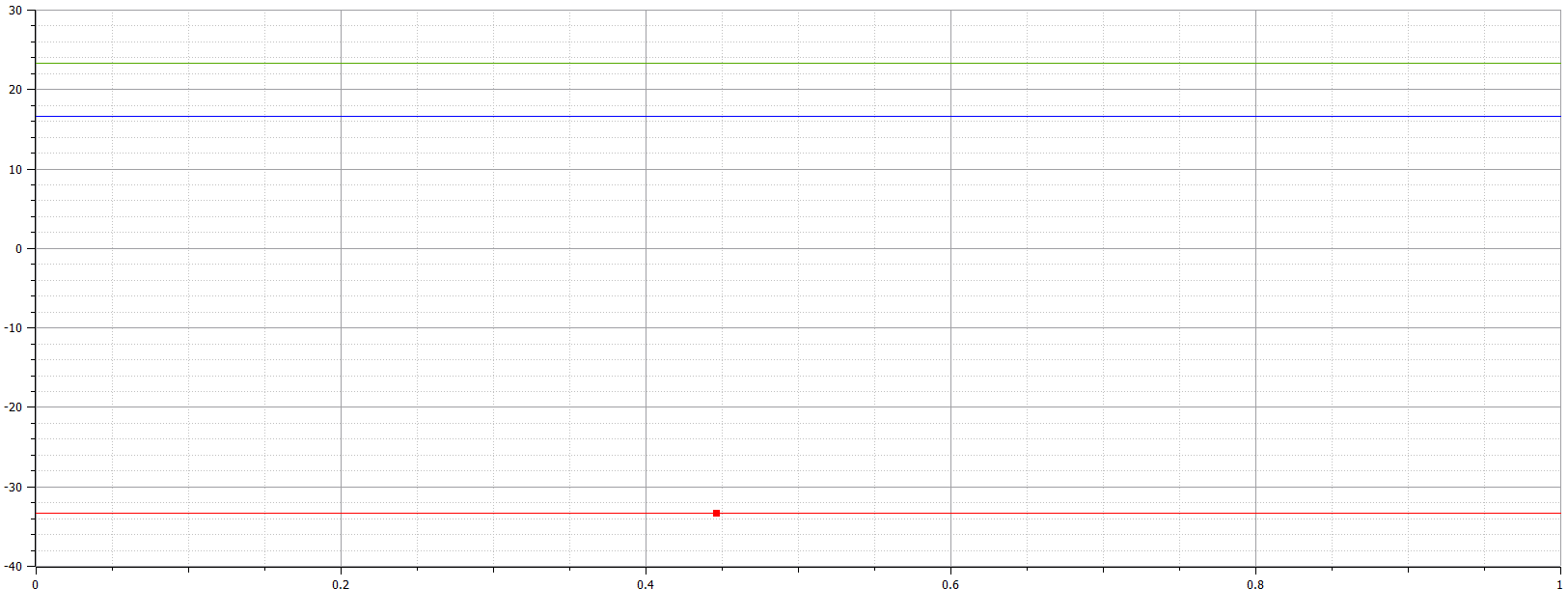
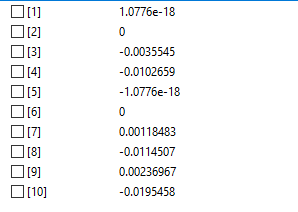
berikut ini adalah contoh penggunaannya

Modelicaiqbal1.PNG

hasilnya didapatkan x sebesar

Hasilmodelica.PNG

Pertemuan 3

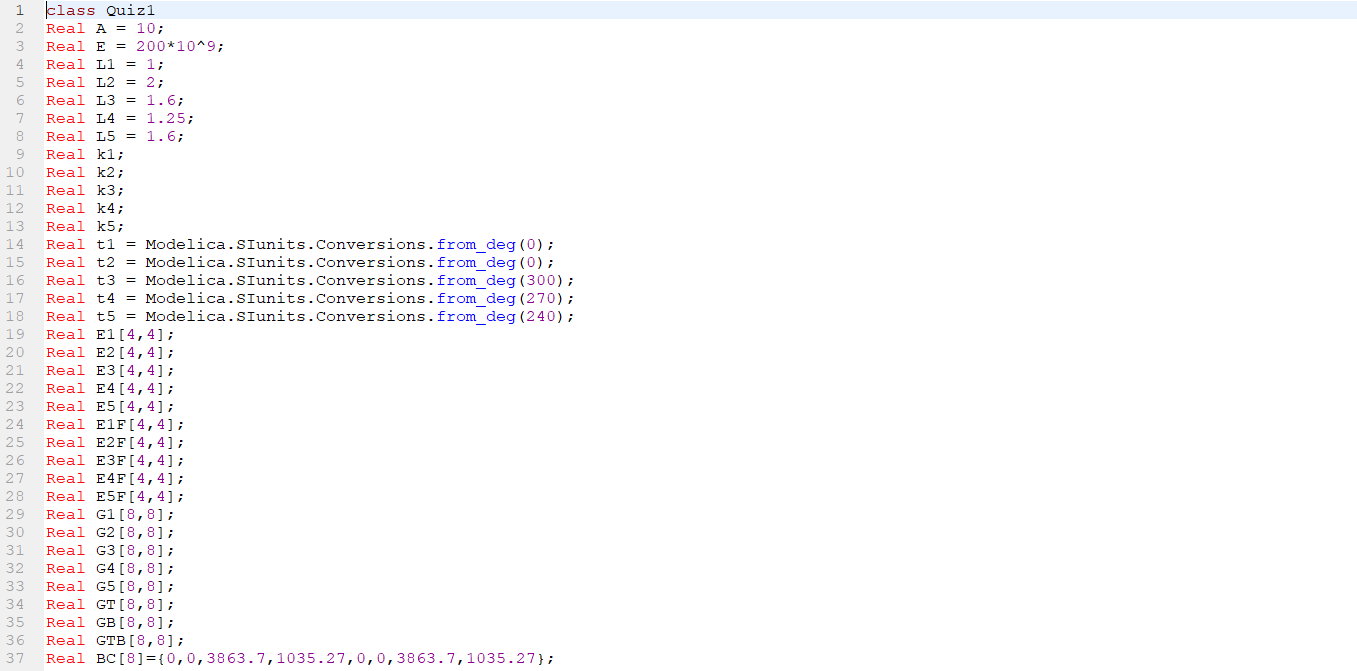
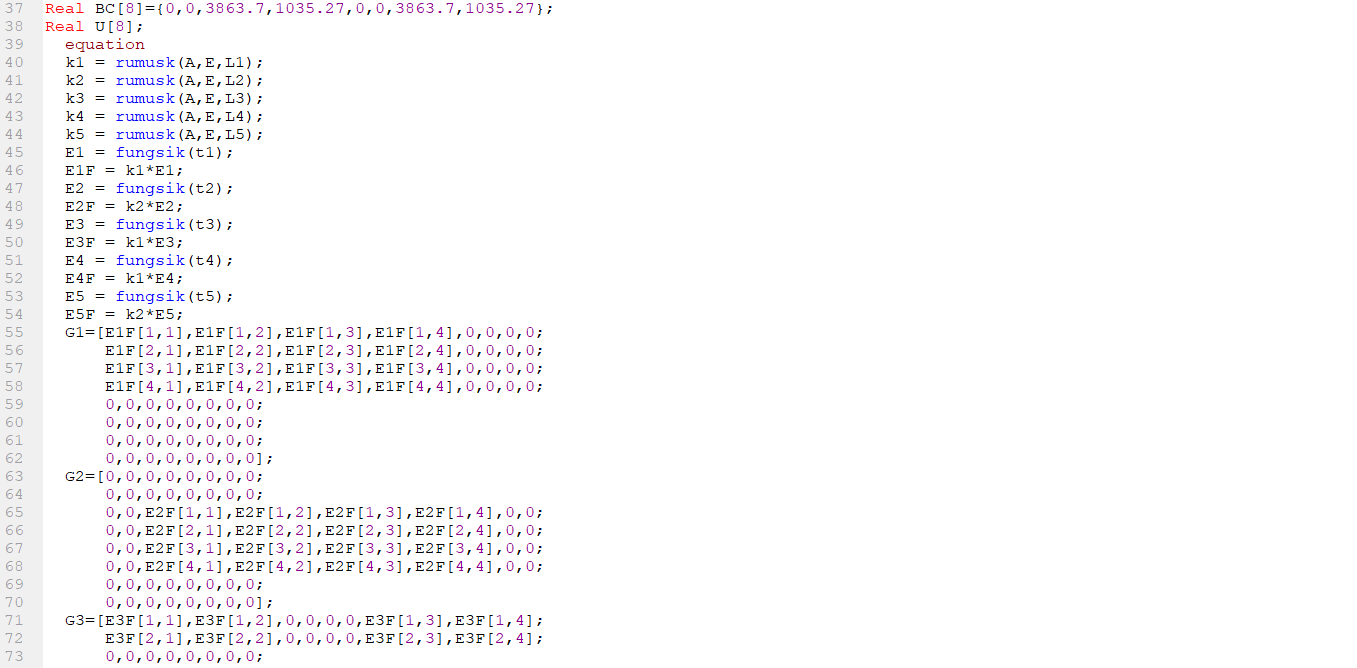
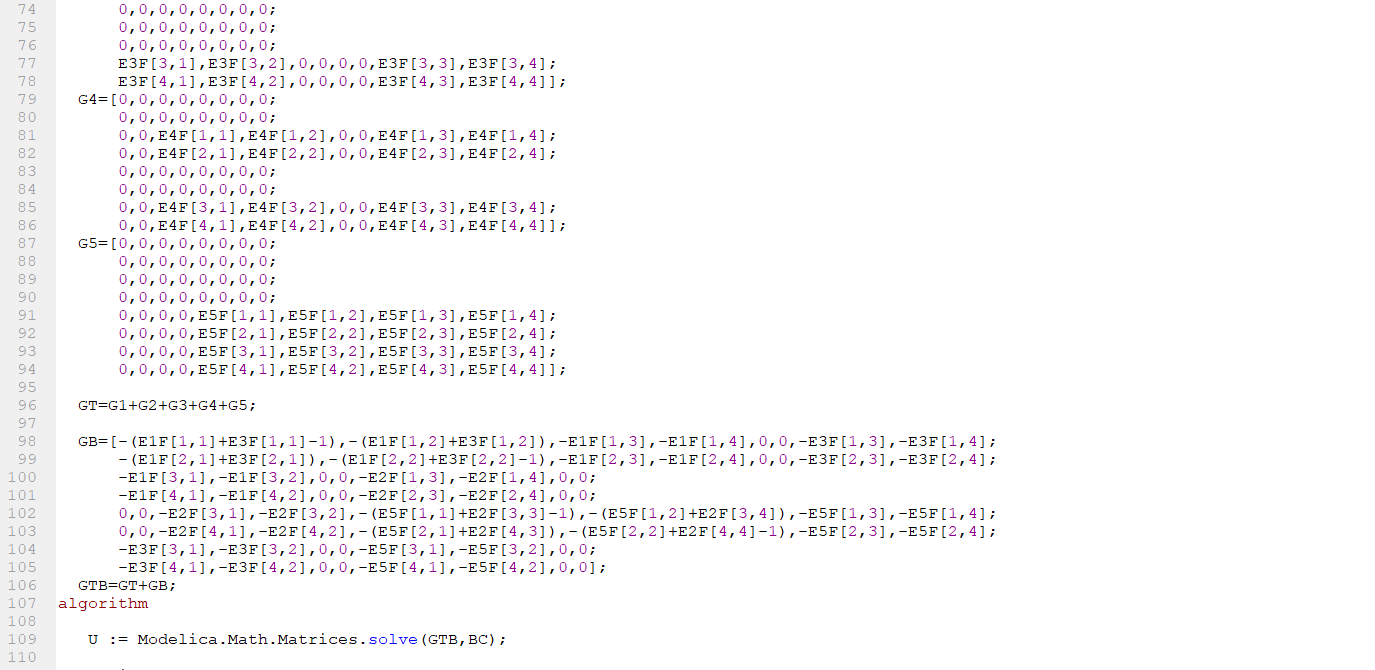
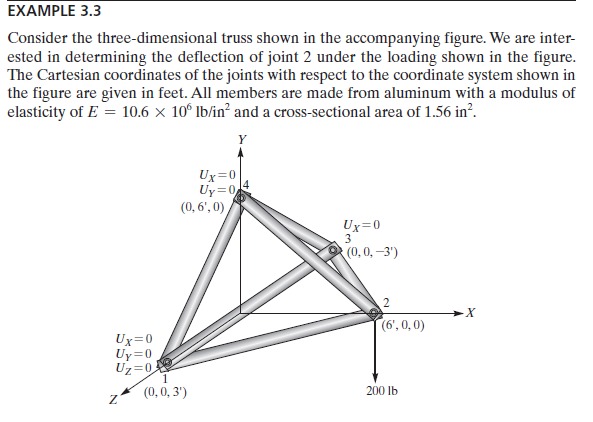
Dalam pertemuan 3 Pak Dai memberikan materi tentang langkah-langkah penyelesaian masalah dalam metode numerik yaitu

Masalah teknik -> Analisis masalah -> Model matematis -> Model numerik -> komputer -> solusi

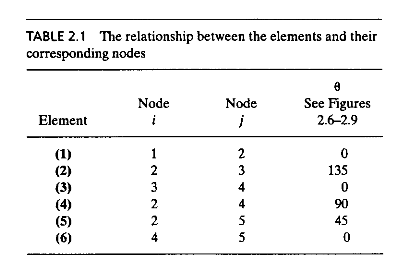
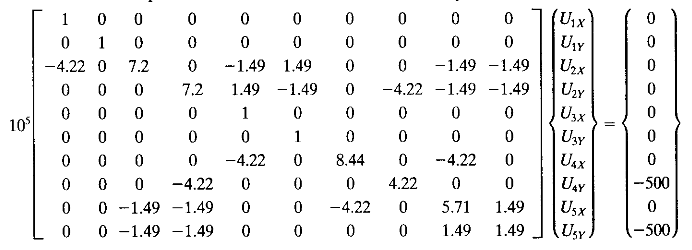
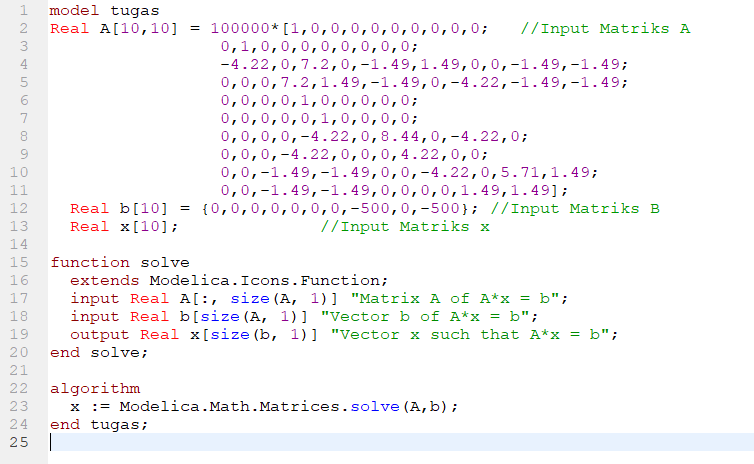
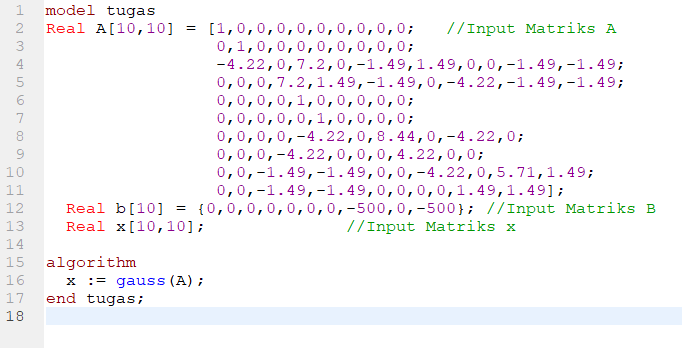
Kemudian untuk tugas Pak Dai memberikan instruksi untuk membuktikan permasalahan yang ada pada buku yaitu persoalan 12.11

Tugas Pertemuan 3

Menyelesaikan soal

Tugas31.PNG

1. Memberikan penamaan agar mudah dalam pengerjaan

Tugas32.PNG

2. Menghitung k untuk tiap elemen

Tugas33.PNG

3. Membuat matriks untuk setiap elemen dengan memasukkan theta kedalam persamaan

Tugas34.PNG

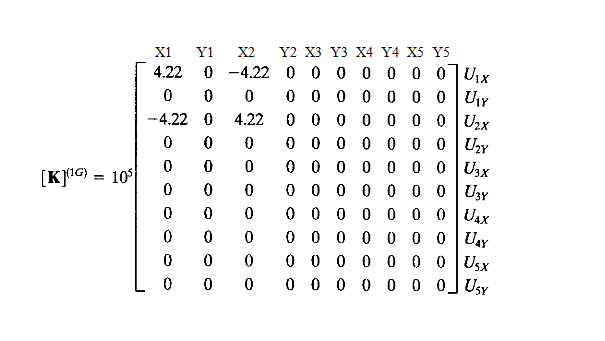
4. Membuat matriks global dari setiap elemen dengan memasukkan matrix dari hasil nomor 3.

Tugas35.PNG

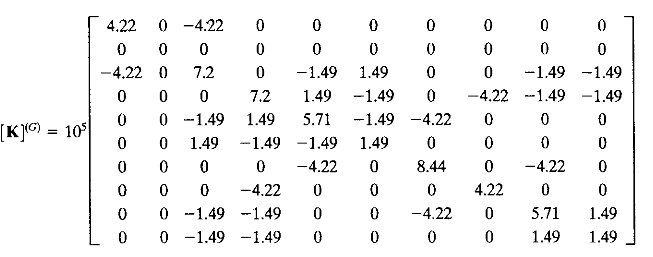
5. Menjumlah semua matrix global dari nomor 4 menjadi matrix global total

Tugas36.PNG

6. Menyelesaikan persamaan

Tugas37.PNG

7. Hasil

Tugas38.PNG

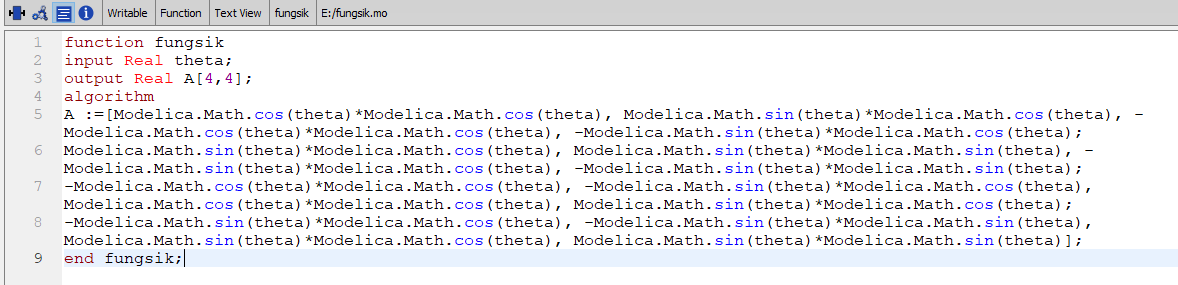
Penyelesaian menggunakan Openmodelica (percobaan pertama)

Untuk percobaan pertama saya mencoba untuk membuat fungsi yang saya beri nama fungsik untuk membuat matriks pada nomor 3 diatas secara otomatis

Tugas39.PNG

lalu kemudian saya panggil fungsi tersebut kedalam class yang sudah saya buat.

Tugas310.PNG

Tugas311.PNG

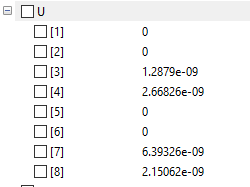
Dalam code ini saya awalnya berharap bahwa saya dapat memasukkan nilai nilai yang berasal dari matriks theta untuk membentuk matriks G[10,10] yang nantinya akan digunakan ke command 

 U := Modelica.Math.Matrices.solve(G,b);

kemudian dari command tersebut akan ditemukan hasil matriks U yaitu displacement pada setiap point.

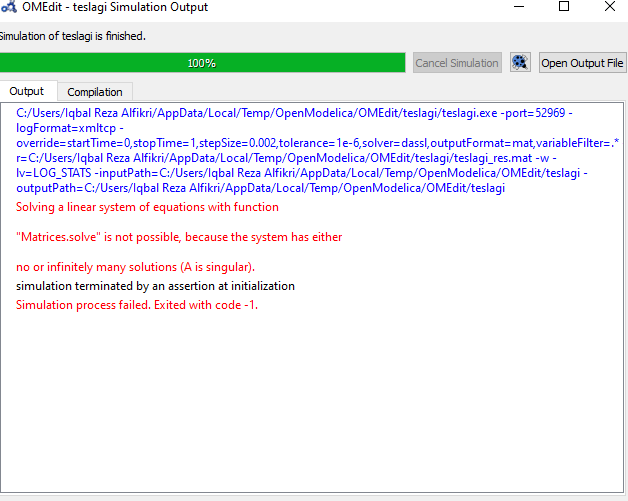
namun setelah saya run terdapat error seperti ini

Tugas312.PNG

Error ini terjadi karena matriks k global total belum disederhanakan dan belum diterapkan boundary condition

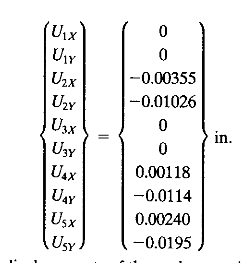
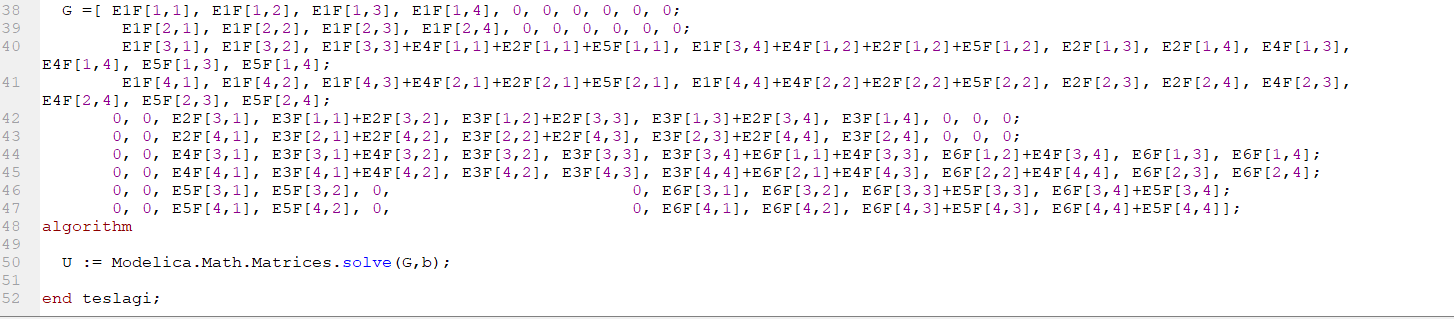
Penyelesaian menggunakan Openmodelica (percobaan kedua)

untuk penyelesaian yang kedua saya hanya memasukkan matrix global yang sudah dijumlahkan kedalam code dibawah ini

Tugas313.PNG

lalu hasilnya adalah seperti ini

Tugas314.PNG

perbedaan percobaan pertama dan kedua adalah di percobaan kedua pembuatan matriks dilakukan secara manual dimana percobaan pertama matriks akan dibuatkan secara otomatis.

berikut ini saya sertakan file OME nya.

file function : https://drive.google.com/file/d/1r6ilGSlWuVNzMAPzV1cYiRzwbBkJP2z_/view?usp=sharing

file class percobaan 1 : https://drive.google.com/file/d/1sGoXsNejOVhWAzn1xJtod0iiRMIv0T4d/view?usp=sharing

file class percobaan 2 : https://drive.google.com/file/d/14xWGhnMeWLZJa-P5XOtDtMD5cPt4dKhY/view?usp=sharing


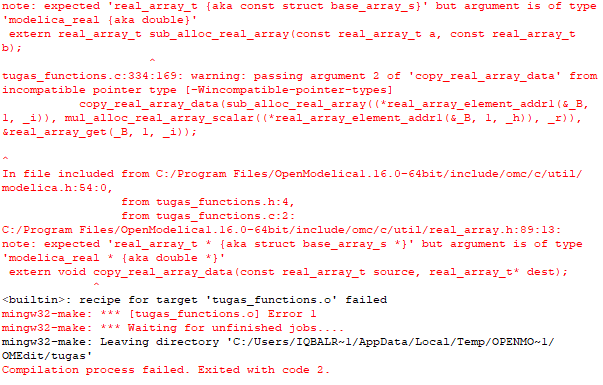
Pertemuan 4

Mencoba fungsi Gauss-Jordan menggunakan loop namun masih error, fungsi tidak bisa dipanggil.

Pertemuan41.PNG

berikut ini adalah code saya

Pertemuan42.PNG


Quiz

berikut ini adalah flowchart code saya yang sedang dalam proses pembuatan

P 20201130 145911 vHDR On.jpg

dan ini adalah codenya yang masih dalam pengerjaan

Pertemuan43.PNG

Pertemuan44.PNG

dan berikut ini adalah fungsi baru yang saya tambahkan yaitu fungsi untuk menghitung k

Pertemuan45.PNG

file function k : https://drive.google.com/file/d/1ejpK3AOfFjUyYHkbSPR-bZ4KSyG24UwV/view?usp=sharing


QUIZ Nomor 4

Quiz11.PNG

Quiz12.PNG

Quiz13.PNG

Quiz14.PNG

Quiz15.PNG

file case nomor 2 : https://drive.google.com/file/d/1IMSU6i-_kH6-0XmpfGw9Ix2KMFK8-29k/view?usp=sharing

QUIZ Nomor 8

pada analisis yang 3d, pada dasarnya langkahnya sama seperti 2d. Namun, persamaan yang digunakan untuk membuat matrix k berbeda dengan 2d. Dan untuk perhitungan panjang pun berbeda dengan 2d. berikut ini adalah fungsi-fungsi yang saya gunakan pada soal nomor 8.

FungsiL.PNG

file fungsi L = https://drive.google.com/file/d/1VDG91gAgRIEFUHuFFzqUfndWj-p1sLtb/view?usp=sharing


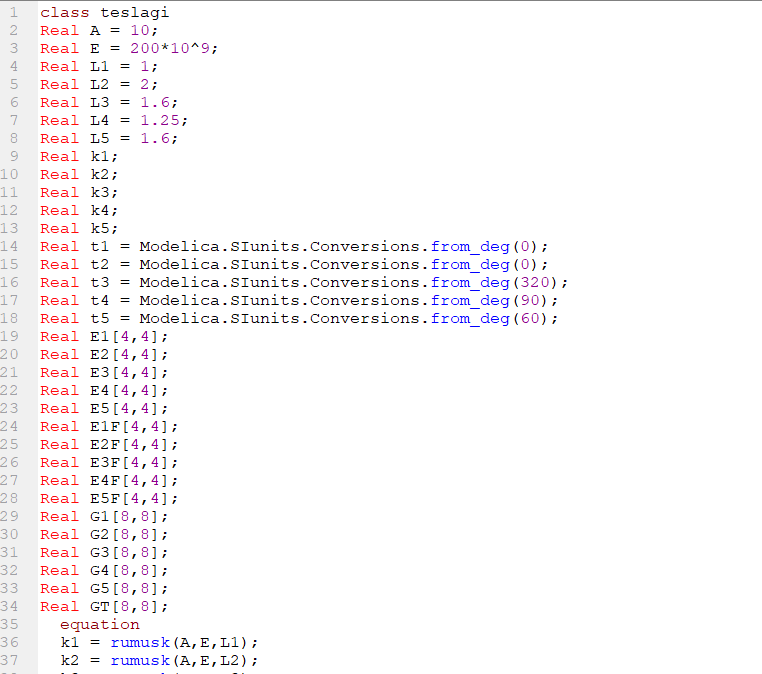
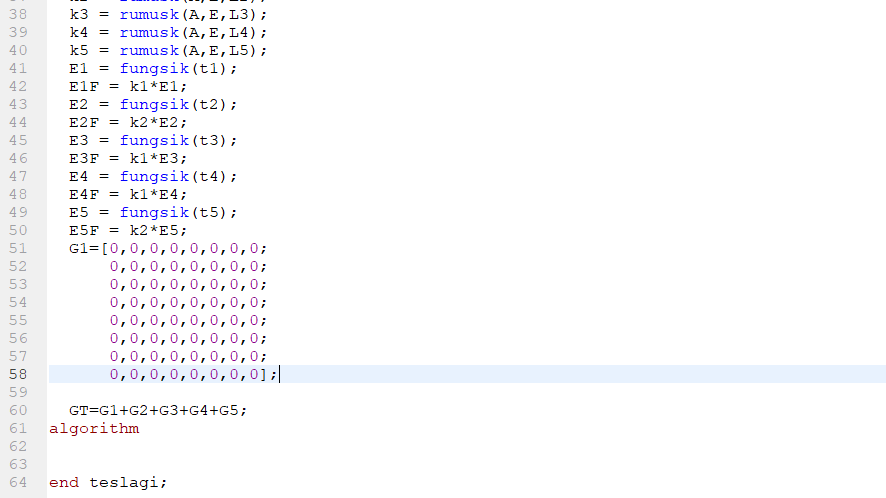
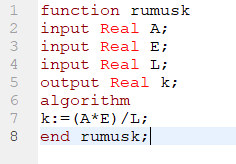
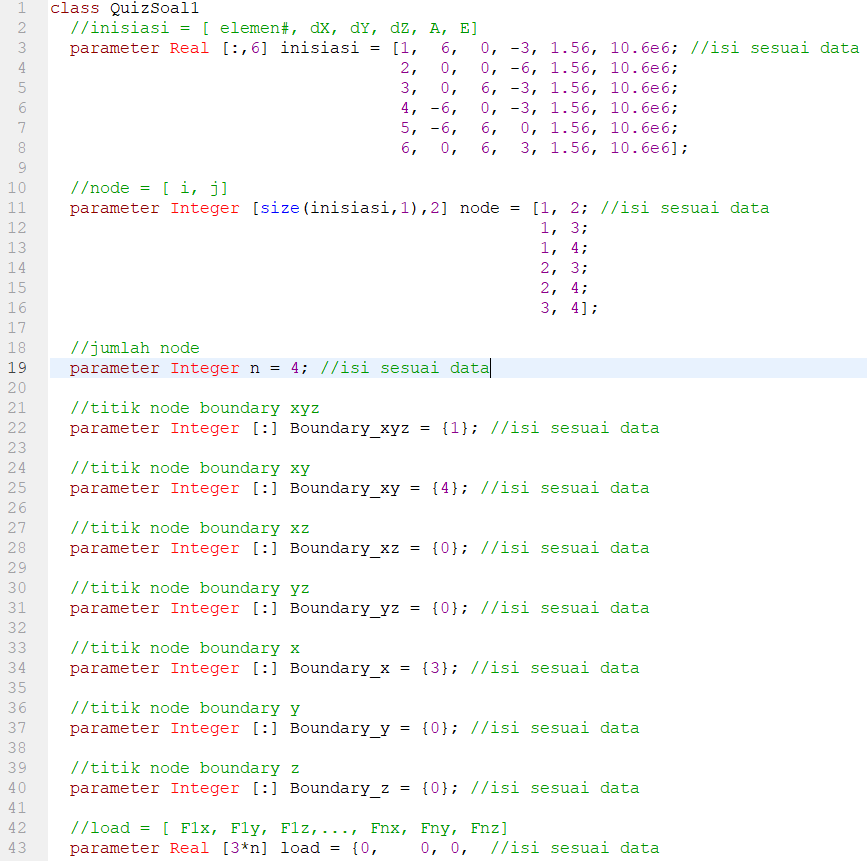
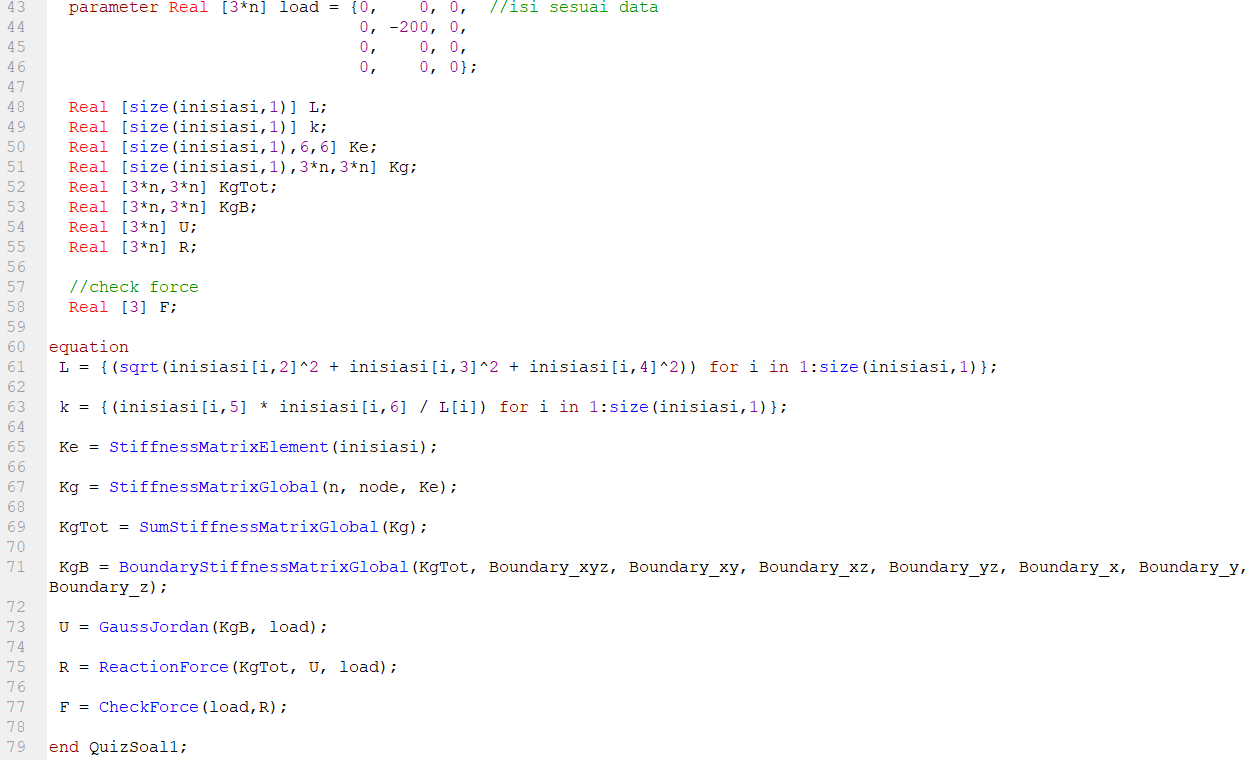
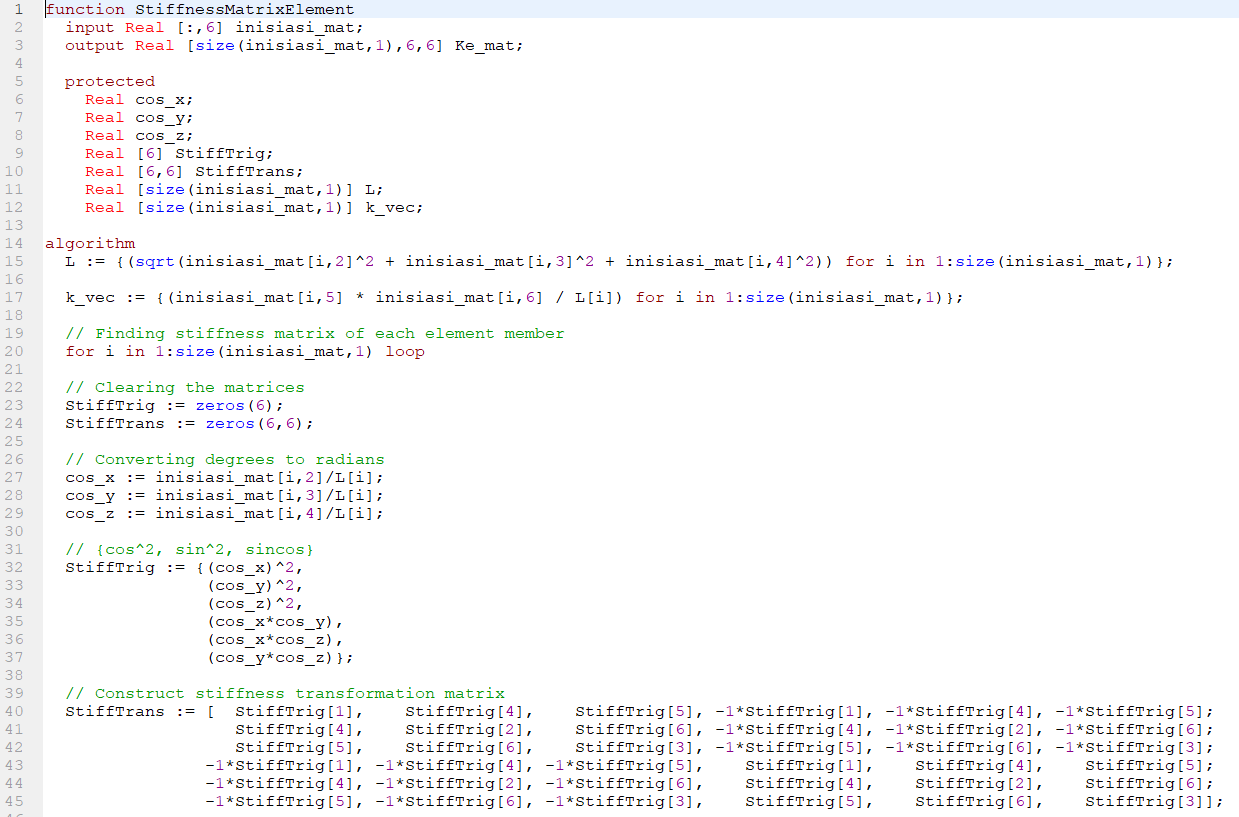
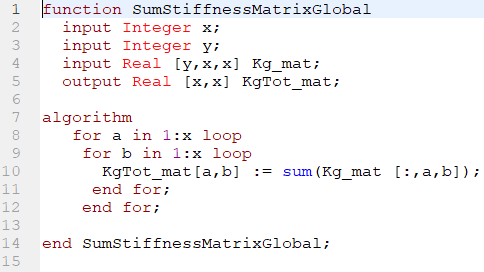
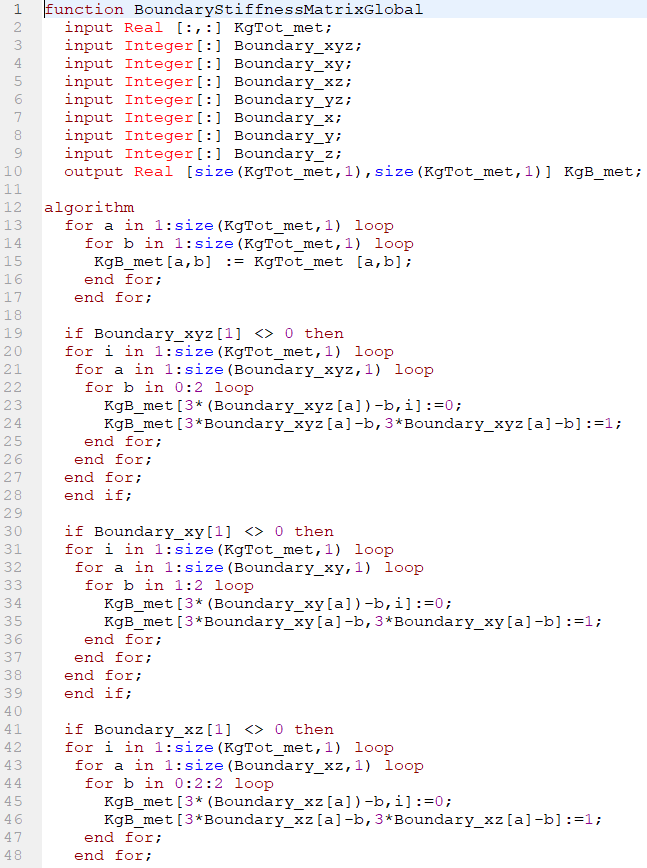
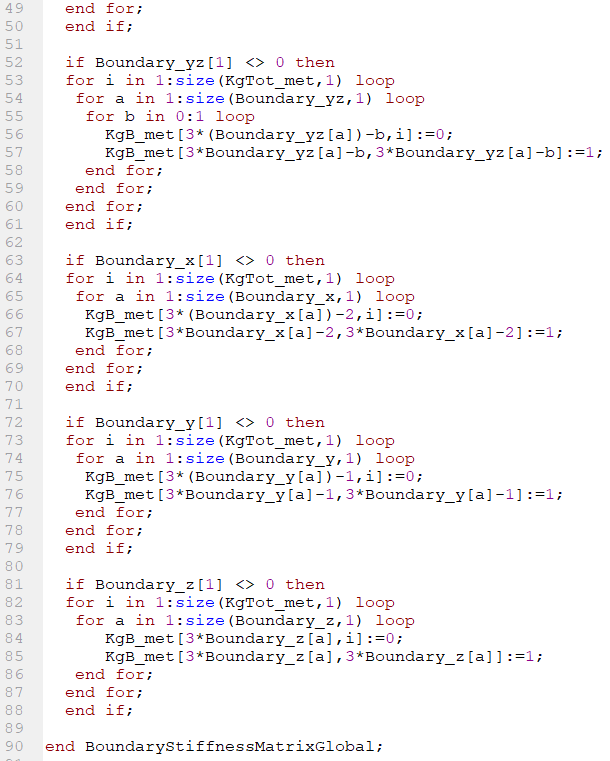
Tugas 5

Soal3.jpeg

Langkah-langkah

1. Menggunakan class untuk menentukan input dan function yang dipanggil

Tugas5a1.PNG

Tugas5a2.PNG

2. Fungsi K untuk setiap elemen

Tugas53.PNG

Tugas54.PNG

3. Fungsi K Global

Tugas55.PNG

4. Fungsi menjumlahkan matriks K global dari setiap elemen

Tugas56.PNG

5. Fungsi Boundary Condition

Tugas57.PNG

Tugas58.PNG

6. Fungsi Gauss Jordan untuk displacement

Tugas59.PNG

7. Fungsi menghitung gaya reaksi

Tugas510.PNG

8. Fungsi mengecek gaya reaksi

Tugas511.PNG

9. Hasil Perhitungan

Tugas512.PNG

Tugas513.PNG


Pertemuan 6

truss setiap nodal hanya punya 3 derajat kebebasan, variabelnya adalah displacement XYZ.

tujuan untuk meminimalkan biaya

constrain harus cukup tangguh

optimasi harga material yang paling murah

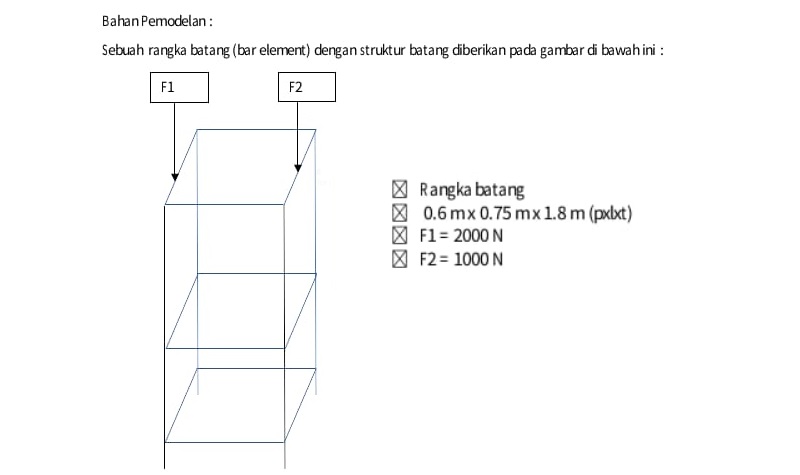
Tugas BEsar

Optimasi pemilihan material dan luas penampang truss

Tugas Besar Metnum Geometri Jos.jpg

Asumsi :

1. Variasi Stiffness terikat dengan variabel area. Memvariasikan Elastisitas tergolong sulit karena setiap material memiliki range yang tidak teratur dan dalam satu material yang sejenis (struktur biaya tetap) tidak terjadi perubahan nilai elastisitas yang berbanding lurus dengan perubahan biaya.

2. Beban akan terdistribusi hanya pada point penghubung (karena bersifat truss)

3. Safety factor bernilai 2.

4. Batas displacement 0,001 m sebelum buckling(pada kolom paling atas)

Solusi :

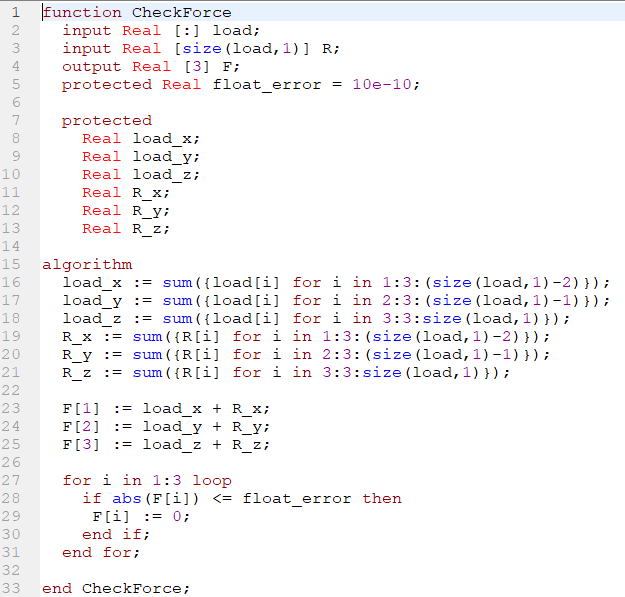
Perhitungan stress 

//define initial variable
parameter Integer Points=16; //Number of Points
parameter Integer Trusses=24; //Number of Trusses
parameter Real Area=0.000224;   //Area L Profile (Dimension=0.03, Thickness=0,004) 
parameter Real Elas=193e9;     //Elasticity SS 304
 //define connection
 parameter Integer C[:,2]=[ 1,5;  // (1)
                         2,6;  // (2)
                         3,7;  // (3)
                         4,8;  // (4)
                         5,6;  // (5)
                         6,7;  // (6)
                         7,8;  // (7)
                         5,8;  // (8)
                         5,9;  // (9)
                         6,10; // (10)
                         7,11; // (11)
                         8,12; // (12)
                         9,10; // (13)
                         10,11;// (14)
                         11,12;// (15)
                         9,12; // (16)
                         9,13; // (17)
                         10,14;// (18)
                         11,15;// (19)
                         12,16;// (20)
                         13,14;// (21)
                         14,15;// (22)
                         15,16;// (23)
                         13,16];//(24)
//define coordinates (please put orderly)
parameter Real P[:,6]=[     0   ,0  ,0,1,1,1;     //node 1
                          0.75,0  ,0,1,1,1;    //node 2
                          0.75,0.6,0,1,1,1;     //node 3
                          0   ,0.6,0,1,1,1;      //node 4
                          
                          0   ,0  ,0.3,0,0,0;   //node 5
                          0.75,0  ,0.3,0,0,0;  //node 6
                          0.75,0.6,0.3,0,0,0;   //node 7
                          0   ,0.6,0.3,0,0,0;    //node 8
                          
                          0   ,0  ,1.05,0,0,0;   //node 9
                          0.75,0  ,1.05,0,0,0;  //node 10  
                          0.75,0.6,1.05,0,0,0;   //node 11
                          0   ,0.6,1.05,0,0,0;    //node 12
                          
                          0   ,0  ,1.8,0,0,0;   //node 13
                          0.75,0  ,1.8,0,0,0;  //node 14
                          0.75,0.6,1.8,0,0,0;   //node 15
                          0   ,0.6,1.8,0,0,0];   //node 16
                          
//define external force (please put orderly)
parameter Real F[Points*3]={0,0,0,
                          0,0,0, 
                          0,0,0, 
                          0,0,0, 
                          0,0,0, 
                          0,0,0, 
                          0,0,0, 
                          0,0,0, 
                          0,0,0, 
                          0,0,0, 
                          0,0,0, 
                          0,0,0, 
                          0,0,-1000, 
                          0,0,-500, 
                          0,0,-500, 
                          0,0,-1000}; 
//solution
Real displacement[N], reaction[N];
Real check[3];
Real stress1[Trusses];
Real safety[Trusses];
Real dis[3];
Real Str[3];
protected
parameter Integer N=3*Points;
Real q1[3], q2[3], g[N,N], G[N,N], G_star[N,N], id[N,N]=identity(N), cx, cy, cz, L, X[3,3];
Real err=10e-15, ers=10e-8;
algorithm
//Creating Global Matrix
G:=id;
for i in 1:Trusses loop
for j in 1:3 loop
 q1[j]:=P[C[i,1],j];
 q2[j]:=P[C[i,2],j];
end for;       
  //Solving Matrix
  L:=Modelica.Math.Vectors.length(q2-q1);
  cx:=(q2[1]-q1[1])/L;
  cy:=(q2[2]-q1[2])/L;
  cz:=(q2[3]-q1[3])/L; 
  X:=(Area*Elas/L)*[cx^2,cx*cy,cx*cz;
                    cy*cx,cy^2,cy*cz;
                    cz*cx,cz*cy,cz^2];
   //Transforming to global matrix
  g:=zeros(N,N); 
  for m,n in 1:3 loop
    g[3*(C[i,1]-1)+m,3*(C[i,1]-1)+n]:=X[m,n];
    g[3*(C[i,2]-1)+m,3*(C[i,2]-1)+n]:=X[m,n];
    g[3*(C[i,2]-1)+m,3*(C[i,1]-1)+n]:=-X[m,n];
    g[3*(C[i,1]-1)+m,3*(C[i,2]-1)+n]:=-X[m,n];
  end for;   
G_star:=G+g;
G:=G_star;
end for;
//Implementing boundary
for x in 1:Points loop
if P[x,4] <> 0 then
  for a in 1:Points*3 loop
    G[(x*3)-2,a]:=0;
    G[(x*3)-2,(x*3)-2]:=1;
  end for;
end if;
if P[x,5] <> 0 then
  for a in 1:Points*3 loop
    G[(x*3)-1,a]:=0;
    G[(x*3)-1,(x*3)-1]:=1;
  end for;
end if;
if P[x,6] <> 0 then
  for a in 1:Points*3 loop
    G[x*3,a]:=0;
    G[x*3,x*3]:=1;
  end for;
 end if;
end for;
//Solving displacement
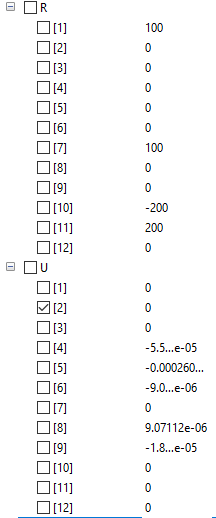
displacement:=Modelica.Math.Matrices.solve(G,F);
//Solving reaction
reaction:=(G_star*displacement)-F;
//Eliminating float error
for i in 1:N loop
 reaction[i]:=if abs(reaction[i])<=err then 0 else reaction[i];
 displacement[i]:=if abs(displacement[i])<=err then 0 else displacement[i];
end for;
//Checking Force
check[1]:=sum({reaction[i] for i in (1:3:(N-2))})+sum({F[i] for i in (1:3:(N-2))});
check[2]:=sum({reaction[i] for i in (2:3:(N-1))})+sum({F[i] for i in (2:3:(N-1))});
check[3]:=sum({reaction[i] for i in (3:3:N)})+sum({F[i] for i in (3:3:N)});  
for i in 1:3 loop
check[i] := if abs(check[i])<=ers then 0 else check[i];
end for;
//Calculating stress in each truss
for i in 1:Trusses loop
for j in 1:3 loop
 q1[j]:=P[C[i,1],j];
 q2[j]:=P[C[i,2],j];
 dis[j]:=abs(displacement[3*(C[i,1]-1)+j]-displacement[3*(C[i,2]-1)+j]);
end for;       
  //Solving Matrix
  L:=Modelica.Math.Vectors.length(q2-q1);
  cx:=(q2[1]-q1[1])/L;
  cy:=(q2[2]-q1[2])/L;
  cz:=(q2[3]-q1[3])/L; 
  X:=(Elas/L)*[cx^2,cx*cy,cx*cz;
               cy*cx,cy^2,cy*cz;
               cz*cx,cz*cy,cz^2];    
  Str:=(X*dis);
  stress1[i]:=Modelica.Math.Vectors.length(Str);
end for;
//Safety factor
for i in 1:Trusses loop
if stress1[i]>0 then
  safety[i]:=Yield/stress1[i];
else
  safety[i]:=0;
end if; 
end for;
end Trusses_3D_Tugas_Besar_Safety;


untuk bagian curve fitting

model callcurve 

 parameter Real [8] X={1.11e-4,1.41e-4,1.71e-4,2.31e-4,3.04e-4,3.75e-4,7.44e-4,8.64e-4};
 parameter Real [8] Y={273700 ,318800 ,381200 ,512800 ,683700 ,838000 ,1663100,1986400};
 Real [3] Coe;
algorithm
Coe:=Curve_Fitting(X,Y);
end callcurve;

Function 

function Curve_Fitting
 input Real X[:];
 input Real Y[size(X,1)];
 input Integer order=2;
 output Real Coe[order+1];
protected
 Real Z[size(X,1),order+1];
 Real ZTr[order+1,size(X,1)];
 Real A[order+1,order+1];
 Real B[order+1];
algorithm
for i in 1:size(X,1) loop
 for j in 1:(order+1) loop
 Z[i,j]:=X[i]^(order+1-j);
 end for;
end for;
ZTr:=transpose(Z);
A:=ZTr*Z;
B:=ZTr*Y;
Coe:=Modelica.Math.Matrices.solve(A,B); //Coe:=fill(2,size(Coe,1));


Program untuk optimasi 

model Opt_Gold
parameter Real[3] y={-834.974,0.356007,2.39937e-5};
parameter Real xlo=111e-6;
parameter Real xhi=3.75e-4; 
parameter Integer N=10; // maximum iteration
parameter Real es=0.0001; // maximum error
Real f1[N], f2[N], x1[N], x2[N], ea[N];
Real xopt,  fx;
protected
Real d, xl, xu, xint, R=(5^(1/2)-1)/2;
algorithm
xl := xlo; 
xu := xhi; 
for i in 1:N loop
d:= R*(xu-xl);
x1[i]:=xl+d;
x2[i]:=xu-d;
f1[i]:=y[1]*x1[i]^2+y[2]*x1[i]+y[3];
f2[i]:=y[1]*x2[i]^2+y[2]*x2[i]+y[3];
xint:=xu-xl; 
if f1[i]>f2[i] then
  xl:=x2[i];
  xopt:=x1[i];
  fx:=f1[i];
  else
    xu:=x1[i];
    xopt:=x2[i];
    fx:=f2[i];
end if; 
ea[i]:=(1-R)*abs((xint)/xopt);
if ea[i]<es then
 break;
end if;
end for; end Opt_Gold;


UAS

WhatsApp Image 2021-01-13 at 4.26.47 PM (1).jpeg

P 20210113 165107.jpg

WhatsApp Image 2021-01-13 at 5.05.42 PM.jpeg

WhatsApp Image 2021-01-13 at 5.26.38 PM.jpeg

WhatsApp Image 2021-01-13 at 5.26.47 PM.jpeg

Retrieved from "http://air.eng.ui.ac.id/index.php?title=Metnum03-Iqbal_Reza_Alfikri&oldid=55082"