Difference between revisions of "Muhammad Naufal Maulana"

From ccitonlinewiki
Jump to: navigation, search
 
(5 intermediate revisions by the same user not shown)
Line 1: Line 1:
−
Assalammualaikum
+
== PERKENALAN SINGKAT ==
 +
Saya Muhammad Naufal Maulana, mahasiswa teknik mesin UI angkatan 2019. Saya tertarik dengan teknik mesin karena memiliki prospek kerja yang luas.
 +
Materi yang sudah saya pelajari sebelum uts ini adalah mengenai turunan numerik, deret mclaurin , interpolasi, regresi, pengertian dari metode numerik, pseucode.
  
−
Perkenalkan nama saya
+
== MINGGU KE 1 ==
−
Muhammad Naufal Maulana
+
Tujuan mempelajari metode numerik
 +
* Memahami konsep-konsep dan prinsip-prinsip dasar dalam metode numerik seperti:Persamaan algoritma, aljabar, pencocokan kurva, persamaan diferensial parsial, dan lainnya
 +
* Mengerti dan mampu menerapkan pemahaman atau aplikasi terhadap konsep metode numerik
 +
* Mampu menerapkan metode numerik dalam hal terkait persoalan keteknikan
 +
* Mendapat added value (nilai tambah)/adab sehingga mahasiswa menjadi orang yang memiliki budi pekerti yang baik
 +
 
 +
==TUGAS 1 ==
 +
Pada pertemuan sebelumnya, saya diberi tugas membuat video terkait penggunaan aplikasi open modelica. Berikut ini adalah hasil tugas saya:
 +
<div class="center" style="width: auto; margin-left: auto; margin-right: auto;">
 +
<youtube width="200" height="100">https://youtu.be/M3GV76YnS1w</youtube>
 +
</div>
 +
 
 +
== MINGGU KE 2 ==
 +
Pertemuan kedua bersama Pak Dai, Kami diminta menjadi pribadi yang lebih baik dari hari kemarin. Setelah itu, kami diminta untuk mempresentasikan tugas kami sebelumnya. Setelah itu materi yang diberikan pada pertemuan ini adalah penggunaan ''class'' untuk memanggil sebuah ''function''.  ''Function'' yang bisa dipanggil beragam jumlahnya, mulai dari penjumlahan sederhana sampai operasi eliminasi matriks.
 +
 
 +
== TUGAS 2 ==
 +
Di akhir kelas, kami diberi tugas untuk membuat video mengenai cara menyelesaikan persamaan aljabar simultan dengan OpenModelica, dengan metode yang telah diajarkan sebelumnya. Berikut ini adalah hasil tugas saya:
 +
<div class="center" style="width: auto; margin-left: auto; margin-right: auto;">
 +
<youtube width="200" height="100">https://youtu.be/-xLi_IO2oGY</youtube>
 +
</div>
 +
 
 +
== MINGGU KE 3 ==
 +
Pada pertemuan ketiga dilakukan pembahasan tentang penggunaan OpenModelica pada permasalahan engineering. Pada permasalahan engineering, metode numerik dapat membantu menyelesaikan persoalan lebih cepat. Contoh metode numerik  yang dapat membantu menyelesaikan permasalaha engineering diantaranya  Metode Stokastik, CFD(Computation fluid dynamics, dan FEA ( FInite Element Analaysis).
 +
Tahapan untuk menyelesaikan masalah engineering menggunakan metode numerik:
 +
*1. Melakukan analisis terhadap masalah engineering
 +
*2. Melakukan pemodelan matematis dari masalah yang sudah dianalisis
 +
*3. Menerjemahkan pemodelan matematis yang sudah dibuat menjadi metode numerik agar bisa dhitung oleh komputer
 +
*4. Melakukan perhitungan metode numerik menggunakan software dan dihasilkanlah solusi dari masalah engineering tersebut
 +
 
 +
== TUGAS 3 ==
 +
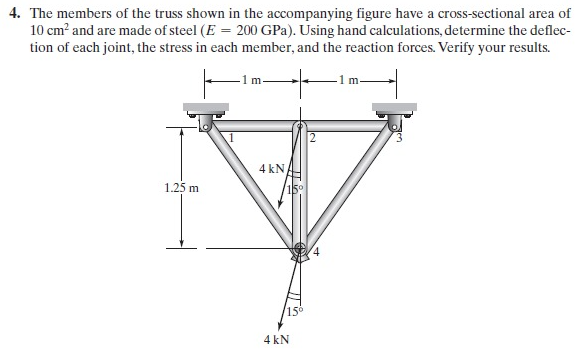
Mencari Truss Analysis menggunakan OpenModelica
 +
[[File:Chapter 3 Problem 4 (Finite Element Analysis, 4th Edition, Saeed Moaveni).png|600px|thumb|center]]
 +
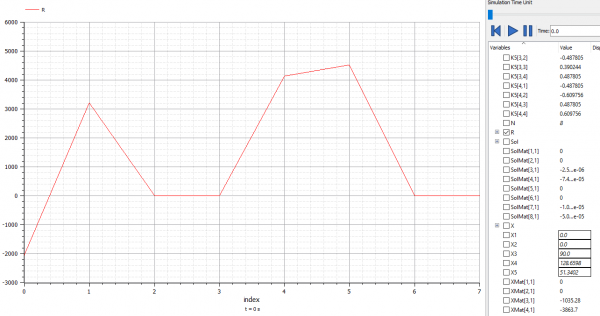
[[File:Grafik Reaction Proccess.png|600px|thumb|right|Grafik Reaction Forces]]
 +
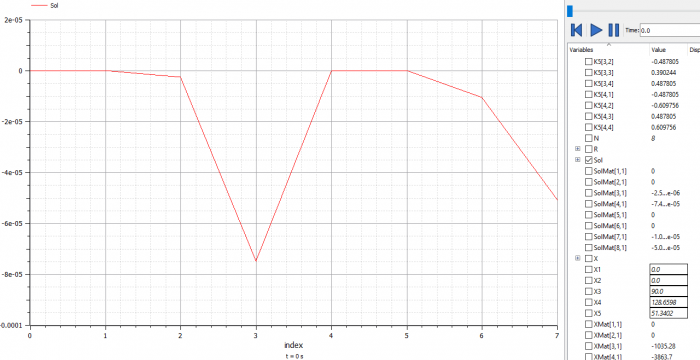
[[File:Grafik Displacement.png|700px|thumb|right|Grafik Displacement]]
 +
{| class="wikitable"
 +
|-
 +
| style='border-style: none  none  solid  solid;' |
 +
''Persamaan''
 +
 
 +
class Trusses_HW
 +
 +
parameter Integer N=8; //Global matrice = 2*points connected
 +
parameter Real A=0.001; //Area m2
 +
parameter Real E=200e9; //Pa
 +
Real G[N,N]; //global
 +
Real Ginitial[N,N]; //global
 +
Real Sol[N]; //global dispplacement
 +
Real X[N]={0,0,-1035.2762,-3863.7033,0,0,-1035.2762,-3863.7033};
 +
Real R[N]; //global reaction force
 +
Real SolMat[N,1];
 +
Real XMat[N,1];
 +
 +
//boundary condition
 +
Integer b1=1;
 +
Integer b2=3;
 +
 +
//truss 1
 +
parameter Real X1=0; //degree between truss
 +
Real k1=A*E/1;
 +
Real K1[4,4]; //stiffness matrice
 +
Integer p1a=1;
 +
Integer p1b=2;
 +
Real G1[N,N];
 +
 +
//truss 2
 +
parameter Real X2=0; //degree between truss
 +
Real k2=A*E/1;
 +
Real K2[4,4]; //stiffness matrice
 +
Integer p2a=2;
 +
Integer p2b=3;
 +
Real G2[N,N];
 +
 +
//truss 3
 +
parameter Real X3=90; //degree between truss
 +
Real k3=A*E/1.25;
 +
Real K3[4,4]; //stiffness matrice
 +
Integer p3a=2;
 +
Integer p3b=4;
 +
Real G3[N,N];
 +
 +
//truss 4
 +
parameter Real X4=90+38.6598; //degree between truss
 +
Real k4=A*E/1.6;
 +
Real K4[4,4]; //stiffness matrice
 +
Integer p4a=1;
 +
Integer p4b=4;
 +
Real G4[N,N];
 +
 +
//truss 5
 +
parameter Real X5=90-38.6598; //degree between truss
 +
Real k5=A*E/1.6;
 +
Real K5[4,4]; //stiffness matrice
 +
Integer p5a=3;
 +
Integer p5b=4;
 +
Real G5[N,N];
 +
 +
/*
 +
for each truss, please ensure pXa is lower then pXb (X represents truss element number)
 +
*/
 +
 +
algorithm
 +
 +
//creating global matrice
 +
K1:=Stiffness_Matrices(X1);
 +
G1:=k1*Local_Global(K1,N,p1a,p1b);
 +
 +
K2:=Stiffness_Matrices(X2);
 +
G2:=k2*Local_Global(K2,N,p2a,p2b);
 +
 +
K3:=Stiffness_Matrices(X3);
 +
G3:=k3*Local_Global(K3,N,p3a,p3b);
 +
 +
K4:=Stiffness_Matrices(X4);
 +
G4:=k4*Local_Global(K4,N,p4a,p4b);
 +
 +
K5:=Stiffness_Matrices(X5);
 +
G5:=k5*Local_Global(K5,N,p5a,p5b);
 +
 +
G:=G1+G2+G3+G4+G5;
 +
Ginitial:=G;
 +
 +
//implementing boundary condition
 +
for i in 1:N loop
 +
  G[2*b1-1,i]:=0;
 +
  G[2*b1,i]:=0;
 +
  G[2*b2-1,i]:=0;
 +
  G[2*b2,i]:=0;
 +
end for;
 +
 +
G[2*b1-1,2*b1-1]:=1;
 +
G[2*b1,2*b1]:=1;
 +
G[2*b2-1,2*b2-1]:=1;
 +
G[2*b2,2*b2]:=1;
 +
 +
//solving displacement
 +
Sol:=Gauss_Jordan(N,G,X);
 +
 +
//solving reaction force
 +
SolMat:=matrix(Sol);
 +
XMat:=matrix(X);
 +
R:=Reaction_Trusses(N,Ginitial,SolMat,XMat);
 +
 +
end Trusses_HW;
 +
|}
 +
 
 +
'''Fungsi Panggil'''
 +
{| class="wikitable"
 +
|-
 +
| style='border-style: none  none  solid  solid;' |
 +
''Matrice Transformation''
 +
 
 +
 
 +
function Stiffness_Matrices
 +
input Real A;
 +
Real Y;
 +
output Real X[4,4];
 +
Real float_error = 10e-10;
 +
 +
final constant Real pi=2*Modelica.Math.asin(1.0);
 +
 +
algorithm
 +
 +
Y:=A/180*pi;
 +
   
 +
X:=[(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y);
 +
 +
Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2;
 +
 +
-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y);
 +
 +
-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2];
 +
 +
for i in 1:4 loop
 +
  for j in 1:4 loop
 +
    if abs(X[i,j]) <= float_error then
 +
      X[i,j] := 0;
 +
    end if;
 +
  end for;
 +
end for;
 +
 +
end Stiffness_Matrices;
 +
|}
 +
 
 +
{| class="wikitable"
 +
|-
 +
| style='border-style: none  none  solid  solid;' |
 +
''Global Element Matrice''
 +
 
 +
function Local_Global
 +
input Real Y[4,4];
 +
input Integer B;
 +
input Integer p1;
 +
input Integer p2;
 +
output Real G[B,B];
 +
 +
algorithm
 +
 +
for i in 1:B loop
 +
  for j in 1:B loop
 +
      G[i,j]:=0;
 +
  end for;
 +
end for;
 +
 +
G[2*p1,2*p1]:=Y[2,2];
 +
G[2*p1-1,2*p1-1]:=Y[1,1];
 +
G[2*p1,2*p1-1]:=Y[2,1];
 +
G[2*p1-1,2*p1]:=Y[1,2];
 +
 +
G[2*p2,2*p2]:=Y[4,4];
 +
G[2*p2-1,2*p2-1]:=Y[3,3];
 +
G[2*p2,2*p2-1]:=Y[4,3];
 +
G[2*p2-1,2*p2]:=Y[3,4];
 +
 +
G[2*p2,2*p1]:=Y[4,2];
 +
G[2*p2-1,2*p1-1]:=Y[3,1];
 +
G[2*p2,2*p1-1]:=Y[4,1];
 +
G[2*p2-1,2*p1]:=Y[3,2];
 +
 +
G[2*p1,2*p2]:=Y[2,4];
 +
G[2*p1-1,2*p2-1]:=Y[1,3];
 +
G[2*p1,2*p2-1]:=Y[2,3];
 +
G[2*p1-1,2*p2]:=Y[1,4];
 +
 +
end Local_Global;
 +
|}
 +
 
 +
 
 +
{| class="wikitable"
 +
|-
 +
| style='border-style: none  none  solid  solid;' |
 +
''Reaction Matrices Equation''
 +
 
 +
function Reaction_Trusses
 +
input Integer N;
 +
input Real A[N,N];
 +
input Real B[N,1];
 +
input Real C[N,1];
 +
Real X[N,1];
 +
output Real Sol[N];
 +
Real float_error = 10e-10;
 +
 +
algorithm
 +
X:=A*B-C;
 +
 +
for i in 1:N loop
 +
  if abs(X[i,1]) <= float_error then
 +
    X[i,1] := 0;
 +
  end if;
 +
end for;
 +
 +
for i in 1:N loop
 +
  Sol[i]:=X[i,1];
 +
end for;
 +
 +
end Reaction_Trusses;
 +
|}
 +
 
 +
{| class="wikitable"
 +
|-
 +
| style='border-style: none  none  solid  solid;' |
 +
''Gauss Jordan''
 +
function Gauss_Jordan
 +
input Integer N;
 +
input Real A[N,N];
 +
input Real B[N];
 +
output Real X[N];
 +
Real float_error = 10e-10;
 +
algorithm
 +
X:=Modelica.Math.Matrices.solve(A,B);
 +
for i in 1:N loop
 +
  if abs(X[i]) <= float_error then
 +
    X[i] := 0;
 +
  end if;
 +
end for;
 +
end Gauss_Jordan;
 +
|}
 +
 
 +
== TUGAS BESAR ==
 +
Tugas besar yang diberikan untuk kelas metnum 02 dan 03 adalah melakukan optimasi pada rangka sederhana:
 +
[[File:Soal Tugas Besar.jpg|500px|center]]
 +
Dengan data sebagai berikut:
 +
[[File:Data Tugas Besar.jpg|500px|center]]
 +
Pada soal ini kami diharapkan dapat mendesain rangka batang dengan harga terjangkau dengan fungsi yang optimal.
 +
 
 +
Faktor atau variabel bebasnya meliputi : Harga, Material yang digunakan, cross section Dilakukan dengan metode optimasi dan membentuk curve fitting pada variabel harga
 +
 
 +
'''Constrain'''
 +
 
 +
- Spesifikasi L (Panjang) dan geometri rangka truss
 +
 
 +
- Gaya beban diberikan kepada struktur (1000 N dan 2000 N)
 +
 
 +
 
 +
'''Asumsi'''
 +
 
 +
- Variasi Stiffness terikat dengan variabel area. Memvariasikan Elastisitas tergolong sulit karena setiap material memiliki range yang tidak teratur dan dalam satu material yang sejenis (struktur biaya tetap) tidak terjadi perubahan nilai elastisitas yang berbanding lurus dengan perubahan biaya.
 +
 
 +
- Beban akan terdistribusi hanya pada point penghubung
 +
Untuk perhitungan displacement, reaction force, dan stress
 +
model Trusses_3D_Tugas_Besar_Safety
 +
 +
//define initial variable
 +
parameter Integer Points=size(P,1); //Number of Points
 +
parameter Integer Trusses=size(C,1); //Number of Trusses
 +
parameter Real Yield=275e6; //Yield Strength (Pa)
 +
parameter Real Area=0.000224;  //Area L Profile (Dimension=0.03, Thickness=0,004) (m2)
 +
parameter Real Elas=193e9;    //Elasticity SS 302  (Pa)
 +
 +
//define connection
 +
parameter Integer C[:,2]=[1,5;
 +
                          2,6;
 +
                          3,7;
 +
                          4,8;
 +
                          5,6;  //1st floor
 +
                          6,7;  //1st floor
 +
                          7,8;  //1st floor
 +
                          5,8;  //1st floor
 +
                          5,9;
 +
                          6,10;
 +
                          7,11;
 +
                          8,12;
 +
                          9,10; //2nd floor
 +
                          10,11;//2nd floor
 +
                          11,12;//2nd floor
 +
                          9,12; //2nd floor
 +
                          9,13;
 +
                          10,14;
 +
                          11,15;
 +
                          12,16;
 +
                          13,14;//3rd floor
 +
                          14,15;//3rd floor
 +
                          15,16;//3rd floor
 +
                        13,16];//3rd floor
 +
                                                             
 +
//define coordinates (please put orderly)
 +
parameter Real P[:,6]=[0.3,-0.375,0,1,1,1;    //1
 +
                        -0.3,-0.375,0,1,1,1;    //2
 +
                        -0.3,0.375,0,1,1,1;    //3
 +
                        0.3,0.375,0,1,1,1;      //4
 +
                           
 +
                        0.3,-0.375,0.6,0,0,0;  //5
 +
                        -0.3,-0.375,0.6,0,0,0;  //6
 +
                        -0.3,0.375,0.6,0,0,0;  //7
 +
                        0.3,0.375,0.6,0,0,0;    //8
 +
                           
 +
                        0.3,-0.375,1.2,0,0,0;  //9
 +
                        -0.3,-0.375,1.2,0,0,0;  //10 
 +
                        -0.3,0.375,1.2,0,0,0;  //11
 +
                        0.3,0.375,1.2,0,0,0;    //12
 +
                           
 +
                        0.3,-0.375,1.8,0,0,0;  //13
 +
                        -0.3,-0.375,1.8,0,0,0;  //14
 +
                        -0.3,0.375,1.8,0,0,0;  //15
 +
                        0.3,0.375,1.8,0,0,0];  //16
 +
                         
 +
//define external force (please put orderly)
 +
parameter Real F[Points*3]={0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,-500,
 +
                            0,0,-1000,
 +
                            0,0,-1000,
 +
                            0,0,-500};
 +
 +
//solution
 +
Real displacement[N], reaction[N];
 +
Real check[3];
 +
 +
Real stress1[Trusses];
 +
Real safety[Trusses];
 +
Real dis[3];
 +
Real Str[3];
 +
 
 +
protected
 +
parameter Integer N=3*Points;
 +
Real q1[3], q2[3], g[N,N], G[N,N], G_star[N,N], id[N,N]=identity(N), cx, cy, cz, L, X[3,3];
 +
Real err=10e-10, ers=10e-4;
 +
 +
algorithm
 +
//Creating Global Matrix
 +
G:=id;
 +
for i in 1:Trusses loop
 +
  for j in 1:3 loop
 +
  q1[j]:=P[C[i,1],j];
 +
  q2[j]:=P[C[i,2],j];
 +
  end for;
 +
     
 +
    //Solving Matrix
 +
    L:=Modelica.Math.Vectors.length(q2-q1);
 +
    cx:=(q2[1]-q1[1])/L;
 +
    cy:=(q2[2]-q1[2])/L;
 +
    cz:=(q2[3]-q1[3])/L;
 +
    X:=(Area*Elas/L)*[cx^2,cx*cy,cx*cz;
 +
                      cy*cx,cy^2,cy*cz;
 +
                      cz*cx,cz*cy,cz^2];
 +
 +
    //Transforming to global matrix
 +
    g:=zeros(N,N);
 +
    for m,n in 1:3 loop
 +
      g[3*(C[i,1]-1)+m,3*(C[i,1]-1)+n]:=X[m,n];
 +
      g[3*(C[i,2]-1)+m,3*(C[i,2]-1)+n]:=X[m,n];
 +
      g[3*(C[i,2]-1)+m,3*(C[i,1]-1)+n]:=-X[m,n];
 +
      g[3*(C[i,1]-1)+m,3*(C[i,2]-1)+n]:=-X[m,n];
 +
    end for; 
 +
 +
  G_star:=G+g;
 +
  G:=G_star;
 +
end for;
 +
 +
//Implementing boundary
 +
for x in 1:Points loop
 +
  if P[x,4] <> 0 then
 +
    for a in 1:Points*3 loop
 +
      G[(x*3)-2,a]:=0;
 +
      G[(x*3)-2,(x*3)-2]:=1;
 +
    end for;
 +
  end if;
 +
  if P[x,5] <> 0 then
 +
    for a in 1:Points*3 loop
 +
      G[(x*3)-1,a]:=0;
 +
      G[(x*3)-1,(x*3)-1]:=1;
 +
    end for;
 +
  end if;
 +
  if P[x,6] <> 0 then
 +
    for a in 1:Points*3 loop
 +
      G[x*3,a]:=0;
 +
      G[x*3,x*3]:=1;
 +
    end for;
 +
  end if;
 +
end for;
 +
 +
//Solving displacement
 +
displacement:=Modelica.Math.Matrices.solve(G,F);
 +
 +
//Solving reaction
 +
reaction:=(G_star*displacement)-F;
 +
 +
//Eliminating float error
 +
for i in 1:N loop
 +
  reaction[i]:=if abs(reaction[i])<=err then 0 else reaction[i];
 +
  displacement[i]:=if abs(displacement[i])<=err then 0 else displacement[i];
 +
end for;
 +
 +
//Checking Force
 +
check[1]:=sum({reaction[i] for i in (1:3:(N-2))})+sum({F[i] for i in (1:3:(N-2))});
 +
check[2]:=sum({reaction[i] for i in (2:3:(N-1))})+sum({F[i] for i in (2:3:(N-1))});
 +
check[3]:=sum({reaction[i] for i in (3:3:N)})+sum({F[i] for i in (3:3:N)});
 +
 
 +
for i in 1:3 loop
 +
  check[i] := if abs(check[i])<=ers then 0 else check[i];
 +
end for;
 +
 +
//Calculating stress in each truss
 +
for i in 1:Trusses loop
 +
for j in 1:3 loop
 +
  q1[j]:=P[C[i,1],j];
 +
  q2[j]:=P[C[i,2],j];
 +
  dis[j]:=abs(displacement[3*(C[i,1]-1)+j]-displacement[3*(C[i,2]-1)+j]);
 +
end for;
 +
     
 +
    //Solving Matrix
 +
    L:=Modelica.Math.Vectors.length(q2-q1);
 +
    cx:=(q2[1]-q1[1])/L;
 +
    cy:=(q2[2]-q1[2])/L;
 +
    cz:=(q2[3]-q1[3])/L;
 +
    X:=(Elas/L)*[cx^2,cx*cy,cx*cz;
 +
                cy*cx,cy^2,cy*cz;
 +
                cz*cx,cz*cy,cz^2];
 +
   
 +
    Str:=(X*dis);
 +
    stress1[i]:=Modelica.Math.Vectors.length(Str);
 +
end for;
 +
 +
//Safety factor
 +
for i in 1:Trusses loop
 +
  if stress1[i]>0 then
 +
    safety[i]:=Yield/stress1[i];
 +
  else
 +
    safety[i]:=0;
 +
  end if;
 +
end for;
 +
 +
end Trusses_3D_Tugas_Besar_Safety;
 +
 
 +
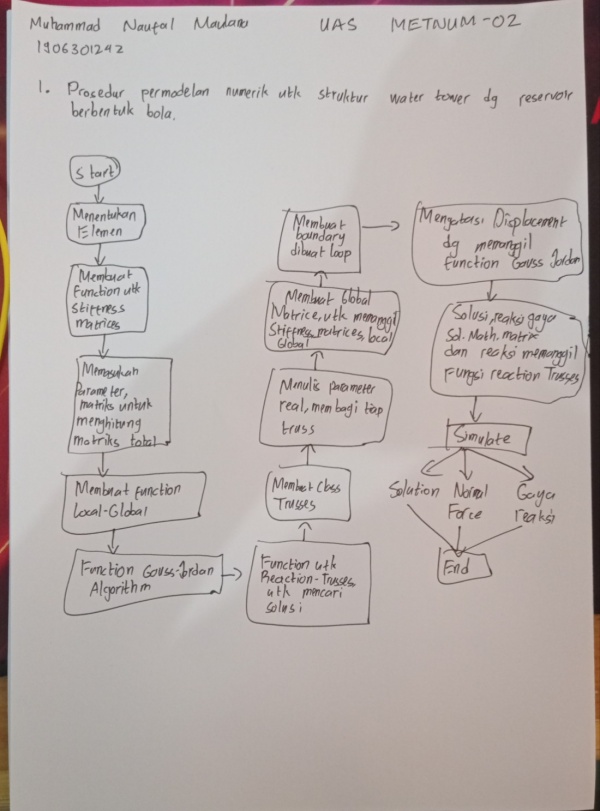
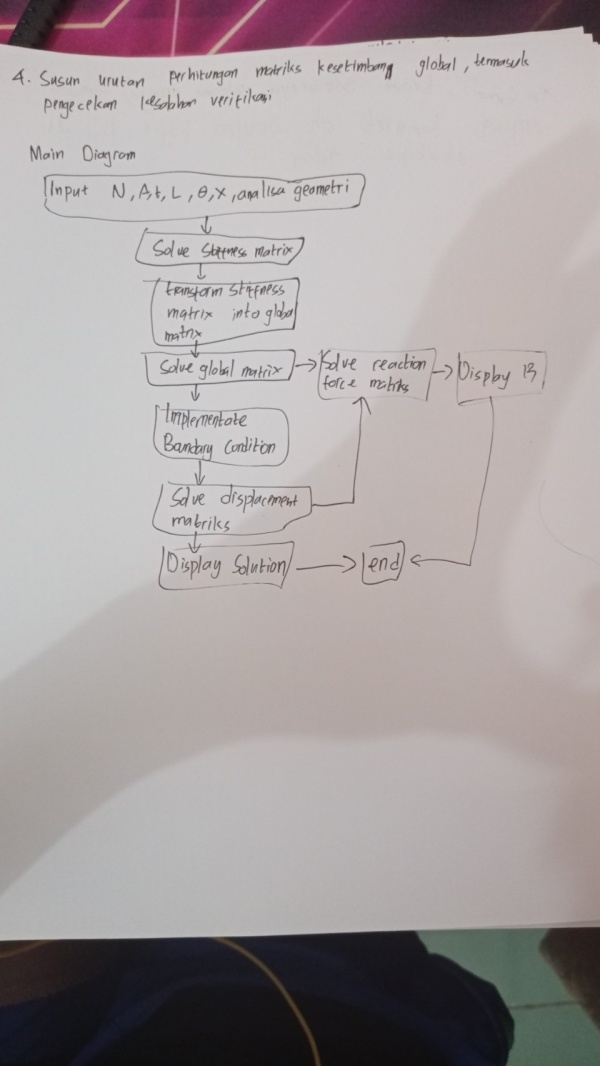
== JAWABAN UAS ==
 +
 
 +
[[File:UAS 1 MNM.jpeg|600px|center]]
 +
[[File:UAS 2 MNM.jpeg|600px|center]]
 +
[[File:UAS 3 MNM.jpeg|600px|center]]
 +
[[File:UAS 4 MNM.jpeg|600px|center]]
 +
[[File:UAS 5 MNM.jpeg|600px|center]]
 +
[[File:UAS 6 MNM.jpeg|600px|center]]

Latest revision as of 18:01, 13 January 2021

PERKENALAN SINGKAT

Saya Muhammad Naufal Maulana, mahasiswa teknik mesin UI angkatan 2019. Saya tertarik dengan teknik mesin karena memiliki prospek kerja yang luas. Materi yang sudah saya pelajari sebelum uts ini adalah mengenai turunan numerik, deret mclaurin , interpolasi, regresi, pengertian dari metode numerik, pseucode.

MINGGU KE 1

Tujuan mempelajari metode numerik

  • Memahami konsep-konsep dan prinsip-prinsip dasar dalam metode numerik seperti:Persamaan algoritma, aljabar, pencocokan kurva, persamaan diferensial parsial, dan lainnya
  • Mengerti dan mampu menerapkan pemahaman atau aplikasi terhadap konsep metode numerik
  • Mampu menerapkan metode numerik dalam hal terkait persoalan keteknikan
  • Mendapat added value (nilai tambah)/adab sehingga mahasiswa menjadi orang yang memiliki budi pekerti yang baik

TUGAS 1

Pada pertemuan sebelumnya, saya diberi tugas membuat video terkait penggunaan aplikasi open modelica. Berikut ini adalah hasil tugas saya:

MINGGU KE 2

Pertemuan kedua bersama Pak Dai, Kami diminta menjadi pribadi yang lebih baik dari hari kemarin. Setelah itu, kami diminta untuk mempresentasikan tugas kami sebelumnya. Setelah itu materi yang diberikan pada pertemuan ini adalah penggunaan class untuk memanggil sebuah function. Function yang bisa dipanggil beragam jumlahnya, mulai dari penjumlahan sederhana sampai operasi eliminasi matriks.

TUGAS 2

Di akhir kelas, kami diberi tugas untuk membuat video mengenai cara menyelesaikan persamaan aljabar simultan dengan OpenModelica, dengan metode yang telah diajarkan sebelumnya. Berikut ini adalah hasil tugas saya:

MINGGU KE 3

Pada pertemuan ketiga dilakukan pembahasan tentang penggunaan OpenModelica pada permasalahan engineering. Pada permasalahan engineering, metode numerik dapat membantu menyelesaikan persoalan lebih cepat. Contoh metode numerik yang dapat membantu menyelesaikan permasalaha engineering diantaranya Metode Stokastik, CFD(Computation fluid dynamics, dan FEA ( FInite Element Analaysis). Tahapan untuk menyelesaikan masalah engineering menggunakan metode numerik:

  • 1. Melakukan analisis terhadap masalah engineering
  • 2. Melakukan pemodelan matematis dari masalah yang sudah dianalisis
  • 3. Menerjemahkan pemodelan matematis yang sudah dibuat menjadi metode numerik agar bisa dhitung oleh komputer
  • 4. Melakukan perhitungan metode numerik menggunakan software dan dihasilkanlah solusi dari masalah engineering tersebut

TUGAS 3

Mencari Truss Analysis menggunakan OpenModelica

Chapter 3 Problem 4 (Finite Element Analysis, 4th Edition, Saeed Moaveni).png
Grafik Reaction Forces
Grafik Displacement

Persamaan 

class Trusses_HW

parameter Integer N=8; //Global matrice = 2*points connected
parameter Real A=0.001; //Area m2
parameter Real E=200e9; //Pa
Real G[N,N]; //global
Real Ginitial[N,N]; //global
Real Sol[N]; //global dispplacement
Real X[N]={0,0,-1035.2762,-3863.7033,0,0,-1035.2762,-3863.7033};
Real R[N]; //global reaction force
Real SolMat[N,1];
Real XMat[N,1];

//boundary condition
Integer b1=1;
Integer b2=3;

//truss 1
parameter Real X1=0; //degree between truss
Real k1=A*E/1;
Real K1[4,4]; //stiffness matrice
Integer p1a=1;
Integer p1b=2;
Real G1[N,N];

//truss 2
parameter Real X2=0; //degree between truss
Real k2=A*E/1;
Real K2[4,4]; //stiffness matrice
Integer p2a=2;
Integer p2b=3;
Real G2[N,N];

//truss 3
parameter Real X3=90; //degree between truss
Real k3=A*E/1.25;
Real K3[4,4]; //stiffness matrice
Integer p3a=2;
Integer p3b=4;
Real G3[N,N];

//truss 4
parameter Real X4=90+38.6598; //degree between truss
Real k4=A*E/1.6;
Real K4[4,4]; //stiffness matrice
Integer p4a=1;
Integer p4b=4;
Real G4[N,N];

//truss 5
parameter Real X5=90-38.6598; //degree between truss
Real k5=A*E/1.6;
Real K5[4,4]; //stiffness matrice
Integer p5a=3;
Integer p5b=4;
Real G5[N,N];

/*
for each truss, please ensure pXa is lower then pXb (X represents truss element number)
*/

algorithm

//creating global matrice
K1:=Stiffness_Matrices(X1);
G1:=k1*Local_Global(K1,N,p1a,p1b);

K2:=Stiffness_Matrices(X2);
G2:=k2*Local_Global(K2,N,p2a,p2b);

K3:=Stiffness_Matrices(X3);
G3:=k3*Local_Global(K3,N,p3a,p3b);

K4:=Stiffness_Matrices(X4);
G4:=k4*Local_Global(K4,N,p4a,p4b);

K5:=Stiffness_Matrices(X5);
G5:=k5*Local_Global(K5,N,p5a,p5b);

G:=G1+G2+G3+G4+G5;
Ginitial:=G;

//implementing boundary condition
for i in 1:N loop
 G[2*b1-1,i]:=0;
 G[2*b1,i]:=0;
 G[2*b2-1,i]:=0;
 G[2*b2,i]:=0;
end for;

G[2*b1-1,2*b1-1]:=1;
G[2*b1,2*b1]:=1;
G[2*b2-1,2*b2-1]:=1;
G[2*b2,2*b2]:=1;

//solving displacement
Sol:=Gauss_Jordan(N,G,X);

//solving reaction force
SolMat:=matrix(Sol);
XMat:=matrix(X);
R:=Reaction_Trusses(N,Ginitial,SolMat,XMat);

end Trusses_HW;

Fungsi Panggil

Matrice Transformation 


function Stiffness_Matrices
input Real A;
Real Y;
output Real X[4,4];
Real float_error = 10e-10;

final constant Real pi=2*Modelica.Math.asin(1.0);

algorithm

Y:=A/180*pi;
    
X:=[(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y);

Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2;

-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y);

-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2];

for i in 1:4 loop
 for j in 1:4 loop
   if abs(X[i,j]) <= float_error then
     X[i,j] := 0;
   end if;
 end for;
end for;

end Stiffness_Matrices;

Global Element Matrice 

function Local_Global
input Real Y[4,4];
input Integer B;
input Integer p1;
input Integer p2;
output Real G[B,B];

algorithm

for i in 1:B loop
 for j in 1:B loop
     G[i,j]:=0;
 end for;
end for;

G[2*p1,2*p1]:=Y[2,2];
G[2*p1-1,2*p1-1]:=Y[1,1];
G[2*p1,2*p1-1]:=Y[2,1];
G[2*p1-1,2*p1]:=Y[1,2];

G[2*p2,2*p2]:=Y[4,4];
G[2*p2-1,2*p2-1]:=Y[3,3];
G[2*p2,2*p2-1]:=Y[4,3];
G[2*p2-1,2*p2]:=Y[3,4];

G[2*p2,2*p1]:=Y[4,2];
G[2*p2-1,2*p1-1]:=Y[3,1];
G[2*p2,2*p1-1]:=Y[4,1];
G[2*p2-1,2*p1]:=Y[3,2];

G[2*p1,2*p2]:=Y[2,4];
G[2*p1-1,2*p2-1]:=Y[1,3];
G[2*p1,2*p2-1]:=Y[2,3];
G[2*p1-1,2*p2]:=Y[1,4];

end Local_Global;


Reaction Matrices Equation 

function Reaction_Trusses
input Integer N;
input Real A[N,N];
input Real B[N,1];
input Real C[N,1];
Real X[N,1];
output Real Sol[N];
Real float_error = 10e-10;

algorithm
X:=A*B-C;

for i in 1:N loop
 if abs(X[i,1]) <= float_error then
   X[i,1] := 0;
 end if;
end for;

for i in 1:N loop
 Sol[i]:=X[i,1];
end for;

end Reaction_Trusses;

Gauss Jordan 

function Gauss_Jordan
input Integer N;
input Real A[N,N];
input Real B[N];
output Real X[N];
Real float_error = 10e-10;
algorithm
X:=Modelica.Math.Matrices.solve(A,B);
for i in 1:N loop
  if abs(X[i]) <= float_error then
    X[i] := 0;
  end if;
end for;
end Gauss_Jordan;

TUGAS BESAR

Tugas besar yang diberikan untuk kelas metnum 02 dan 03 adalah melakukan optimasi pada rangka sederhana:

Soal Tugas Besar.jpg

Dengan data sebagai berikut:

Data Tugas Besar.jpg

Pada soal ini kami diharapkan dapat mendesain rangka batang dengan harga terjangkau dengan fungsi yang optimal.

Faktor atau variabel bebasnya meliputi : Harga, Material yang digunakan, cross section Dilakukan dengan metode optimasi dan membentuk curve fitting pada variabel harga

Constrain

- Spesifikasi L (Panjang) dan geometri rangka truss

- Gaya beban diberikan kepada struktur (1000 N dan 2000 N)


Asumsi

- Variasi Stiffness terikat dengan variabel area. Memvariasikan Elastisitas tergolong sulit karena setiap material memiliki range yang tidak teratur dan dalam satu material yang sejenis (struktur biaya tetap) tidak terjadi perubahan nilai elastisitas yang berbanding lurus dengan perubahan biaya.

- Beban akan terdistribusi hanya pada point penghubung Untuk perhitungan displacement, reaction force, dan stress 

model Trusses_3D_Tugas_Besar_Safety

//define initial variable
parameter Integer Points=size(P,1); //Number of Points
parameter Integer Trusses=size(C,1); //Number of Trusses
parameter Real Yield=275e6; //Yield Strength (Pa)
parameter Real Area=0.000224;   //Area L Profile (Dimension=0.03, Thickness=0,004) (m2)
parameter Real Elas=193e9;     //Elasticity SS 302  (Pa)

//define connection
parameter Integer C[:,2]=[1,5; 
                          2,6;
                          3,7;
                          4,8;
                          5,6;  //1st floor
                          6,7;  //1st floor
                          7,8;  //1st floor
                          5,8;  //1st floor
                          5,9;
                         6,10;
                         7,11;
                         8,12;
                         9,10; //2nd floor
                         10,11;//2nd floor 
                         11,12;//2nd floor
                          9,12; //2nd floor
                          9,13;
                         10,14;
                         11,15;
                         12,16;
                         13,14;//3rd floor
                         14,15;//3rd floor
                         15,16;//3rd floor
                        13,16];//3rd floor
                                                              
//define coordinates (please put orderly)
parameter Real P[:,6]=[0.3,-0.375,0,1,1,1;     //1
                       -0.3,-0.375,0,1,1,1;    //2
                       -0.3,0.375,0,1,1,1;     //3
                       0.3,0.375,0,1,1,1;      //4
                           
                       0.3,-0.375,0.6,0,0,0;   //5
                       -0.3,-0.375,0.6,0,0,0;  //6
                       -0.3,0.375,0.6,0,0,0;   //7
                       0.3,0.375,0.6,0,0,0;    //8
                            
                       0.3,-0.375,1.2,0,0,0;   //9
                       -0.3,-0.375,1.2,0,0,0;  //10  
                       -0.3,0.375,1.2,0,0,0;   //11
                       0.3,0.375,1.2,0,0,0;    //12
                            
                       0.3,-0.375,1.8,0,0,0;   //13
                       -0.3,-0.375,1.8,0,0,0;  //14
                       -0.3,0.375,1.8,0,0,0;   //15
                       0.3,0.375,1.8,0,0,0];   //16
                          
//define external force (please put orderly)
parameter Real F[Points*3]={0,0,0,
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,-500, 
                            0,0,-1000, 
                            0,0,-1000, 
                            0,0,-500}; 

//solution
Real displacement[N], reaction[N];
Real check[3];

Real stress1[Trusses];
Real safety[Trusses];
Real dis[3];
Real Str[3];
 
protected
parameter Integer N=3*Points;
Real q1[3], q2[3], g[N,N], G[N,N], G_star[N,N], id[N,N]=identity(N), cx, cy, cz, L, X[3,3];
Real err=10e-10, ers=10e-4;

algorithm
//Creating Global Matrix
G:=id;
for i in 1:Trusses loop
 for j in 1:3 loop
  q1[j]:=P[C[i,1],j];
  q2[j]:=P[C[i,2],j];
 end for;
      
   //Solving Matrix
   L:=Modelica.Math.Vectors.length(q2-q1);
   cx:=(q2[1]-q1[1])/L;
   cy:=(q2[2]-q1[2])/L;
   cz:=(q2[3]-q1[3])/L; 
   X:=(Area*Elas/L)*[cx^2,cx*cy,cx*cz;
                     cy*cx,cy^2,cy*cz;
                     cz*cx,cz*cy,cz^2];

   //Transforming to global matrix
   g:=zeros(N,N); 
   for m,n in 1:3 loop
     g[3*(C[i,1]-1)+m,3*(C[i,1]-1)+n]:=X[m,n];
     g[3*(C[i,2]-1)+m,3*(C[i,2]-1)+n]:=X[m,n];
     g[3*(C[i,2]-1)+m,3*(C[i,1]-1)+n]:=-X[m,n];
     g[3*(C[i,1]-1)+m,3*(C[i,2]-1)+n]:=-X[m,n];
   end for;  

 G_star:=G+g;
 G:=G_star;
end for;

//Implementing boundary
for x in 1:Points loop
 if P[x,4] <> 0 then
   for a in 1:Points*3 loop
     G[(x*3)-2,a]:=0;
     G[(x*3)-2,(x*3)-2]:=1;
   end for;
 end if;
 if P[x,5] <> 0 then
   for a in 1:Points*3 loop
     G[(x*3)-1,a]:=0;
     G[(x*3)-1,(x*3)-1]:=1;
   end for;
 end if;
 if P[x,6] <> 0 then
   for a in 1:Points*3 loop
     G[x*3,a]:=0;
     G[x*3,x*3]:=1;
   end for;
 end if;
end for;

//Solving displacement
displacement:=Modelica.Math.Matrices.solve(G,F);

//Solving reaction
reaction:=(G_star*displacement)-F;

//Eliminating float error
for i in 1:N loop
 reaction[i]:=if abs(reaction[i])<=err then 0 else reaction[i];
 displacement[i]:=if abs(displacement[i])<=err then 0 else displacement[i];
end for;

//Checking Force
check[1]:=sum({reaction[i] for i in (1:3:(N-2))})+sum({F[i] for i in (1:3:(N-2))});
check[2]:=sum({reaction[i] for i in (2:3:(N-1))})+sum({F[i] for i in (2:3:(N-1))});
check[3]:=sum({reaction[i] for i in (3:3:N)})+sum({F[i] for i in (3:3:N)});
  
for i in 1:3 loop
 check[i] := if abs(check[i])<=ers then 0 else check[i];
end for;

//Calculating stress in each truss
for i in 1:Trusses loop
for j in 1:3 loop
  q1[j]:=P[C[i,1],j];
  q2[j]:=P[C[i,2],j];
  dis[j]:=abs(displacement[3*(C[i,1]-1)+j]-displacement[3*(C[i,2]-1)+j]);
end for;
      
   //Solving Matrix
   L:=Modelica.Math.Vectors.length(q2-q1);
   cx:=(q2[1]-q1[1])/L;
   cy:=(q2[2]-q1[2])/L;
   cz:=(q2[3]-q1[3])/L; 
   X:=(Elas/L)*[cx^2,cx*cy,cx*cz;
                cy*cx,cy^2,cy*cz;
                cz*cx,cz*cy,cz^2];
   
   Str:=(X*dis);
   stress1[i]:=Modelica.Math.Vectors.length(Str);
end for;

//Safety factor
for i in 1:Trusses loop
 if stress1[i]>0 then
   safety[i]:=Yield/stress1[i];
 else
   safety[i]:=0;
 end if; 
end for;

end Trusses_3D_Tugas_Besar_Safety;

JAWABAN UAS

UAS 1 MNM.jpeg
UAS 2 MNM.jpeg
UAS 3 MNM.jpeg
UAS 4 MNM.jpeg
UAS 5 MNM.jpeg
UAS 6 MNM.jpeg
Retrieved from "http://air.eng.ui.ac.id/index.php?title=Muhammad_Naufal_Maulana&oldid=55180"