Difference between revisions of "Umar"

From ccitonlinewiki
Jump to: navigation, search
(→‎Contoh Soal Truss)
(→‎Ujian Akhir Semester)
 
(64 intermediate revisions by the same user not shown)
Line 52: Line 52:
 
* Setelah itu cari penyelesaian dengan bantuan komputer untuk mendapatkan ''output'' yang diinginkan
 
* Setelah itu cari penyelesaian dengan bantuan komputer untuk mendapatkan ''output'' yang diinginkan
  
−
Agar Kami bisa lebih paham tentang dasar-dasar metode numerik, Pak Dai menyuruh Kami untuk mencoba membuat fungsi untuk menyelesaikan Persamaan 9.12 di buku ''Numerical Methods for Engineers 7th Edition'' oleh Chapra dengan cara apapun (misalnya eliminasi gauss). Kedua, Kami disuru latihan menyelesaikan sistem persamaan dengan membuat fungsi penyelesaian dengan cara pseudocode 9.4 untuk menjawab soal 9.5 yang ada di buku yang sama juga. Latihan yang kedua ini dimaksudkan agar Kami paham dalam penggunaan ''array'' dalam penggunaan OpenModelica, yang dimana ''array'' ini dapat memudahkan mengumpulkan himpunan penyelesaian.
+
Agar Kami bisa lebih paham tentang dasar-dasar metode numerik, Pak Dai menyuruh Kami untuk mencoba membuat fungsi untuk menyelesaikan Persamaan 9.12 di buku ''Numerical Methods for Engineers 7th Edition'' oleh Chapra dengan cara apapun (misalnya eliminasi gauss). Kedua, Kami disuru latihan menyelesaikan sistem persamaan dengan membuat fungsi penyelesaian dengan cara pseudocode 9.4 untuk menjawab soal 9.5 yang ada di buku yang sama juga. Latihan yang kedua ini dimaksudkan agar Kami paham dalam penggunaan ''array'' dalam penggunaan OpenModelica, yang dimana ''array'' ini dapat memudahkan mengumpulkan himpunan penyelesaian. Brikut adalah fungsi Gauss Jordan di OpenModelica
  
  
−
== Contoh Soal Truss ==
+
function GaussJordan
 +
 +
input Real [:,:] A;
 +
output Real [:,:] B;
 +
 +
protected // untuk local variable
 +
Integer h = 1;    //pivot row
 +
Integer k = 1;    //pivot coloumn
 +
Integer m = size(A,1); //Number of row
 +
Integer n = size(A,2); //Number of column
 +
Integer c = 0;
 +
Integer max_row; // Row index of max number in pivot column
 +
 +
Real [:] pivot_column;
 +
Real [:] pivot_row;
 +
Real [:,:] temp_array;
 +
Real r;
 +
 +
Real float_error = 10e-10;
 +
 +
 +
algorithm
 +
 +
//fungsi input A dan output B
 +
B := A;
 +
 
 +
while h <= m and k <= n loop
 +
 +
  for i in 1 : m loop
 +
    for j in 1 : n loop
 +
      if abs(B[i,j]) <= float_error then
 +
        B[i,j] := 0;
 +
      end if;
 +
    end for;
 +
  end for;
 +
 +
//Finding pivot
 +
  pivot_column:= {B[i,h] for i in h:m};
 +
 
 +
    //Mencari baris terbawah yang mempunyai nilai pivot tertinggi
 +
    c:=h-1;
 +
    for element in pivot_column loop
 +
      c:= c+1;
 +
      if abs(element)== max(abs(pivot_column)) then
 +
        max_row :=c;
 +
      end if;
 +
    end for;
 +
   
 +
  //Jika tidak ada pivot di kolom ini, pindah ke kolom selanjutnya
 +
  if B[max_row,k] == 0 then
 +
    k:=k+1;
 +
   
 +
  else
 +
    // tukar row h - max_row
 +
    temp_array := B;
 +
    temp_array[h] := B[max_row];
 +
    temp_array[max_row] := B[h];
 +
    B:= temp_array;
 +
   
 +
    //devide pivot row by pivot number
 +
      B[h] := B[h]/B[h,k];
 +
     
 +
      for i in (h+1) :m loop
 +
        r := B[i,k]/B[h,k];
 +
     
 +
      B[i,k]:=0;
 +
     
 +
      for j in (k+1) : n loop
 +
        B[i,j] := B[i,j]-B[h,j] * r;
 +
      end for;
 +
    end for;
 +
   
 +
    //move ke pivot kolom dan row selanjutnya
 +
    h := h+1;
 +
    k := k+1;
 +
   
 +
  end if;
 +
 
 +
end while;
 +
 +
// proses dari kanan atas
 +
h :=m;
 +
k :=n;
 +
 +
while h >=1 and k>=1 loop
 +
 
 +
  //dealing with error
 +
  for i in 1:m loop
 +
    for j in 1:n loop
 +
      if abs(B[i,j]) <=float_error then
 +
        B[i,j]:=0;
 +
      end if;
 +
    end for;
 +
  end for;
 +
 +
//finding pivot
 +
    pivot_row := {B[h,i] for i in 1:k};
 +
   
 +
    //Get position index k of pivot
 +
    c := 0;
 +
    for element in pivot_row loop
 +
      c := c+1;
 +
      if element <> 0 then
 +
        break;
 +
      end if;
 +
    end for;
 +
    k:= c;
 +
   
 +
  // no pivot in this row, move to next row
 +
  if B[h,k] == 0 then
 +
    h:= h-1;
 +
   
 +
  else
 +
    //perform row operatation
 +
    for i in 1:(h-1) loop
 +
      r := B[i,k];
 +
      B[i] := B[i] - B[h] *r;
 +
    end for;
 +
   
 +
    //move to next pivot row dan column
 +
    h:=h+1;
 +
    k:=k+1;
 +
   
 +
  end if;
 +
 
 +
end while;
 +
   
 +
     
 +
end GaussJordan;
 +
 
 +
 
 +
=== '''Contoh Soal Truss''' ===
  
  
 
[[File:Example 3.1 RS.jpg|500px|thumb|centre]]
 
[[File:Example 3.1 RS.jpg|500px|thumb|centre]]
−
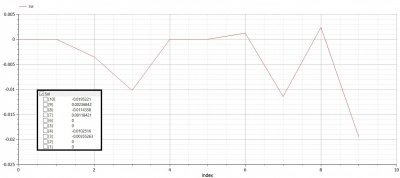
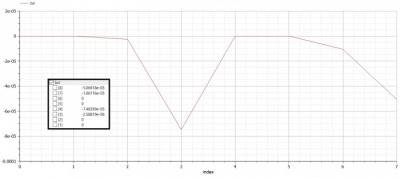
[[File:Soal Trusses 1 Displacement RS.jpg|700px|thumb|right|Grafik Displacement]]
+
[[File:Soal Trusses 1 Displacement RS.jpg|400px|thumb|right|Grafik Displacement]]
−
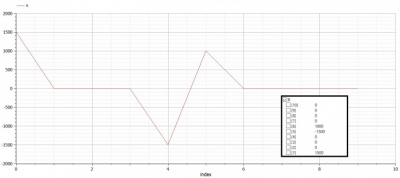
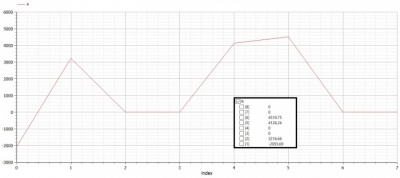
[[File:Soal Trusses 1 Reaction RS.jpg|700px|thumb|right|Grafik Reaction Forces]]
+
[[File:Soal Trusses 1 Reaction RS.jpg|400px|thumb|right|Grafik Reaction Forces]]
−
{| class="wikitable"
 
−
|-
 
−
| style='border-style: none  none  solid  solid;' |
 
  
 
'''Kodingan'''
 
'''Kodingan'''
Line 182: Line 310:
 
   
 
   
 
  end Trusses;
 
  end Trusses;
−
 
  
 
=== Tugas Pertemuan 3 ===
 
=== Tugas Pertemuan 3 ===
Line 188: Line 315:
 
[[File:soal statur.png|400px|thumb|center]]
 
[[File:soal statur.png|400px|thumb|center]]
  
−
'''code'''
+
'''Kodingan'''
−
[[File:Soal Trusses 2 Displacement RS.jpg|700px|thumb|right|Grafik Displacement]]
+
[[File:Soal Trusses 2 Displacement RS.jpg|400px|thumb|right|Grafik Displacement]]
−
[[File:Soal Trusses 2 Reaction RS.jpg|700px|thumb|right|Grafik Reaction Forces]]
+
[[File:Soal Trusses 2 Reaction RS.jpg|400px|thumb|right|Grafik Reaction Forces]]
 
{| class="wikitable"
 
{| class="wikitable"
 
|-
 
|-
Line 406: Line 533:
  
 
|}
 
|}
 +
 +
 +
== '''Pertemuan 4 (2 Desember 2020)''' ==
 +
 +
=== '''Kuis : Membuat class diagram dan flowchart setiap class''' ===
 +
 +
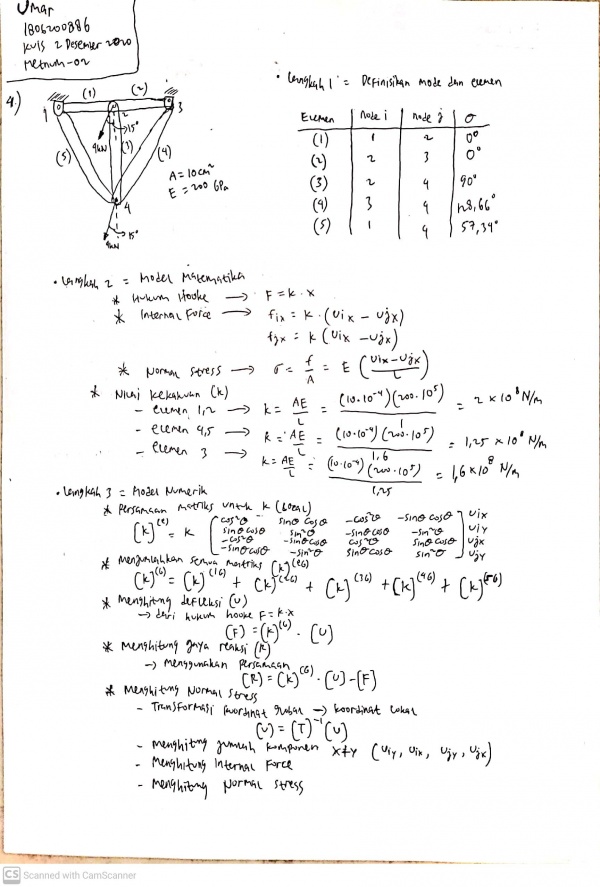
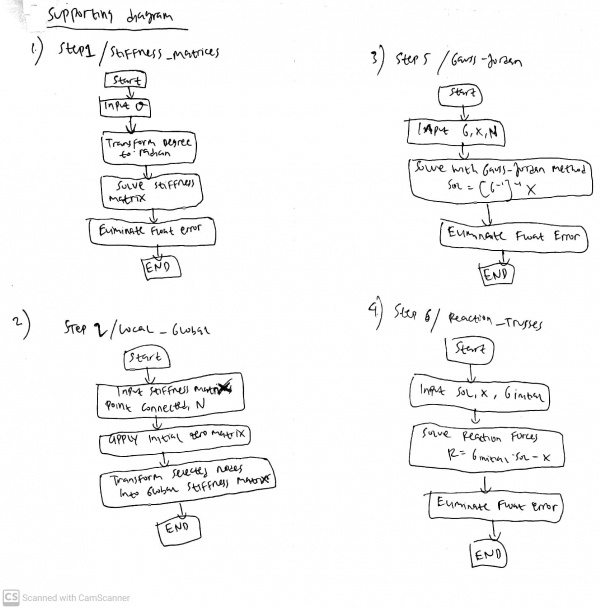
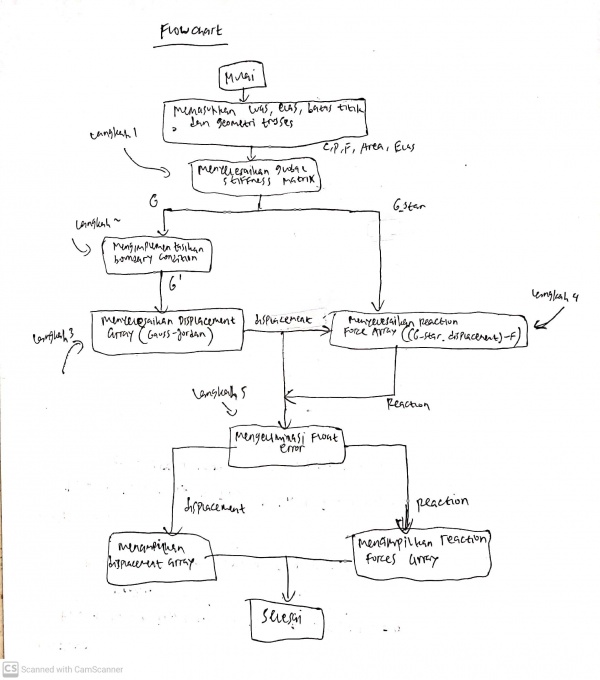
[[File:Perhitungan.jpg|600px|thumb|center]]
 +
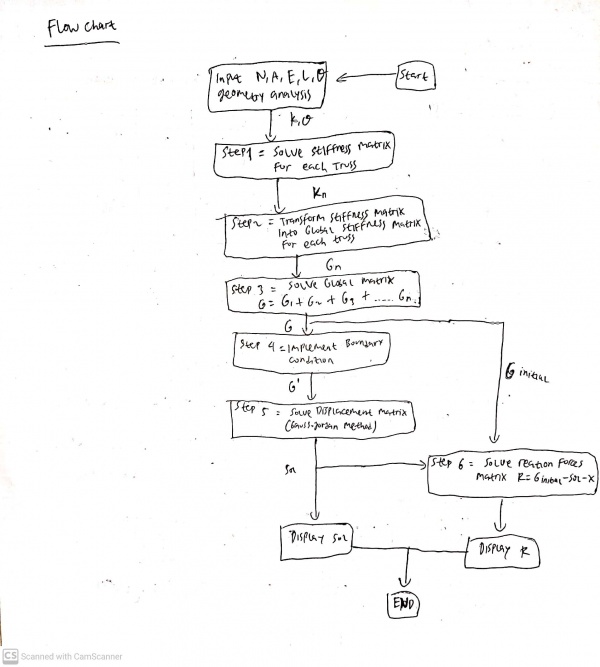
[[File:Flowchartt.jpg|600px|thumb|center]]
 +
[[File:supportingdiagramm.jpg|600px|thumb|center]]
 +
 +
=== '''Tugas Pertemuan 4''' ===
 +
 +
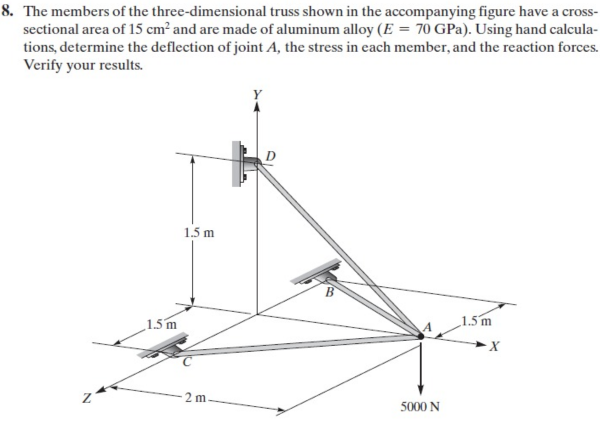
=== '''Soal''' ===
 +
[[File:pr4metnum.png|600px|thumb|center]]
 +
 +
=== '''Flow Chart''' ===
 +
[[File:flowcharttt.jpg|600px|thumb|center]]
 +
 +
=== '''Perhitungan di OpenModelica''' ===
 +
 +
'''Berikut kodingannya :'''
 +
 +
class Trusses3D_Tes
 +
 +
//define initial variable
 +
parameter Integer Points=4; //Number of Points
 +
parameter Integer Trusses=3; //Number of Trusses
 +
parameter Real Area=0.0015; //Area
 +
parameter Real Elas=70e9; //Elasticity
 +
 +
//define connection
 +
parameter Integer C[Trusses,2]=[1,2;
 +
                                1,3;
 +
                                1,4];
 +
                             
 +
//define coordinates (please put orderly)
 +
parameter Real P[Points,3]=[2,0,0;
 +
                            0,0,1.5;
 +
                            0,0,-1.5;
 +
                            0,1.5,0];
 +
 +
//define external force (please put orderly)
 +
parameter Real F[Points*3]={0,-5000,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0};
 +
 +
//define boundary
 +
parameter Integer b[:]={2,3,4};
 +
 +
//solution
 +
Real displacement[N], reaction[N];
 +
 +
protected
 +
parameter Integer N=3*Points;
 +
Integer boundary[3*size(b,1)]=cat(1,(3*b).-2,(3*b).-1,3*b);
 +
Real q1[3], q2[3], g[N,N], G[N,N], G_star[N,N], id[N,N]=identity(N), err=10e-10, cx, cy, cz, L, X[3,3];
 +
 +
algorithm
 +
//Creating Global Matrix
 +
G:=id;
 +
for i in 1:Trusses loop
 +
  for j in 1:3 loop
 +
    q1[j]:=P[C[i,1],j];
 +
    q2[j]:=P[C[i,2],j];
 +
  end for;
 +
 +
        //Solving Matrix
 +
        L:=Modelica.Math.Vectors.length(q2-q1);
 +
        cx:=(q2[1]-q1[1])/L;
 +
        cy:=(q2[2]-q1[2])/L;
 +
        cz:=(q2[3]-q1[3])/L;
 +
        X:=(Area*Elas/L)*[cx^2,cx*cy,cx*cz;
 +
                          cy*cx,cy^2,cy*cz;
 +
                          cz*cx,cz*cy,cz^2];
 +
 +
        //Transforming to global matrix
 +
        g:=zeros(N,N);
 +
        for m,n in 1:3 loop
 +
          g[3*(C[i,1]-1)+m,3*(C[i,1]-1)+n]:=X[m,n];
 +
          g[3*(C[i,2]-1)+m,3*(C[i,2]-1)+n]:=X[m,n];
 +
          g[3*(C[i,2]-1)+m,3*(C[i,1]-1)+n]:=-X[m,n];
 +
          g[3*(C[i,1]-1)+m,3*(C[i,2]-1)+n]:=-X[m,n];
 +
        end for; 
 +
 
 +
  G_star:=G+g;
 +
  G:=G_star;
 +
end for;
 +
 +
//Implementing boundary
 +
for i in boundary loop
 +
  for j in 1:N loop
 +
    G[i,j]:=id[i,j];
 +
  end for;
 +
end for;
 +
 +
//Solving displacement
 +
displacement:=Modelica.Math.Matrices.solve(G,F);
 +
 +
//Solving reaction
 +
reaction:=(G_star*displacement)-F;
 +
 +
//Eliminating float error
 +
for i in 1:N loop
 +
  reaction[i]:=if abs(reaction[i])<=err then 0 else reaction[i];
 +
  displacement[i]:=if abs(displacement[i])<=err then 0 else displacement[i];
 +
end for;
 +
 +
end Trusses3D_Tes;
 +
 +
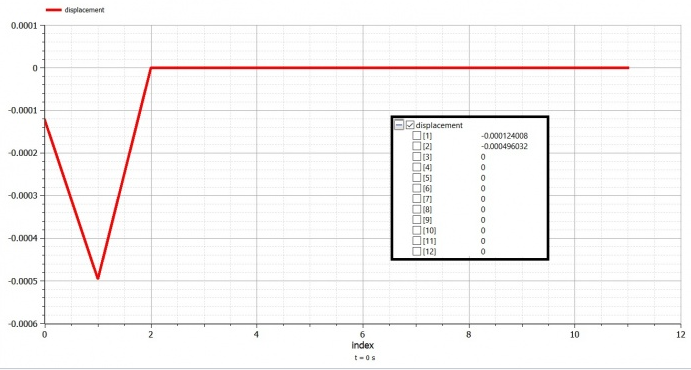
[[File:3D grf1.png|700px|thumb|center]]
 +
[[File:3D grf.png|700px|thumb|center]]
 +
 +
=== '''Verifikasi Perhitungan''' ===
 +
[[File:Verifikasi.jpg|600px|thumb|center|]]
 +
 +
== '''Pertemuan 5 (16 Desember 2020)''' ==
 +
Pada pertemuan kelima, Kami diajarkan mengenai pengaplikasian metode numerik dalam optimasi sebuah sistem menggunakan OpenModelica. Materi ini dijelaskan melalui sebuah video oleh Bu Candra serta pseudocode dari persoalan tersebut, contoh persoalan tersebut adalah optimasi menggunakan metode Bracket. Berikut adalah pseudocode yang di OpenModelica :
 +
 +
'''FungsiObjek.mo'''
 +
function FungsiObjek
 +
 
 +
input Real x;
 +
output Real y;
 +
 +
algorithm
 +
 +
y:= 2*Modelica.Math.sin(x)-x^2/10;
 +
 +
end FungsiObjek;
 +
 +
Fungsi tersebut akan dipanggil di suatu file model
 +
 +
'''BracketOptimal.mo'''
 +
model BracketOptimal
 +
 +
parameter Integer n = 8;
 +
Real x1[n];
 +
Real x2[n];
 +
Real xup;
 +
Real xlow;
 +
Real f1[n];
 +
Real f2[n];
 +
Real xopt;
 +
Real yopt;
 +
Real d;
 +
 +
algorithm
 +
xup := 4;
 +
xlow := 0;
 +
 +
for i in 1:n loop
 +
  d:=((5^(1/2)-1)/2) * (xup-xlow);
 +
  x1[i] := xlow+d;
 +
  x2[i] := xup-d;
 +
  f1[i] := FungsiObjek(x1[i]);
 +
  f2[i] := FungsiObjek(x2[i]);
 +
 
 +
  if f1[i]>f2[i] then
 +
    xup := xup;
 +
    xlow := x2[i];
 +
    xopt := xup;
 +
    yopt := f1[i];
 +
    else
 +
      xlow :=xlow;
 +
      xup := x1[i];
 +
      xopt := xup;
 +
  end if;
 +
end for;
 +
 
 +
 +
end BracketOptimal;
 +
 +
== '''Pertemuan 6 (23 Desember 2020)''' ==
 +
 +
=== '''Tugas Besar''' ===
 +
 +
Kami diberi tugas besar yaitu melakukan oprimasi rangka sederhana, dengan tujuan mendesain rangka yang ''reliable'' dengan biaya yang optimal. Geometri dan ''load'' sudah diberikan Pak Dai di grup whatsapp. Tugas besar ini harus mempertimbangkan tiga variabel bebas yaitu, biaya, material, ''area cross section truss''. Kemudian juga membuat kurva efisiensi biaya dengan ''curve fitting''. Berikut bahan permodelannya :
 +
[[File:soaltubesumar.png|600px|thumb|center]]
 +
 +
'''Pendefinisian Masalah'''
 +
 +
Pertama - tama, Saya mendefinisikan node dan elemen pada struktur batang di soal.
 +
[[File:strukturbatangumar.png|600px|thumb|center]]
 +
 +
'''Asumsi dan Batasan'''
 +
 +
Asumsi :
 +
*Strukturnya sebagai truss, sehingga tidak ada bending.
 +
*Beban terdistribusi pada node-node yang ada.
 +
*Batas displacement 0,001m sebelum terjadi buckling.
 +
 +
Batasan :
 +
*Node 1, 2, 3, dan 4 merupakan fixed.
 +
*Beban F1 dan F2 terdistribusi ke node sekitarnya.
 +
*Beban eksternal pada node 14 dan 15 sebesar 500N arah y negatif.
 +
*Beban eksternal pada node 13 dan 16 sebesar 1000N arah y negatif.
 +
 +
'''Penentuan Penyelesaian yang akan dikerjakan'''
 +
 +
Data yang perlu dicari adalah variasi profil dari besi siku dengan suatu material dan variasi material dari besi siku dengan luas profil tertentu beserta dengan biayanya, karena yang ingin dicari adalah variasi material (elastisitas) dan luas profil dari besi siku yang optimum. Setelah mendapat seluruh data yang diperlukan, disusun dalam bentuk excel untuk proses perhitungan untuk sistem permodelan dan untuk 3D-trusses digunakan coding-an yang sudah dibuat oleh saudara Josiah, Christopher, dan Fahmi. Hasilnya berupa stress pada tiap truss yang terjadi pada elastisitas dan luas profil area tertentu. Kemudian untuk memenuhi data yang kurang digunakan permodelan Curve fitting untuk digunakan pada perhitungan dan komputasi nanti.
 +
 +
'''Menentukan variasi material dan variasi luas penampang untuk menghitung optimasi'''
 +
 +
Material tetap, variasi luas
 +
[[File:dataumar1.png|600px|thumb|center]]
 +
[[File:dataumar2.png|600px|thumb|center]]
 +
 +
Luas penampang tetap, variasi material
 +
[[File:dataumar3.png|600px|thumb|center]]
 +
 +
 +
'''Membuat Codingan untuk Mencari nilai displacement, reaction force, dan melakukan optimasi
 +
----
 +
 +
Berikut adalah coding hasil diskusi dua kelas yang dipimpin oleh Josiah, Fahmi, dan Christo
 +
 +
*Untuk perhitungan displacement, reaction force, stress, dan safety factor:
 +
model TugasBesar_Umar_Metnum02
 +
 +
//define initial variable
 +
parameter Integer Points=size(P,1); //Number of Points
 +
parameter Integer Trusses=size(C,1); //Number of Trusses
 +
parameter Real Yield=215e6; //Yield Strength (Pa)
 +
parameter Real Area=0.000224;  //Area L Profile (Dimension=0.03, Thickness=0,004) (m2)
 +
parameter Real Elas=193e9;    //Elasticity SS 304  (Pa)
 +
 +
//define connection
 +
parameter Integer C[:,2]=[1,5;
 +
                          2,6;
 +
                          3,7;
 +
                          4,8;
 +
                          5,6;  //1st floor
 +
                          6,7;  //1st floor
 +
                          7,8;  //1st floor
 +
                          5,8;  //1st floor
 +
                          5,9;
 +
                          6,10;
 +
                          7,11;
 +
                          8,12;
 +
                          9,10; //2nd floor
 +
                          10,11;//2nd floor
 +
                          11,12;//2nd floor
 +
                          9,12; //2nd floor
 +
                          9,13;
 +
                          10,14;
 +
                          11,15;
 +
                          12,16;
 +
                          13,14;//3rd floor
 +
                          14,15;//3rd floor
 +
                          15,16;//3rd floor
 +
                        13,16];//3rd floor
 +
                                                             
 +
//define coordinates (please put orderly)
 +
parameter Real P[:,6]=[0.3,-0.375,0,1,1,1;    //1
 +
                        -0.3,-0.375,0,1,1,1;    //2
 +
                        -0.3,0.375,0,1,1,1;    //3
 +
                        0.3,0.375,0,1,1,1;      //4
 +
                           
 +
                        0.3,-0.375,0.6,0,0,0;  //5
 +
                        -0.3,-0.375,0.6,0,0,0;  //6
 +
                        -0.3,0.375,0.6,0,0,0;  //7
 +
                        0.3,0.375,0.6,0,0,0;    //8
 +
                           
 +
                        0.3,-0.375,1.2,0,0,0;  //9
 +
                        -0.3,-0.375,1.2,0,0,0;  //10 
 +
                        -0.3,0.375,1.2,0,0,0;  //11
 +
                        0.3,0.375,1.2,0,0,0;    //12
 +
                           
 +
                        0.3,-0.375,1.8,0,0,0;  //13
 +
                        -0.3,-0.375,1.8,0,0,0;  //14
 +
                        -0.3,0.375,1.8,0,0,0;  //15
 +
                        0.3,0.375,1.8,0,0,0];  //16
 +
                         
 +
//define external force (please put orderly)
 +
parameter Real F[Points*3]={0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,0,
 +
                            0,0,-500,
 +
                            0,0,-1000,
 +
                            0,0,-1000,
 +
                            0,0,-500};
 +
 +
//solution
 +
Real displacement[N], reaction[N];
 +
Real check[3];
 +
 +
Real stress1[Trusses];
 +
Real safety[Trusses];
 +
Real dis[3];
 +
Real Str[3];
 +
 
 +
protected
 +
parameter Integer N=3*Points;
 +
Real q1[3], q2[3], g[N,N], G[N,N], G_star[N,N], id[N,N]=identity(N), cx, cy, cz, L, X[3,3];
 +
Real err=10e-10, ers=10e-4;
 +
 +
algorithm
 +
//Creating Global Matrix
 +
G:=id;
 +
for i in 1:Trusses loop
 +
  for j in 1:3 loop
 +
  q1[j]:=P[C[i,1],j];
 +
  q2[j]:=P[C[i,2],j];
 +
  end for;
 +
     
 +
    //Solving Matrix
 +
    L:=Modelica.Math.Vectors.length(q2-q1);
 +
    cx:=(q2[1]-q1[1])/L;
 +
    cy:=(q2[2]-q1[2])/L;
 +
    cz:=(q2[3]-q1[3])/L;
 +
    X:=(Area*Elas/L)*[cx^2,cx*cy,cx*cz;
 +
                      cy*cx,cy^2,cy*cz;
 +
                      cz*cx,cz*cy,cz^2];
 +
 +
    //Transforming to global matrix
 +
    g:=zeros(N,N);
 +
    for m,n in 1:3 loop
 +
      g[3*(C[i,1]-1)+m,3*(C[i,1]-1)+n]:=X[m,n];
 +
      g[3*(C[i,2]-1)+m,3*(C[i,2]-1)+n]:=X[m,n];
 +
      g[3*(C[i,2]-1)+m,3*(C[i,1]-1)+n]:=-X[m,n];
 +
      g[3*(C[i,1]-1)+m,3*(C[i,2]-1)+n]:=-X[m,n];
 +
    end for; 
 +
 +
  G_star:=G+g;
 +
  G:=G_star;
 +
end for;
 +
 +
//Implementing boundary
 +
for x in 1:Points loop
 +
  if P[x,4] <> 0 then
 +
    for a in 1:Points*3 loop
 +
      G[(x*3)-2,a]:=0;
 +
      G[(x*3)-2,(x*3)-2]:=1;
 +
    end for;
 +
  end if;
 +
  if P[x,5] <> 0 then
 +
    for a in 1:Points*3 loop
 +
      G[(x*3)-1,a]:=0;
 +
      G[(x*3)-1,(x*3)-1]:=1;
 +
    end for;
 +
  end if;
 +
  if P[x,6] <> 0 then
 +
    for a in 1:Points*3 loop
 +
      G[x*3,a]:=0;
 +
      G[x*3,x*3]:=1;
 +
    end for;
 +
  end if;
 +
end for;
 +
 +
//Solving displacement
 +
displacement:=Modelica.Math.Matrices.solve(G,F);
 +
 +
//Solving reaction
 +
reaction:=(G_star*displacement)-F;
 +
 +
//Eliminating float error
 +
for i in 1:N loop
 +
  reaction[i]:=if abs(reaction[i])<=err then 0 else reaction[i];
 +
  displacement[i]:=if abs(displacement[i])<=err then 0 else displacement[i];
 +
end for;
 +
 +
//Checking Force
 +
check[1]:=sum({reaction[i] for i in (1:3:(N-2))})+sum({F[i] for i in (1:3:(N-2))});
 +
check[2]:=sum({reaction[i] for i in (2:3:(N-1))})+sum({F[i] for i in (2:3:(N-1))});
 +
check[3]:=sum({reaction[i] for i in (3:3:N)})+sum({F[i] for i in (3:3:N)});
 +
 
 +
for i in 1:3 loop
 +
  check[i] := if abs(check[i])<=ers then 0 else check[i];
 +
end for;
 +
 +
//Calculating stress in each truss
 +
for i in 1:Trusses loop
 +
for j in 1:3 loop
 +
  q1[j]:=P[C[i,1],j];
 +
  q2[j]:=P[C[i,2],j];
 +
  dis[j]:=abs(displacement[3*(C[i,1]-1)+j]-displacement[3*(C[i,2]-1)+j]);
 +
end for;
 +
     
 +
    //Solving Matrix
 +
    L:=Modelica.Math.Vectors.length(q2-q1);
 +
    cx:=(q2[1]-q1[1])/L;
 +
    cy:=(q2[2]-q1[2])/L;
 +
    cz:=(q2[3]-q1[3])/L;
 +
    X:=(Elas/L)*[cx^2,cx*cy,cx*cz;
 +
                cy*cx,cy^2,cy*cz;
 +
                cz*cx,cz*cy,cz^2];
 +
   
 +
    Str:=(X*dis);
 +
    stress1[i]:=Modelica.Math.Vectors.length(Str);
 +
end for;
 +
 +
//Safety factor
 +
for i in 1:Trusses loop
 +
  if stress1[i]>0 then
 +
    safety[i]:=Yield/stress1[i];
 +
  else
 +
    safety[i]:=0;
 +
  end if;
 +
end for;
 +
 +
end TugasBesar_Umar_Metnum02;
 +
 +
 +
*Untuk membuat kurva curve fitting:
 +
function Curve_Fitting
 +
 +
input Real X[:];
 +
input Real Y[size(X,1)];
 +
input Integer order=2;
 +
output Real Coe[order+1];
 +
 +
protected
 +
Real Z[size(X,1),order+1];
 +
Real ZTr[order+1,size(X,1)];
 +
Real A[order+1,order+1];
 +
Real B[order+1];
 +
 +
algorithm
 +
 +
for i in 1:size(X,1) loop
 +
  for j in 1:(order+1) loop
 +
  Z[i,j]:=X[i]^(order+1-j);
 +
  end for;
 +
end for;
 +
ZTr:=transpose(Z);
 +
 +
A:=ZTr*Z;
 +
B:=ZTr*Y;
 +
Coe:=Modelica.Math.Matrices.solve(A,B);
 +
 +
end Curve_Fitting;
 +
 +
 +
* Untuk golden section:
 +
model Opt_Gold
 +
 +
parameter Real xd[:];
 +
parameter Real yd[size(xd,1)];
 +
parameter Real xlo=87e-6;
 +
parameter Real xhi=504e-6;
 +
parameter Integer N=10; // maximum iteration
 +
parameter Real es=0.0001; // maximum error
 +
 +
Real f1[N], f2[N], x1[N], x2[N], ea[N], y[3];
 +
Real xopt,  fx;
 +
protected
 +
Real d, xl, xu, xint, R=(5^(1/2)-1)/2;
 +
 +
algorithm
 +
xl := xlo;
 +
xu := xhi;
 +
y  := Curve_Fitting(xd,yd);
 +
 
 +
for i in 1:N loop
 +
  d:= R*(xu-xl);
 +
  x1[i]:=xl+d;
 +
  x2[i]:=xu-d;
 +
  f1[i]:=y[1]*x1[i]^2+y[2]*x1[i]+y[3];
 +
  f2[i]:=y[1]*x2[i]^2+y[2]*x2[i]+y[3];
 +
  xint:=xu-xl;
 +
 
 +
  if f1[i]>f2[i] then
 +
    xl:=x2[i];
 +
    xopt:=x1[i];
 +
    fx:=f1[i];
 +
    else
 +
      xu:=x1[i];
 +
      xopt:=x2[i];
 +
      fx:=f2[i];
 +
  end if;
 +
 
 +
  ea[i]:=(1-R)*abs((xint)/xopt);
 +
  if ea[i]<es then
 +
    break;
 +
  end if;
 +
end for;
 +
 +
end Opt_Gold;
 +
 +
 +
'''Hasil Perhitungan'''
 +
 +
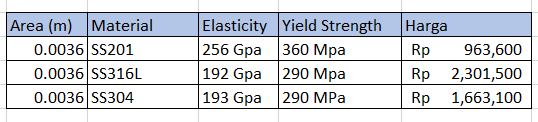
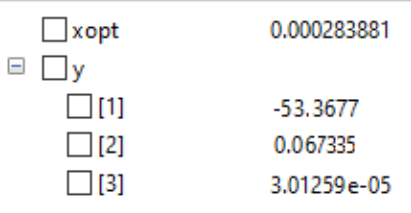
*Nilai luas area penampang optimum untuk material SS201 adalah 283,81 mm^2 atau untuk ukuran yang ada di pasaran ukuran optimumnya adalah batang L dengan lebar 6m dan tebal 60mm.
 +
 +
[[File:ItunganUmar1.png|600px|thumb|center]]
 +
 +
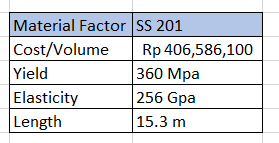
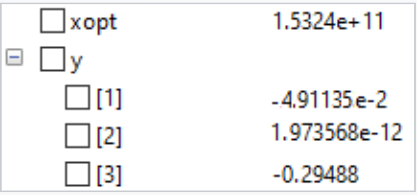
*Material optimum yang dapat digunakan untuk luas penampang 360mm^2 adalah material dengan nilai elastisitas 15324000000000 N/m^2 atau material yang paling mendekati adalah SS316L
 +
 +
[[File:ItunganUmar2.png|600px|thumb|center]]
 +
 +
 +
== '''Ujian Akhir Semester''' ==
 +
 +
'''Nomor 1'''
 +
[[File:nomer1umarr.jpg|600px|thumb|center]]
 +
'''Nomor 2 & 3'''
 +
[[File:nomer23umar.jpg|600px|thumb|center]]
 +
'''Nomor 4'''
 +
[[File:nomer4umar.jpg|600px|thumb|center]]
 +
'''Nomor 5 & 6'''
 +
[[File:nomer56umarr.jpg|600px|thumb|center]]
 +
 +
'''Berikut adalah hasil pengerjaan di OpenModelica untuk nomor 7'''
 +
 +
model UAS_Umar_Metnum02
 +
//define initial variable
 +
  parameter Integer Points = size(P, 1);
 +
    //Number of Points
 +
  parameter Integer Trusses = size(C, 1);
 +
    //Number of Trusses
 +
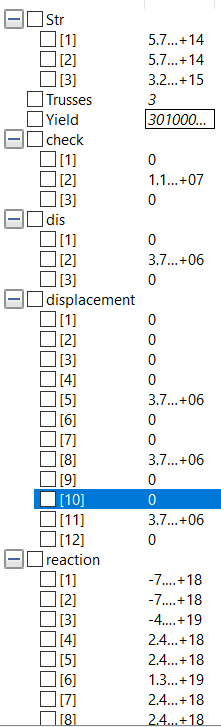
  parameter Real Yield = 301e6;
 +
    //Yield Strength (Pa)
 +
  parameter Real Area = 4256;
 +
    //Area L Profile
 +
  parameter Real Elas = 197e9;
 +
    //Elasticity Stainless Steel 201  (Pa)
 +
    //define connection
 +
  parameter Integer C[:, 2] = [1, 2; 1, 3; 1, 4];
 +
    //define coordinates (please put orderly)
 +
  parameter Real P[:, 6] = [0, 0, 0, 1, 1, 1;          //1
 +
                            6.5, 6.5, 36.5, 1, 1, 1;  //2
 +
                            6.5, 6.5, 36.5, 1, 1, 1;  //3
 +
                            6.5, 6.5, 36.5, 1, 1, 1];  //4
 +
    //define external force (please put orderly)
 +
  parameter Real F[Points * 3] = {0,0,0,0,3708180,0,0,3708180,0,0,3708180,0};
 +
    //solution
 +
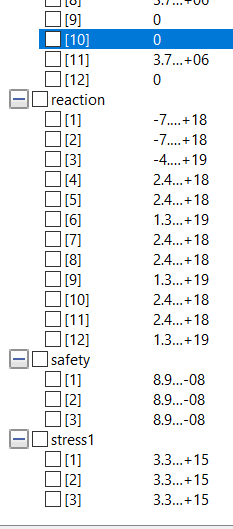
  Real displacement[N], reaction[N];
 +
  Real check[3];
 +
  Real stress1[Trusses];
 +
  Real safety[Trusses];
 +
  Real dis[3];
 +
  Real Str[3];
 +
protected
 +
  parameter Integer N = 3 * Points;
 +
  Real q1[3], q2[3], g[N, N], G[N, N], G_star[N, N], id[N, N] = identity(N), cx, cy, cz, L, X[3, 3];
 +
  Real err = 10e-10, ers = 10e-4;
 +
algorithm
 +
    //Creating Global Matrix
 +
  G := id;
 +
  for i in 1:Trusses loop
 +
    for j in 1:3 loop
 +
      q1[j] := P[C[i, 1], j];
 +
      q2[j] := P[C[i, 2], j];
 +
    end for;
 +
    L := Modelica.Math.Vectors.length(q2 - q1);
 +
    cx := (q2[1] - q1[1]) / L;
 +
    cy := (q2[2] - q1[2]) / L;
 +
    cz := (q2[3] - q1[3]) / L;
 +
    X := Area * Elas / L * [cx ^ 2, cx * cy, cx * cz; cy * cx, cy ^ 2, cy * cz; cz * cx, cz * cy, cz ^ 2];
 +
    g := zeros(N, N);
 +
    for m, n in 1:3 loop
 +
      g[3 * (C[i, 1] - 1) + m, 3 * (C[i, 1] - 1) + n] := X[m, n];
 +
      g[3 * (C[i, 2] - 1) + m, 3 * (C[i, 2] - 1) + n] := X[m, n];
 +
      g[3 * (C[i, 2] - 1) + m, 3 * (C[i, 1] - 1) + n] := -X[m, n];
 +
      g[3 * (C[i, 1] - 1) + m, 3 * (C[i, 2] - 1) + n] := -X[m, n];
 +
    end for;
 +
    G_star := G + g;
 +
    G := G_star;
 +
  end for;
 +
//Solving Matrix
 +
//Transforming to global matrix
 +
//Implementing boundary
 +
  for x in 1:Points loop
 +
    if P[x, 4] <> 0 then
 +
      for a in 1:Points * 3 loop
 +
        G[x * 3 - 2, a] := 0;
 +
        G[x * 3 - 2, x * 3 - 2] := 1;
 +
      end for;
 +
    end if;
 +
    if P[x, 5] <> 0 then
 +
      for a in 1:Points * 3 loop
 +
        G[x * 3 - 1, a] := 0;
 +
        G[x * 3 - 1, x * 3 - 1] := 1;
 +
      end for;
 +
    end if;
 +
    if P[x, 6] <> 0 then
 +
      for a in 1:Points * 3 loop
 +
        G[x * 3, a] := 0;
 +
        G[x * 3, x * 3] := 1;
 +
      end for;
 +
    end if;
 +
  end for;
 +
//Solving displacement
 +
  displacement := Modelica.Math.Matrices.solve(G, F);
 +
//Solving reaction
 +
  reaction := G_star * displacement - F;
 +
//Eliminating float error
 +
  for i in 1:N loop
 +
    reaction[i] := if abs(reaction[i]) <= err then 0 else reaction[i];
 +
    displacement[i] := if abs(displacement[i]) <= err then 0 else displacement[i];
 +
  end for;
 +
//Checking Force
 +
  check[1] := sum({reaction[i] for i in 1:3:N - 2}) + sum({F[i] for i in 1:3:N - 2});
 +
  check[2] := sum({reaction[i] for i in 2:3:N - 1}) + sum({F[i] for i in 2:3:N - 1});
 +
  check[3] := sum({reaction[i] for i in 3:3:N}) + sum({F[i] for i in 3:3:N});
 +
  for i in 1:3 loop
 +
    check[i] := if abs(check[i]) <= ers then 0 else check[i];
 +
  end for;
 +
//Calculating stress in each truss
 +
  for i in 1:Trusses loop
 +
    for j in 1:3 loop
 +
      q1[j] := P[C[i, 1], j];
 +
      q2[j] := P[C[i, 2], j];
 +
      dis[j] := abs(displacement[3 * (C[i, 1] - 1) + j] - displacement[3 * (C[i, 2] - 1) + j]);
 +
    end for;
 +
    L := Modelica.Math.Vectors.length(q2 - q1);
 +
    cx := (q2[1] - q1[1]) / L;
 +
    cy := (q2[2] - q1[2]) / L;
 +
    cz := (q2[3] - q1[3]) / L;
 +
    X := Elas / L * [cx ^ 2, cx * cy, cx * cz; cy * cx, cy ^ 2, cy * cz; cz * cx, cz * cy, cz ^ 2];
 +
    Str := X * dis;
 +
    stress1[i] := Modelica.Math.Vectors.length(Str);
 +
  end for;
 +
//Solving Matrix
 +
//Safety factor
 +
  for i in 1:Trusses loop
 +
    if stress1[i] > 0 then
 +
      safety[i] := Yield / stress1[i];
 +
    else
 +
      safety[i] := 0;
 +
    end if;
 +
  end for;
 +
 +
end UAS_Umar_Metnum02;
 +
 +
[[File:ssmodelicaumar1.png|600px|thumb|center]]
 +
[[File:ssmodelicaumar2.png|600px|thumb|center]]
 +
[[File:ssmodelicaumar3.png|600px|thumb|center]]

Latest revision as of 03:40, 14 January 2021

بِسْمِ اللهِ الرَّحْمَنِ الرَّحِيْمِ

السَّلاَمُ عَلَيْكُمْ وَرَحْمَةُ اللهِ وَبَرَكَاتُ

Biodata Diri

Umar.jpg

Nama  : Umar

NPM  : 1806200886

TTL  : Jakarta, 16 September 2000

Tempat Tinggal: Jakarta

Saya adalah Mahasiswa Fakultas Teknik Universitas Indonesia, Program Studi Teknik Mesin angkatan 2018. Saat ini Saya sedang menempuh perkuliahan di semester 5, yang artinya sudah tahun ketiga pekuliahan.

Alasan Saya mengambil Program Studi (Prodi) Teknik Mesin karena Prodi ini cukup luas prospeknya dan juga prodi yang mempelajari ilmu engineering yang lebih advance dari prodi teknik lainnya menurut Saya dan akan terus digunakan di masa mendatang.


Pertemuan 1 (11 November 2020)

Setelah melewati pembelajaran selama paruh semester awal dengan Bapak Dr. Ir. Engkos A. Kosasih, M.T. dan melakukan Ujian Tengah Semester (UTS), kelas Metode Numerik - 02 berganti dosen menjadi Bapak Dr. Ir. Ahmad Indra Siswantara atau biasa disapa Pak Da'i. Pada pertemuan pertama setelah UTS ini, Pak Da'i meminta mahasiswa untuk membuat akun pada situs wiki air. Kami dijelaskan bagaimana cara - cara untuk menggunakan wiki air ini supaya lebih familiar dalam menggunakannya, karena tugas - tugas kedepannya akan dikerjakan di wiki air. Pak Da'i pada saat itu juga memaparkan kembali apa tujuan dari pembelajaran metode numerik ini, berikut tujuan - tujuan yang beliau sebutkan :

  • Memahami konsep dan prinsip dasar dalam Metode Numerik
  • Mampu mengaplikasikan pemahaman dari konsep yang ada di dalam permodelan numerik
  • Mampu mengaplikasikan metode numerik dalam persoalan teknik
  • Mendapat nilai tambah/moral sehingga kita menjadi orang yang lebih beradab seperti yang sudah tertulis di sila ke-2 Pancasila.

Uniknya pada perkuliahan kemarin Saya mendapat suatu pelajaran hidup bahwa kita sebagai manusia punya limitasi terkait ilmu pengetahuan atau apapun itu, salah satu kasusnya dalam bidang matematika yaitu belum terpecahkan jawaban atau belum terdefinisi jawaban dari bilangan berapapun dibagi dengan 0 (n/0) walaupun menggunakan software sekalipun. Maka dari itu kita bisa petik hikmah bahwa sebagai manusia tidak boleh merasa "tinggi" walaupun orang - orang menganggap kita sebagai orang hebat sekalipun.

Tugas Pertemuan 1

Pada minggu pertama, setiap mahasiswa diminta untuk melihat dan mempraktikan tentang tutorial atau dasar Open Modellica dari youtube. Kemudian diharapkan untuk mengupload video tutorial singkat di Youtube. Berikut ini adalah video tutorial dasar dari saya untuk penghitungan volume langkah piston pada OpenModelica.

Pertemuan 2 (Rabu, 18 November 2020)

Pak Dai menjelaskan bahwa menggunakan Software OpenModelica lebih nyaman dan sederhana dibanding Software atau program yang sejenis lainnya. Sangat cocok untuk digunakan oleh para Engineer, karena dapat menyelesaikan persoalan perhitungan terkait masalah - masalah keteknikan atau permodelan desain (seperti : perpindahan kalor, sistem fluida, pengendalian sistem, dan rangkaian elektronika). Selain itu OpenModelica juga tidak rumit untuk seorang pemula untuk mempelajarinya. Kemudian Open Modelica tidak mempunyai bahasa program sendiri, itulah yang menyebabkan waktu simulasi permodelannya cukup lama. Open modelica memproses data lebih lama dikarenakan Open Modelica harus menerjemahkan perintah yang ditulis ke bahasa C++, setelah diproses baru akan muncul data - data perhitungan yang kita inginkan.


Tugas Pertemuan 2

Berikut tugas kedua yaitu pengaplikasian OpenModelica dengan class dan function dalam permasalahan matriks variabel array


Pertemuan 3 (25 November 2020)

Pada awal-awal Pak Dai memaparkan tiga aplikasi metode numerik yang sering digunakan dalam menyelesaikan permasalahan teknik, pertama ada Computation Fluid Dynamics (CFD), lalu FInite Element Analysis (FEA), dan Metode Stokastik. CFD dan FEA berbasis ilmu fisika, kemudian metode stokastik berbasis data dan statistik. Ada lima langkah yang Pak Dai paparkan dalam mengaplikasikan metode numerik ke permasalahan teknik :

  • Riset masalah tekniknya terlebih dahulu
  • Menganalisis masalah (mendefinisikan variabel yang mau dicari dan mencari parameter fisikanya)
  • Membuat model matematika
  • Membuat model numerik
  • Setelah itu cari penyelesaian dengan bantuan komputer untuk mendapatkan output yang diinginkan

Agar Kami bisa lebih paham tentang dasar-dasar metode numerik, Pak Dai menyuruh Kami untuk mencoba membuat fungsi untuk menyelesaikan Persamaan 9.12 di buku Numerical Methods for Engineers 7th Edition oleh Chapra dengan cara apapun (misalnya eliminasi gauss). Kedua, Kami disuru latihan menyelesaikan sistem persamaan dengan membuat fungsi penyelesaian dengan cara pseudocode 9.4 untuk menjawab soal 9.5 yang ada di buku yang sama juga. Latihan yang kedua ini dimaksudkan agar Kami paham dalam penggunaan array dalam penggunaan OpenModelica, yang dimana array ini dapat memudahkan mengumpulkan himpunan penyelesaian. Brikut adalah fungsi Gauss Jordan di OpenModelica 


function GaussJordan

input Real [:,:] A;
output Real [:,:] B;

protected // untuk local variable
Integer h = 1;    //pivot row
Integer k = 1;    //pivot coloumn
Integer m = size(A,1); //Number of row
Integer n = size(A,2); //Number of column
Integer c = 0;
Integer max_row; // Row index of max number in pivot column

Real [:] pivot_column;
Real [:] pivot_row;
Real [:,:] temp_array;
Real r;

Real float_error = 10e-10;


algorithm

//fungsi input A dan output B 
B := A;
  
while h <= m and k <= n loop

  for i in 1 : m loop
    for j in 1 : n loop
     if abs(B[i,j]) <= float_error then
       B[i,j] := 0;
      end if;
    end for;
  end for;

//Finding pivot 
  pivot_column:= {B[i,h] for i in h:m};
  
    //Mencari baris terbawah yang mempunyai nilai pivot tertinggi
    c:=h-1;
    for element in pivot_column loop
      c:= c+1;
      if abs(element)== max(abs(pivot_column)) then
        max_row :=c;
      end if;
    end for;
    
  //Jika tidak ada pivot di kolom ini, pindah ke kolom selanjutnya
  if B[max_row,k] == 0 then
    k:=k+1;
   
  else 
    // tukar row h - max_row
    temp_array := B;
    temp_array[h] := B[max_row];
    temp_array[max_row] := B[h];
    B:= temp_array;
    
    //devide pivot row by pivot number
     B[h] := B[h]/B[h,k];
     
     for i in (h+1) :m loop
       r := B[i,k]/B[h,k];
      
      B[i,k]:=0;
      
      for j in (k+1) : n loop
        B[i,j] := B[i,j]-B[h,j] * r;
      end for;
    end for;
    
    //move ke pivot kolom dan row selanjutnya
    h := h+1;
    k := k+1;
    
  end if;
  
end while;

// proses dari kanan atas
h :=m;
k :=n;

while h >=1 and k>=1 loop
  
  //dealing with error
  for i in 1:m loop
    for j in 1:n loop
      if abs(B[i,j]) <=float_error then
        B[i,j]:=0;
      end if;
    end for;
  end for; 

//finding pivot 
    pivot_row := {B[h,i] for i in 1:k};
    
    //Get position index k of pivot 
    c := 0;
    for element in pivot_row loop
      c := c+1;
      if element <> 0 then
        break;
      end if;
    end for;
    k:= c;
    
  // no pivot in this row, move to next row
  if B[h,k] == 0 then 
    h:= h-1;
    
  else
    //perform row operatation
    for i in 1:(h-1) loop
      r := B[i,k];
      B[i] := B[i] - B[h] *r;
    end for;
    
    //move to next pivot row dan column
    h:=h+1;
    k:=k+1;
    
  end if;
  
end while;
    
     
end GaussJordan;


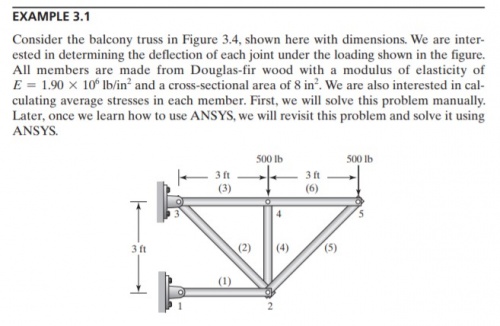
Contoh Soal Truss

Example 3.1 RS.jpg
Grafik Displacement
Grafik Reaction Forces

Kodingan 

model Trusses

parameter Integer N=10; //Global matrice = 2*points connected
parameter Real A=8;
parameter Real E=1.9e6;
Real G[N,N]; //global
Real Ginitial[N,N]; //global
Real Sol[N]; //global dispplacement
Real X[N]={0,0,0,0,0,0,0,-500,0,-500};
Real R[N]; //global reaction force
Real SolMat[N,1];
Real XMat[N,1];

//boundary coundition
Integer b1=1;
Integer b2=3;

//truss 1
parameter Real X1=0; //degree between truss
Real k1=A*E/36;
Real K1[4,4]; //stiffness matrice
Integer p1a=1;
Integer p1b=2;
Real G1[N,N];

//truss 2
parameter Real X2=135; //degree between truss
Real k2=A*E/50.912;
Real K2[4,4]; //stiffness matrice
Integer p2a=2;
Integer p2b=3;
Real G2[N,N];

//truss 3
parameter Real X3=0; //degree between truss
Real k3=A*E/36;
Real K3[4,4]; //stiffness matrice
Integer p3a=3;
Integer p3b=4;
Real G3[N,N];

//truss 4
parameter Real X4=90; //degree between truss
Real k4=A*E/36;
Real K4[4,4]; //stiffness matrice
Integer p4a=2;
Integer p4b=4;
Real G4[N,N];

//truss 5
parameter Real X5=45; //degree between truss
Real k5=A*E/50.912;
Real K5[4,4]; //stiffness matrice
Integer p5a=2;
Integer p5b=5;
Real G5[N,N];

//truss 6
parameter Real X6=0; //degree between truss
Real k6=A*E/36;
Real K6[4,4]; //stiffness matrice
Integer p6a=4;
Integer p6b=5;
Real G6[N,N];

/*
for each truss, please ensure pXa is lower then pXb (X represents truss element number)
*/

algorithm

//creating global matrice
K1:=Stiffness_Matrices(X1);
G1:=k1*Local_Global(K1,N,p1a,p1b);

K2:=Stiffness_Matrices(X2);
G2:=k2*Local_Global(K2,N,p2a,p2b);

K3:=Stiffness_Matrices(X3);
G3:=k3*Local_Global(K3,N,p3a,p3b);

K4:=Stiffness_Matrices(X4);
G4:=k4*Local_Global(K4,N,p4a,p4b);

K5:=Stiffness_Matrices(X5);
G5:=k5*Local_Global(K5,N,p5a,p5b);

K6:=Stiffness_Matrices(X6);
G6:=k6*Local_Global(K6,N,p6a,p6b);

G:=G1+G2+G3+G4+G5+G6;
Ginitial:=G;

//implementing boundary condition
for i in 1:N loop
 G[2*b1-1,i]:=0;
 G[2*b1,i]:=0;
 G[2*b2-1,i]:=0;
 G[2*b2,i]:=0;
end for;

G[2*b1-1,2*b1-1]:=1;
G[2*b1,2*b1]:=1;
G[2*b2-1,2*b2-1]:=1;
G[2*b2,2*b2]:=1;

//solving displacement
Sol:=Gauss_Jordan(N,G,X);

//solving reaction force
SolMat:=matrix(Sol);
XMat:=matrix(X);
R:=Reaction_Trusses(N,Ginitial,SolMat,XMat);

end Trusses;

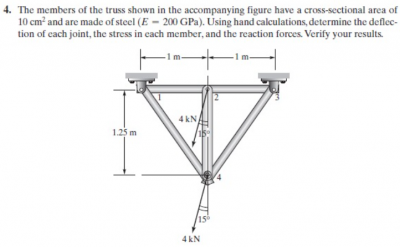
Tugas Pertemuan 3

Kami diberi tugas oleh Pak Dai untuk membuat program sederhana atau penyelesaian dari soal statika struktur dibawah ini dengan OpenModelica

Soal statur.png

Kodingan

Grafik Displacement
Grafik Reaction Forces

Persamaan 

class Trusses_HW

parameter Integer N=8; //Global matrice = 2*points connected
parameter Real A=0.001; //Area m2
parameter Real E=200e9; //Pa
Real G[N,N]; //global
Real Ginitial[N,N]; //global
Real Sol[N]; //global dispplacement
Real X[N]={0,0,-1035.2762,-3863.7033,0,0,-1035.2762,-3863.7033};
Real R[N]; //global reaction force
Real SolMat[N,1];
Real XMat[N,1];

//boundary condition
Integer b1=1;
Integer b2=3;

//truss 1
parameter Real X1=0; //degree between truss
Real k1=A*E/1;
Real K1[4,4]; //stiffness matrice
Integer p1a=1;
Integer p1b=2;
Real G1[N,N];

//truss 2
parameter Real X2=0; //degree between truss
Real k2=A*E/1;
Real K2[4,4]; //stiffness matrice
Integer p2a=2;
Integer p2b=3;
Real G2[N,N];

//truss 3
parameter Real X3=90; //degree between truss
Real k3=A*E/1.25;
Real K3[4,4]; //stiffness matrice
Integer p3a=2;
Integer p3b=4;
Real G3[N,N];

//truss 4
parameter Real X4=90+38.6598; //degree between truss
Real k4=A*E/1.6;
Real K4[4,4]; //stiffness matrice
Integer p4a=1;
Integer p4b=4;
Real G4[N,N];

//truss 5
parameter Real X5=90-38.6598; //degree between truss
Real k5=A*E/1.6;
Real K5[4,4]; //stiffness matrice
Integer p5a=3;
Integer p5b=4;
Real G5[N,N];

/*
for each truss, please ensure pXa is lower then pXb (X represents truss element number)
*/

algorithm

//creating global matrice
K1:=Stiffness_Matrices(X1);
G1:=k1*Local_Global(K1,N,p1a,p1b);

K2:=Stiffness_Matrices(X2);
G2:=k2*Local_Global(K2,N,p2a,p2b);

K3:=Stiffness_Matrices(X3);
G3:=k3*Local_Global(K3,N,p3a,p3b);

K4:=Stiffness_Matrices(X4);
G4:=k4*Local_Global(K4,N,p4a,p4b);

K5:=Stiffness_Matrices(X5);
G5:=k5*Local_Global(K5,N,p5a,p5b);

G:=G1+G2+G3+G4+G5;
Ginitial:=G;

//implementing boundary condition
for i in 1:N loop
 G[2*b1-1,i]:=0;
 G[2*b1,i]:=0;
 G[2*b2-1,i]:=0;
 G[2*b2,i]:=0;
end for;

G[2*b1-1,2*b1-1]:=1;
G[2*b1,2*b1]:=1;
G[2*b2-1,2*b2-1]:=1;
G[2*b2,2*b2]:=1;

//solving displacement
Sol:=Gauss_Jordan(N,G,X);

//solving reaction force
SolMat:=matrix(Sol);
XMat:=matrix(X);
R:=Reaction_Trusses(N,Ginitial,SolMat,XMat);

end Trusses_HW;

Fungsi Panggil

Matrice Transformation 

function Stiffness_Matrices
input Real A;
Real Y;
output Real X[4,4];
Real float_error = 10e-10;

final constant Real pi=2*Modelica.Math.asin(1.0);

algorithm

Y:=A/180*pi;
    
X:=[(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y);

Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2;

-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y);

-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2];

for i in 1:4 loop
 for j in 1:4 loop
   if abs(X[i,j]) <= float_error then
     X[i,j] := 0;
   end if;
 end for;
end for;

end Stiffness_Matrices;

Global Element Matrice 

function Local_Global
input Real Y[4,4];
input Integer B;
input Integer p1;
input Integer p2;
output Real G[B,B];

algorithm

for i in 1:B loop
 for j in 1:B loop
     G[i,j]:=0;
 end for;
end for;

G[2*p1,2*p1]:=Y[2,2];
G[2*p1-1,2*p1-1]:=Y[1,1];
G[2*p1,2*p1-1]:=Y[2,1];
G[2*p1-1,2*p1]:=Y[1,2];

G[2*p2,2*p2]:=Y[4,4];
G[2*p2-1,2*p2-1]:=Y[3,3];
G[2*p2,2*p2-1]:=Y[4,3];
G[2*p2-1,2*p2]:=Y[3,4];

G[2*p2,2*p1]:=Y[4,2];
G[2*p2-1,2*p1-1]:=Y[3,1];
G[2*p2,2*p1-1]:=Y[4,1];
G[2*p2-1,2*p1]:=Y[3,2];

G[2*p1,2*p2]:=Y[2,4];
G[2*p1-1,2*p2-1]:=Y[1,3];
G[2*p1,2*p2-1]:=Y[2,3];
G[2*p1-1,2*p2]:=Y[1,4];

end Local_Global;

Reaction Matrice Equation 

function Reaction_Trusses
input Integer N;
input Real A[N,N];
input Real B[N,1];
input Real C[N,1];
Real X[N,1];
output Real Sol[N];
Real float_error = 10e-10;

algorithm
X:=A*B-C;

for i in 1:N loop
 if abs(X[i,1]) <= float_error then
   X[i,1] := 0;
 end if;
end for;

for i in 1:N loop
 Sol[i]:=X[i,1];
end for;

end Reaction_Trusses;


Pertemuan 4 (2 Desember 2020)

Kuis : Membuat class diagram dan flowchart setiap class

Perhitungan.jpg
Flowchartt.jpg
Supportingdiagramm.jpg

Tugas Pertemuan 4

Soal

Pr4metnum.png

Flow Chart

Flowcharttt.jpg

Perhitungan di OpenModelica

Berikut kodingannya : 

class Trusses3D_Tes

//define initial variable
parameter Integer Points=4; //Number of Points
parameter Integer Trusses=3; //Number of Trusses
parameter Real Area=0.0015; //Area
parameter Real Elas=70e9; //Elasticity

//define connection
parameter Integer C[Trusses,2]=[1,2;
                                1,3;
                                1,4];
                              
//define coordinates (please put orderly)
parameter Real P[Points,3]=[2,0,0;
                            0,0,1.5;
                            0,0,-1.5;
                            0,1.5,0]; 

//define external force (please put orderly)
parameter Real F[Points*3]={0,-5000,0,
                            0,0,0, 
                            0,0,0, 
                            0,0,0}; 

//define boundary
parameter Integer b[:]={2,3,4};

//solution
Real displacement[N], reaction[N];

protected
parameter Integer N=3*Points;
Integer boundary[3*size(b,1)]=cat(1,(3*b).-2,(3*b).-1,3*b);
Real q1[3], q2[3], g[N,N], G[N,N], G_star[N,N], id[N,N]=identity(N), err=10e-10, cx, cy, cz, L, X[3,3];

algorithm
//Creating Global Matrix
G:=id;
for i in 1:Trusses loop
 for j in 1:3 loop
   q1[j]:=P[C[i,1],j];
   q2[j]:=P[C[i,2],j];
 end for;

       //Solving Matrix
       L:=Modelica.Math.Vectors.length(q2-q1);
       cx:=(q2[1]-q1[1])/L;
       cy:=(q2[2]-q1[2])/L;
       cz:=(q2[3]-q1[3])/L; 
       X:=(Area*Elas/L)*[cx^2,cx*cy,cx*cz;
                         cy*cx,cy^2,cy*cz;
                         cz*cx,cz*cy,cz^2];

       //Transforming to global matrix
       g:=zeros(N,N); 
       for m,n in 1:3 loop
         g[3*(C[i,1]-1)+m,3*(C[i,1]-1)+n]:=X[m,n];
         g[3*(C[i,2]-1)+m,3*(C[i,2]-1)+n]:=X[m,n];
         g[3*(C[i,2]-1)+m,3*(C[i,1]-1)+n]:=-X[m,n];
         g[3*(C[i,1]-1)+m,3*(C[i,2]-1)+n]:=-X[m,n];
       end for;  
 
 G_star:=G+g;
 G:=G_star;
end for;

//Implementing boundary
for i in boundary loop
 for j in 1:N loop
   G[i,j]:=id[i,j];
 end for;
end for;

//Solving displacement
displacement:=Modelica.Math.Matrices.solve(G,F);

//Solving reaction
reaction:=(G_star*displacement)-F;

//Eliminating float error
for i in 1:N loop
 reaction[i]:=if abs(reaction[i])<=err then 0 else reaction[i];
 displacement[i]:=if abs(displacement[i])<=err then 0 else displacement[i];
end for;

end Trusses3D_Tes;
3D grf1.png
3D grf.png

Verifikasi Perhitungan

Verifikasi.jpg

Pertemuan 5 (16 Desember 2020)

Pada pertemuan kelima, Kami diajarkan mengenai pengaplikasian metode numerik dalam optimasi sebuah sistem menggunakan OpenModelica. Materi ini dijelaskan melalui sebuah video oleh Bu Candra serta pseudocode dari persoalan tersebut, contoh persoalan tersebut adalah optimasi menggunakan metode Bracket. Berikut adalah pseudocode yang di OpenModelica : 

FungsiObjek.mo
function FungsiObjek
 
input Real x;
output Real y;

algorithm

y:= 2*Modelica.Math.sin(x)-x^2/10;

end FungsiObjek;

Fungsi tersebut akan dipanggil di suatu file model 

BracketOptimal.mo
model BracketOptimal

parameter Integer n = 8;
Real x1[n];
Real x2[n];
Real xup;
Real xlow;
Real f1[n];
Real f2[n];
Real xopt;
Real yopt;
Real d;

algorithm
xup := 4;
xlow := 0;

for i in 1:n loop
  d:=((5^(1/2)-1)/2) * (xup-xlow);
  x1[i] := xlow+d;
  x2[i] := xup-d;
  f1[i] := FungsiObjek(x1[i]);
  f2[i] := FungsiObjek(x2[i]);
  
  if f1[i]>f2[i] then
    xup := xup;
    xlow := x2[i];
    xopt := xup;
    yopt := f1[i];
    else
      xlow :=xlow;
      xup := x1[i];
      xopt := xup;
  end if;
end for;
 

end BracketOptimal;

Pertemuan 6 (23 Desember 2020)

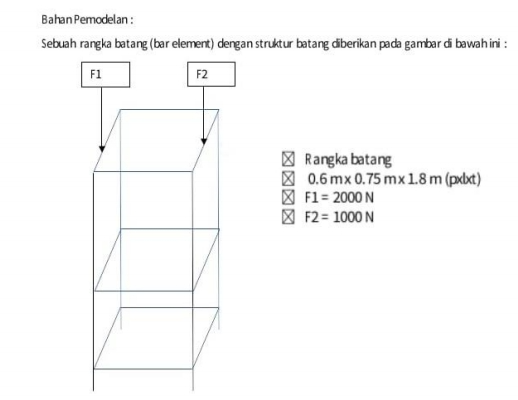
Tugas Besar

Kami diberi tugas besar yaitu melakukan oprimasi rangka sederhana, dengan tujuan mendesain rangka yang reliable dengan biaya yang optimal. Geometri dan load sudah diberikan Pak Dai di grup whatsapp. Tugas besar ini harus mempertimbangkan tiga variabel bebas yaitu, biaya, material, area cross section truss. Kemudian juga membuat kurva efisiensi biaya dengan curve fitting. Berikut bahan permodelannya :

Soaltubesumar.png

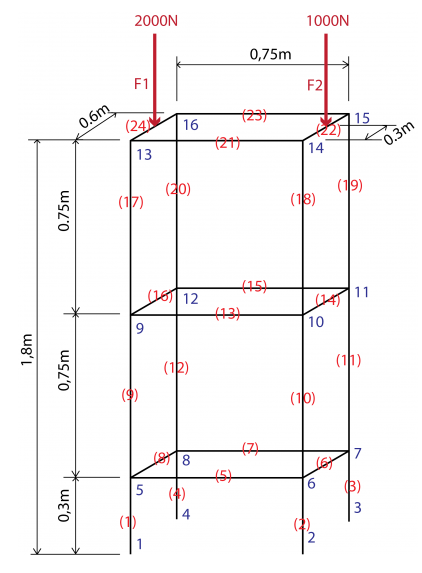
Pendefinisian Masalah

Pertama - tama, Saya mendefinisikan node dan elemen pada struktur batang di soal.

Strukturbatangumar.png

Asumsi dan Batasan

Asumsi :

  • Strukturnya sebagai truss, sehingga tidak ada bending.
  • Beban terdistribusi pada node-node yang ada.
  • Batas displacement 0,001m sebelum terjadi buckling.

Batasan :

  • Node 1, 2, 3, dan 4 merupakan fixed.
  • Beban F1 dan F2 terdistribusi ke node sekitarnya.
  • Beban eksternal pada node 14 dan 15 sebesar 500N arah y negatif.
  • Beban eksternal pada node 13 dan 16 sebesar 1000N arah y negatif.

Penentuan Penyelesaian yang akan dikerjakan

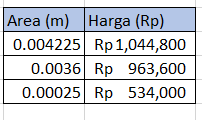
Data yang perlu dicari adalah variasi profil dari besi siku dengan suatu material dan variasi material dari besi siku dengan luas profil tertentu beserta dengan biayanya, karena yang ingin dicari adalah variasi material (elastisitas) dan luas profil dari besi siku yang optimum. Setelah mendapat seluruh data yang diperlukan, disusun dalam bentuk excel untuk proses perhitungan untuk sistem permodelan dan untuk 3D-trusses digunakan coding-an yang sudah dibuat oleh saudara Josiah, Christopher, dan Fahmi. Hasilnya berupa stress pada tiap truss yang terjadi pada elastisitas dan luas profil area tertentu. Kemudian untuk memenuhi data yang kurang digunakan permodelan Curve fitting untuk digunakan pada perhitungan dan komputasi nanti.

Menentukan variasi material dan variasi luas penampang untuk menghitung optimasi

Material tetap, variasi luas

Dataumar1.png
Dataumar2.png

Luas penampang tetap, variasi material

Dataumar3.png


Membuat Codingan untuk Mencari nilai displacement, reaction force, dan melakukan optimasi

Berikut adalah coding hasil diskusi dua kelas yang dipimpin oleh Josiah, Fahmi, dan Christo

  • Untuk perhitungan displacement, reaction force, stress, dan safety factor:
model TugasBesar_Umar_Metnum02

//define initial variable
parameter Integer Points=size(P,1); //Number of Points
parameter Integer Trusses=size(C,1); //Number of Trusses
parameter Real Yield=215e6; //Yield Strength (Pa)
parameter Real Area=0.000224;   //Area L Profile (Dimension=0.03, Thickness=0,004) (m2)
parameter Real Elas=193e9;     //Elasticity SS 304  (Pa)

//define connection
parameter Integer C[:,2]=[1,5; 
                          2,6;
                          3,7;
                          4,8;
                          5,6;  //1st floor
                          6,7;  //1st floor
                          7,8;  //1st floor
                          5,8;  //1st floor
                          5,9;
                         6,10;
                         7,11;
                         8,12;
                         9,10; //2nd floor
                         10,11;//2nd floor 
                         11,12;//2nd floor
                          9,12; //2nd floor
                          9,13;
                         10,14;
                         11,15;
                         12,16;
                         13,14;//3rd floor
                         14,15;//3rd floor
                         15,16;//3rd floor
                        13,16];//3rd floor
                                                              
//define coordinates (please put orderly)
parameter Real P[:,6]=[0.3,-0.375,0,1,1,1;     //1
                       -0.3,-0.375,0,1,1,1;    //2
                       -0.3,0.375,0,1,1,1;     //3
                       0.3,0.375,0,1,1,1;      //4
                           
                       0.3,-0.375,0.6,0,0,0;   //5
                       -0.3,-0.375,0.6,0,0,0;  //6
                       -0.3,0.375,0.6,0,0,0;   //7
                       0.3,0.375,0.6,0,0,0;    //8
                            
                       0.3,-0.375,1.2,0,0,0;   //9
                       -0.3,-0.375,1.2,0,0,0;  //10  
                       -0.3,0.375,1.2,0,0,0;   //11
                       0.3,0.375,1.2,0,0,0;    //12
                            
                       0.3,-0.375,1.8,0,0,0;   //13
                       -0.3,-0.375,1.8,0,0,0;  //14
                       -0.3,0.375,1.8,0,0,0;   //15
                       0.3,0.375,1.8,0,0,0];   //16
                          
//define external force (please put orderly)
parameter Real F[Points*3]={0,0,0,
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,0, 
                            0,0,-500, 
                            0,0,-1000, 
                            0,0,-1000, 
                            0,0,-500}; 

//solution
Real displacement[N], reaction[N];
Real check[3];

Real stress1[Trusses];
Real safety[Trusses];
Real dis[3];
Real Str[3];
 
protected
parameter Integer N=3*Points;
Real q1[3], q2[3], g[N,N], G[N,N], G_star[N,N], id[N,N]=identity(N), cx, cy, cz, L, X[3,3];
Real err=10e-10, ers=10e-4;

algorithm
//Creating Global Matrix
G:=id;
for i in 1:Trusses loop
 for j in 1:3 loop
  q1[j]:=P[C[i,1],j];
  q2[j]:=P[C[i,2],j];
 end for;
      
   //Solving Matrix
   L:=Modelica.Math.Vectors.length(q2-q1);
   cx:=(q2[1]-q1[1])/L;
   cy:=(q2[2]-q1[2])/L;
   cz:=(q2[3]-q1[3])/L; 
   X:=(Area*Elas/L)*[cx^2,cx*cy,cx*cz;
                     cy*cx,cy^2,cy*cz;
                     cz*cx,cz*cy,cz^2];

   //Transforming to global matrix
   g:=zeros(N,N); 
   for m,n in 1:3 loop
     g[3*(C[i,1]-1)+m,3*(C[i,1]-1)+n]:=X[m,n];
     g[3*(C[i,2]-1)+m,3*(C[i,2]-1)+n]:=X[m,n];
     g[3*(C[i,2]-1)+m,3*(C[i,1]-1)+n]:=-X[m,n];
     g[3*(C[i,1]-1)+m,3*(C[i,2]-1)+n]:=-X[m,n];
   end for;  

 G_star:=G+g;
 G:=G_star;
end for;

//Implementing boundary
for x in 1:Points loop
 if P[x,4] <> 0 then
   for a in 1:Points*3 loop
     G[(x*3)-2,a]:=0;
     G[(x*3)-2,(x*3)-2]:=1;
   end for;
 end if;
 if P[x,5] <> 0 then
   for a in 1:Points*3 loop
     G[(x*3)-1,a]:=0;
     G[(x*3)-1,(x*3)-1]:=1;
   end for;
 end if;
 if P[x,6] <> 0 then
   for a in 1:Points*3 loop
     G[x*3,a]:=0;
     G[x*3,x*3]:=1;
   end for;
 end if;
end for;

//Solving displacement
displacement:=Modelica.Math.Matrices.solve(G,F);

//Solving reaction
reaction:=(G_star*displacement)-F;

//Eliminating float error
for i in 1:N loop
 reaction[i]:=if abs(reaction[i])<=err then 0 else reaction[i];
 displacement[i]:=if abs(displacement[i])<=err then 0 else displacement[i];
end for;

//Checking Force
check[1]:=sum({reaction[i] for i in (1:3:(N-2))})+sum({F[i] for i in (1:3:(N-2))});
check[2]:=sum({reaction[i] for i in (2:3:(N-1))})+sum({F[i] for i in (2:3:(N-1))});
check[3]:=sum({reaction[i] for i in (3:3:N)})+sum({F[i] for i in (3:3:N)});
  
for i in 1:3 loop
 check[i] := if abs(check[i])<=ers then 0 else check[i];
end for;

//Calculating stress in each truss
for i in 1:Trusses loop
for j in 1:3 loop
  q1[j]:=P[C[i,1],j];
  q2[j]:=P[C[i,2],j];
  dis[j]:=abs(displacement[3*(C[i,1]-1)+j]-displacement[3*(C[i,2]-1)+j]);
end for;
      
   //Solving Matrix
   L:=Modelica.Math.Vectors.length(q2-q1);
   cx:=(q2[1]-q1[1])/L;
   cy:=(q2[2]-q1[2])/L;
   cz:=(q2[3]-q1[3])/L; 
   X:=(Elas/L)*[cx^2,cx*cy,cx*cz;
                cy*cx,cy^2,cy*cz;
                cz*cx,cz*cy,cz^2];
   
   Str:=(X*dis);
   stress1[i]:=Modelica.Math.Vectors.length(Str);
end for;

//Safety factor
for i in 1:Trusses loop
 if stress1[i]>0 then
   safety[i]:=Yield/stress1[i];
 else
   safety[i]:=0;
 end if; 
end for;

end TugasBesar_Umar_Metnum02;


  • Untuk membuat kurva curve fitting:
function Curve_Fitting

input Real X[:];
input Real Y[size(X,1)];
input Integer order=2;
output Real Coe[order+1];

protected
Real Z[size(X,1),order+1];
Real ZTr[order+1,size(X,1)];
Real A[order+1,order+1];
Real B[order+1];

algorithm

for i in 1:size(X,1) loop
 for j in 1:(order+1) loop
 Z[i,j]:=X[i]^(order+1-j);
 end for;
end for;
ZTr:=transpose(Z);

A:=ZTr*Z;
B:=ZTr*Y;
Coe:=Modelica.Math.Matrices.solve(A,B);

end Curve_Fitting;


  • Untuk golden section:
model Opt_Gold

parameter Real xd[:];
parameter Real yd[size(xd,1)];
parameter Real xlo=87e-6;
parameter Real xhi=504e-6; 
parameter Integer N=10; // maximum iteration
parameter Real es=0.0001; // maximum error

Real f1[N], f2[N], x1[N], x2[N], ea[N], y[3];
Real xopt,  fx;
protected
Real d, xl, xu, xint, R=(5^(1/2)-1)/2;

algorithm
xl := xlo; 
xu := xhi;
y  := Curve_Fitting(xd,yd);
 
for i in 1:N loop
 d:= R*(xu-xl);
 x1[i]:=xl+d;
 x2[i]:=xu-d;
 f1[i]:=y[1]*x1[i]^2+y[2]*x1[i]+y[3];
 f2[i]:=y[1]*x2[i]^2+y[2]*x2[i]+y[3];
 xint:=xu-xl;
 
 if f1[i]>f2[i] then
   xl:=x2[i];
   xopt:=x1[i];
   fx:=f1[i];
   else
     xu:=x1[i];
     xopt:=x2[i];
     fx:=f2[i];
 end if;
 
 ea[i]:=(1-R)*abs((xint)/xopt);
 if ea[i]<es then
   break;
 end if;
end for;

end Opt_Gold;


Hasil Perhitungan

  • Nilai luas area penampang optimum untuk material SS201 adalah 283,81 mm^2 atau untuk ukuran yang ada di pasaran ukuran optimumnya adalah batang L dengan lebar 6m dan tebal 60mm.
ItunganUmar1.png
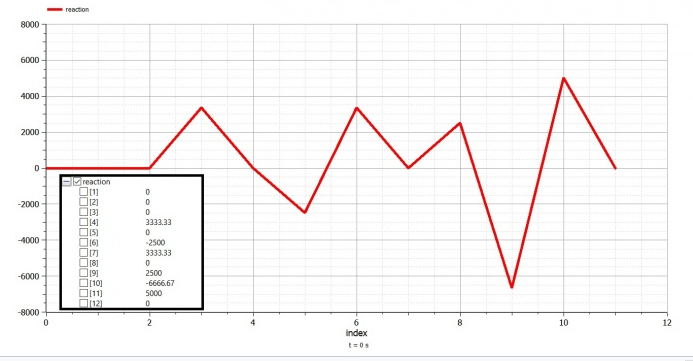
  • Material optimum yang dapat digunakan untuk luas penampang 360mm^2 adalah material dengan nilai elastisitas 15324000000000 N/m^2 atau material yang paling mendekati adalah SS316L
ItunganUmar2.png


Ujian Akhir Semester

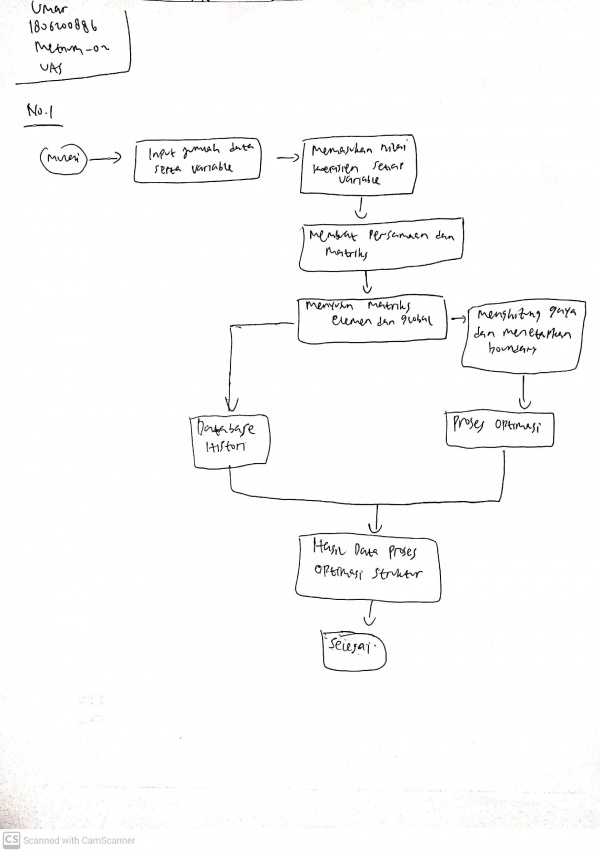
Nomor 1

Nomer1umarr.jpg

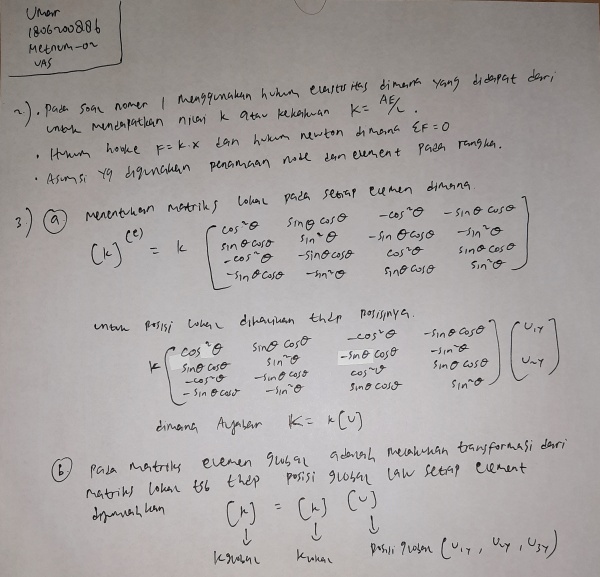
Nomor 2 & 3

Nomer23umar.jpg

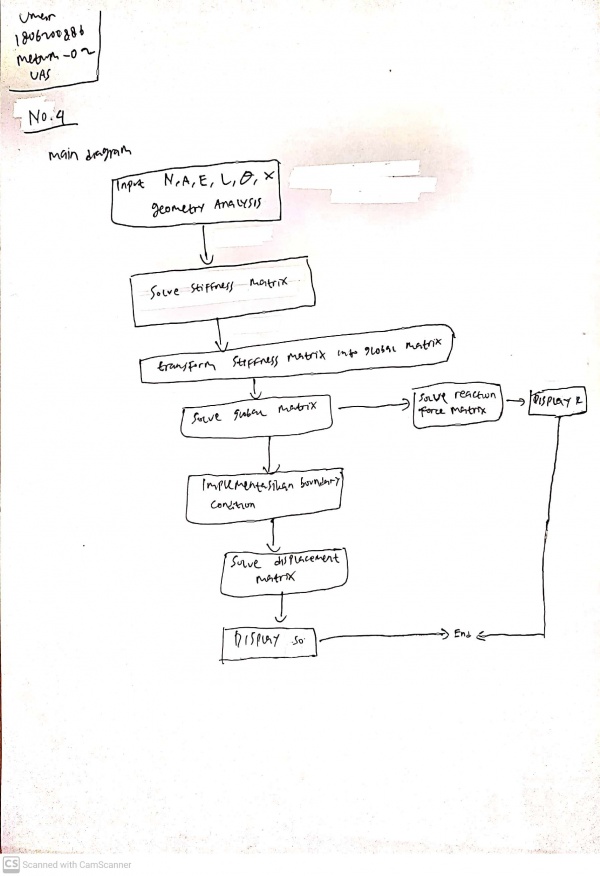
Nomor 4

Nomer4umar.jpg

Nomor 5 & 6

Nomer56umarr.jpg

Berikut adalah hasil pengerjaan di OpenModelica untuk nomor 7

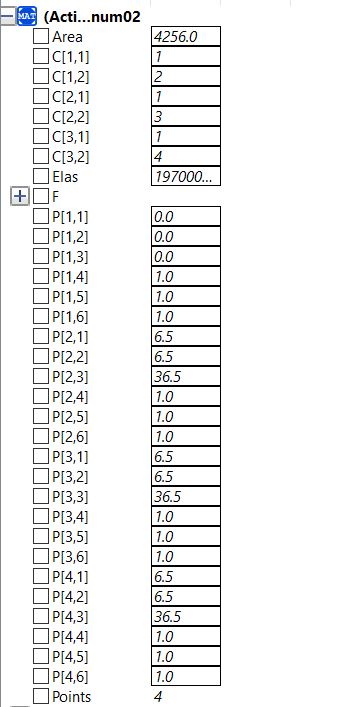
model UAS_Umar_Metnum02 

//define initial variable
 parameter Integer Points = size(P, 1);
   //Number of Points
 parameter Integer Trusses = size(C, 1);
   //Number of Trusses
 parameter Real Yield = 301e6;
   //Yield Strength (Pa)
 parameter Real Area = 4256;
   //Area L Profile
 parameter Real Elas = 197e9;
   //Elasticity Stainless Steel 201  (Pa)
   //define connection
 parameter Integer C[:, 2] = [1, 2; 1, 3; 1, 4];
   //define coordinates (please put orderly)
 parameter Real P[:, 6] = [0, 0, 0, 1, 1, 1;          //1
                           6.5, 6.5, 36.5, 1, 1, 1;   //2
                           6.5, 6.5, 36.5, 1, 1, 1;   //3
                           6.5, 6.5, 36.5, 1, 1, 1];  //4
   //define external force (please put orderly)
 parameter Real F[Points * 3] = {0,0,0,0,3708180,0,0,3708180,0,0,3708180,0};
   //solution
 Real displacement[N], reaction[N];
 Real check[3];
 Real stress1[Trusses];
 Real safety[Trusses];
 Real dis[3];
 Real Str[3];

protected 

 parameter Integer N = 3 * Points;
 Real q1[3], q2[3], g[N, N], G[N, N], G_star[N, N], id[N, N] = identity(N), cx, cy, cz, L, X[3, 3];
 Real err = 10e-10, ers = 10e-4;

algorithm 

   //Creating Global Matrix
 G := id;
 for i in 1:Trusses loop
   for j in 1:3 loop
     q1[j] := P[C[i, 1], j];
     q2[j] := P[C[i, 2], j];
   end for;
   L := Modelica.Math.Vectors.length(q2 - q1);
   cx := (q2[1] - q1[1]) / L;
   cy := (q2[2] - q1[2]) / L;
   cz := (q2[3] - q1[3]) / L;
   X := Area * Elas / L * [cx ^ 2, cx * cy, cx * cz; cy * cx, cy ^ 2, cy * cz; cz * cx, cz * cy, cz ^ 2];
   g := zeros(N, N);
   for m, n in 1:3 loop
     g[3 * (C[i, 1] - 1) + m, 3 * (C[i, 1] - 1) + n] := X[m, n];
     g[3 * (C[i, 2] - 1) + m, 3 * (C[i, 2] - 1) + n] := X[m, n];
     g[3 * (C[i, 2] - 1) + m, 3 * (C[i, 1] - 1) + n] := -X[m, n];
     g[3 * (C[i, 1] - 1) + m, 3 * (C[i, 2] - 1) + n] := -X[m, n];
   end for;
   G_star := G + g;
   G := G_star;
 end for;

//Solving Matrix //Transforming to global matrix //Implementing boundary 

 for x in 1:Points loop
   if P[x, 4] <> 0 then
     for a in 1:Points * 3 loop
       G[x * 3 - 2, a] := 0;
       G[x * 3 - 2, x * 3 - 2] := 1;
     end for;
   end if;
   if P[x, 5] <> 0 then
     for a in 1:Points * 3 loop
       G[x * 3 - 1, a] := 0;
       G[x * 3 - 1, x * 3 - 1] := 1;
     end for;
   end if;
   if P[x, 6] <> 0 then
     for a in 1:Points * 3 loop
       G[x * 3, a] := 0;
       G[x * 3, x * 3] := 1;
     end for;
   end if;
 end for;

//Solving displacement 

 displacement := Modelica.Math.Matrices.solve(G, F);

//Solving reaction 

 reaction := G_star * displacement - F;

//Eliminating float error 

 for i in 1:N loop
   reaction[i] := if abs(reaction[i]) <= err then 0 else reaction[i];
   displacement[i] := if abs(displacement[i]) <= err then 0 else displacement[i];
 end for;

//Checking Force 

 check[1] := sum({reaction[i] for i in 1:3:N - 2}) + sum({F[i] for i in 1:3:N - 2});
 check[2] := sum({reaction[i] for i in 2:3:N - 1}) + sum({F[i] for i in 2:3:N - 1});
 check[3] := sum({reaction[i] for i in 3:3:N}) + sum({F[i] for i in 3:3:N});
 for i in 1:3 loop
   check[i] := if abs(check[i]) <= ers then 0 else check[i];
 end for;

//Calculating stress in each truss 

 for i in 1:Trusses loop
   for j in 1:3 loop
     q1[j] := P[C[i, 1], j];
     q2[j] := P[C[i, 2], j];
     dis[j] := abs(displacement[3 * (C[i, 1] - 1) + j] - displacement[3 * (C[i, 2] - 1) + j]);
   end for;
   L := Modelica.Math.Vectors.length(q2 - q1);
   cx := (q2[1] - q1[1]) / L;
   cy := (q2[2] - q1[2]) / L;
   cz := (q2[3] - q1[3]) / L;
   X := Elas / L * [cx ^ 2, cx * cy, cx * cz; cy * cx, cy ^ 2, cy * cz; cz * cx, cz * cy, cz ^ 2];
   Str := X * dis;
   stress1[i] := Modelica.Math.Vectors.length(Str);
 end for;

//Solving Matrix //Safety factor 

 for i in 1:Trusses loop
   if stress1[i] > 0 then
     safety[i] := Yield / stress1[i];
   else
     safety[i] := 0;
   end if;
 end for;

end UAS_Umar_Metnum02;

Ssmodelicaumar1.png
Ssmodelicaumar2.png
Ssmodelicaumar3.png
Retrieved from "http://air.eng.ui.ac.id/index.php?title=Umar&oldid=55617"