Difference between revisions of "Muhammad Rayhan Faturrahman"
Mrayhanfatur (talk | contribs) (→BIODATA) |
Mrayhanfatur (talk | contribs) (→Jawaban UAS (13 Januari 2021)) |
||
| (60 intermediate revisions by the same user not shown) | |||
| Line 6: | Line 6: | ||
Jurusan : Teknik Mesin | Jurusan : Teknik Mesin | ||
| + | |||
| + | Angkatan : 2019 | ||
Hobi : Kulineran | Hobi : Kulineran | ||
| − | Selamat sore teman-teman, perkenalkan nama saya Fatur, | + | Jargon : Selaras Dalam Dinamika |
| + | |||
| + | |||
| + | |||
| + | Selamat sore teman-teman, perkenalkan nama saya Fatur, teman-teman saya di Departemen Teknik Mesin biasa memanggil saya bob. Saya memilih jurusan Teknik Mesin karena terisnpirasi dari saudara saya yang seorang process engineer, saya sering memerhatikannya melakukan simulasi desain pabrik dan menurut saya itu merupakan pekerjaan yang seru sekaligus menantang, berangkat dari sana saya memilih untuk menjadi seorang engineer juga tapi pada disiplin yang berbeda dengan harapan nantinya kami dapat membuat perusahaan konsultan sendiri. | ||
| + | |||
| + | == Komputasi == | ||
| + | Saya memiliki ketertarikan pada dunia komputasi sejak awal memasuki perkuliahan teknik, namun masih muncul banyak pertanyaan "harus mulai belajar dari mana?" dan pertanyaan lain sebagainya, alasan inilah yang semata-mata menjadi pemicu saya untuk mencoba mengambil mata kuliah metode numerik di semester 3 ini. | ||
| + | |||
| + | == Perkuliahan Minggu Pertama (Rabu, 11 November 2020) == | ||
| + | |||
| + | Pada pertemuan pertama yang diisi oleh Bapak Dr. Ir. Ahmad Indra Siswantara. Mahasiswa dijelaskan mengenai esensi atau tujuan pembelajaran metode numerik, yaitu: | ||
| + | |||
| + | 1. Memahami konsep-konsep dan prinsip-prinsip dasar dalam metode numerik seperti:Persamaan algoritma, aljabar, pencocokan kurva, persamaan diferensial parsial, dan lainnya | ||
| + | |||
| + | 2. Mengerti dan mampu menerapkan pemahaman atau aplikasi terhadap konsep metode numerik | ||
| + | |||
| + | 3. Mampu menerapkan metode numerik dalam hal terkait persoalan keteknikan | ||
| + | |||
| + | 4. Mendapat added value (nilai tambah)/adab sehingga mahasiswa menjadi orang yang memiliki budi pekerti yang baik | ||
| + | |||
| + | Selain empat poin yang telah disebutkan, Mahasiswa juga mendapatkan pelajaran terkait penggunaan aplikasi OpenModelica. Dimana Mahasiswa dijelaskan dengan sangat sistematis mengenai penggunaan sederhana OpenModelica. | ||
| + | |||
| + | Untuk memenuhi tugas minggu pertama terkait pengantar aplikasi OpenModelica, saya telah membuat model matematis untuk menyelesaikan problem fisika dengan tema Hukum Kekekalan Momentum | ||
| + | |||
| + | LINK TUGAS : https://youtu.be/JjA5Otn82pY | ||
| + | [[File:TugasPer1.mp4|720px|center]] | ||
| + | |||
| + | |||
| + | == Perkuliahan Minggu Ke-2 (18 November 2020) == | ||
| + | |||
| + | '''Catatan Penting''' | ||
| + | |||
| + | Menjadi pribadi yang lebih baik dari hari kemarin, bahwa menjadi manusia haruslah terus berkembang setiap harinya, merupakan pesan moral sekaligus pelajaran hidup pembuka kuliah yang disampaikan Pak Dai pada perkuliahan kali ini. | ||
| + | |||
| + | Beberapa hal penting yang pahami pada perkuliahan kali ini adalah: | ||
| + | |||
| + | - Metode Numerik sangat mengassist perhitungan di bidang engineering, faktor cepat dan akurat memegang peranan penting dalam penggunaannya di lapangan. | ||
| + | |||
| + | - Efisiensi adalah alasan utama mengapa kita sebagai calon engineer semestinya memiliki keahlian metode numerik, terutama pemodelan/ | ||
| + | |||
| + | - Berbicara mengenai pemodelan, Pak Dai sempat membahas mengenai mengapa pada perkuliahan metode numerik beliau memilih menggunakan OpenModellica dibanding bahasa pemrograman | ||
| + | lain, seperti Fortran dan C, beliau menekankan bahwa Modelica merupakan bahasa pemodelan, hal ini menjadikannya tools yang mudah dioperasikan oleh kami yang berlatar | ||
| + | belakang bukan dari Computer Science. | ||
| + | |||
| + | - Selain itu, Modelica adalah aplikasi yang bersifat Open-Source alias gratis, sehingga mahasiswa tidak perlu mengeluarkan biaya sepeserpun. | ||
| + | |||
| + | '''Tugas Minggu Ke-2''' | ||
| + | |||
| + | Pak Dai menugaskan kami untuk membuat sebuah class dan fungsi panggil. Disini saya akan mengerjakan sebuah soal Aljabar Linier dan mencari penyelesaiannya menggunakan perintah Modelica.Math.Matrices.solve | ||
| + | [[File:TugasPer2.mp4|720px|center]] | ||
| + | |||
| + | |||
| + | == Perkuliahan Minggu Ke-3 (25 November 2020) == | ||
| + | Pada perkuliahan ini Pak Dai menjelaskan tentang beberapa pengaplikasian metode numerik di dunia engineering, antara lain | ||
| + | |||
| + | - Computational Fluid Dynamic | ||
| + | |||
| + | - Metode Stokastik | ||
| + | |||
| + | - Finite Element Analysis | ||
| + | |||
| + | Sebelum membahas tentang aplikasi metode numerik pada permasalahan engineering, kita mereview tugas minggu ke-2 mengenai class dan function panggil, kemudian ternyata satu kelas masih kurang mencapai target Pak Dai, karena masih menggunakan function yang sudah disediakan oleh OpenModelica dan bukan membuat sendiri. Akhirnya cristo mencoba menjelaskan penyelesaian yang sudah dia buat seperti di bawah ini : | ||
| + | |||
| + | // Gauss-Jordan Algorithm | ||
| + | // Transforms input matrix A into reduced row echelon form matrix B | ||
| + | // Christopher S.E. November 2020 | ||
| + | input Real [:,:] A; // An augmented matrix of m*n | ||
| + | output Real [:,:] B; // Output matrix in reduced row echelon form | ||
| + | // Local variables | ||
| + | |||
| + | protected | ||
| + | Integer h = 1; // Initialize pivot row | ||
| + | Integer k = 1; // Initialize pivot column | ||
| + | Integer m = size(A,1); // Number of rows in matrix | ||
| + | Integer n = size(A,2); // Number of columns in matrix | ||
| + | Integer c = 0; // Index counter | ||
| + | Integer max_row; // Row index of max number in pivot column | ||
| + | |||
| + | Real [:] pivot_column; // Vector containing pivot column data | ||
| + | Real [:] pivot_row; // Vector containing backwards pivot row data | ||
| + | Real [:,:] temp_array; // Stores matrix data when switching rows | ||
| + | Real r; // Ratio value used for row operations | ||
| + | |||
| + | // Limit to handle floating point errors | ||
| + | Real float_error = 10e-10; | ||
| + | |||
| + | algorithm | ||
| + | |||
| + | // Transfer input matrix A into variable B | ||
| + | B := A; | ||
| + | |||
| + | while h <= m and k <= n loop | ||
| + | |||
| + | // Dealing with floating point errors | ||
| + | for i in 1:m loop | ||
| + | for j in 1:n loop | ||
| + | if abs(B[i,j]) <= float_error then | ||
| + | B[i,j] := 0; | ||
| + | end if; | ||
| + | end for; | ||
| + | end for; | ||
| + | |||
| + | // Finding the pivot | ||
| + | pivot_column := {B[i,h] for i in h:m}; | ||
| + | |||
| + | // Get position index of lowest row with greatest pivot number | ||
| + | c:= h-1; | ||
| + | for element in pivot_column loop | ||
| + | c := c+1; | ||
| + | if abs(element) == max(abs(pivot_column)) then | ||
| + | max_row := c; | ||
| + | end if; | ||
| + | end for; | ||
| + | |||
| + | // No pivot in this column, move on to next column | ||
| + | if B[max_row, k] == 0 then | ||
| + | k := k+1; | ||
| + | |||
| + | else | ||
| + | // Swap Rows h <-> max_row | ||
| + | temp_array := B; | ||
| + | temp_array[h] := B[max_row]; | ||
| + | temp_array[max_row] := B[h]; | ||
| + | B:= temp_array; | ||
| + | |||
| + | // Divide pivot row by pivot number | ||
| + | B[h] := B[h]/B[h,k]; | ||
| + | |||
| + | // For all rows below the pivot | ||
| + | for i in (h+1):m loop | ||
| + | |||
| + | // Store the ratio of the row to the pivot | ||
| + | r := B[i,k] / B[h,k]; | ||
| + | |||
| + | // Set lower part of pivot column to zero | ||
| + | B[i,k] := 0; | ||
| + | |||
| + | // Operations on the remaining row elements | ||
| + | for j in (k+1):n loop | ||
| + | B[i,j] := B[i,j] - B[h,j] * r; | ||
| + | end for; | ||
| + | |||
| + | end for; | ||
| + | |||
| + | // Move on to next pivot row and column | ||
| + | h := h+1; | ||
| + | k := k+1; | ||
| + | |||
| + | end if; | ||
| + | |||
| + | end while; | ||
| + | |||
| + | // The matrix is now in row echelon form | ||
| + | |||
| + | // Set values of (h,k) to (m,n) | ||
| + | h := m; | ||
| + | k := n; | ||
| + | |||
| + | while h >= 1 and k >=1 loop | ||
| + | |||
| + | // Dealing with floating point errors | ||
| + | for i in 1:m loop | ||
| + | for j in 1:n loop | ||
| + | if abs(B[i,j]) <= float_error then | ||
| + | B[i,j] := 0; | ||
| + | end if; | ||
| + | end for; | ||
| + | end for; | ||
| + | |||
| + | // Finding the pivot | ||
| + | pivot_row := {B[h,i] for i in 1:k}; | ||
| + | |||
| + | // Get position index k of pivot | ||
| + | c := 0; | ||
| + | for element in pivot_row loop | ||
| + | c := c+1; | ||
| + | if element <> 0 then | ||
| + | break; | ||
| + | end if; | ||
| + | end for; | ||
| + | k := c; | ||
| + | |||
| + | // No pivot in this row, move on to next row | ||
| + | if B[h, k] == 0 then | ||
| + | '''Bold text''' h := h-1; | ||
| + | |||
| + | else | ||
| + | |||
| + | // Perform row operations | ||
| + | for i in 1:(h-1) loop | ||
| + | r := B[i,k]; | ||
| + | B[i] := B[i] - B[h] * r; | ||
| + | end for; | ||
| + | |||
| + | // Move on to next pivot row and column | ||
| + | h := h-1; | ||
| + | k := k-1; | ||
| + | |||
| + | end if; | ||
| + | |||
| + | end while; | ||
| + | |||
| + | // The matrix is now in reduced row echelon form | ||
| + | |||
| + | end GaussJordan; | ||
| + | |||
| + | '''Tugas Minggu Ke-3''' | ||
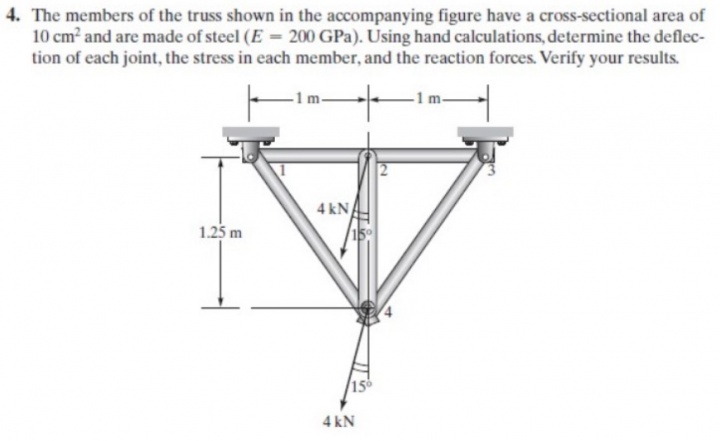
| + | [[File:soal_m3.jpg|720px|center]] | ||
| + | |||
| + | '''Persamaan''' | ||
| + | |||
| + | class Trusses_Tugas3_MRayhanFatur | ||
| + | |||
| + | parameter Integer N=8; //Global matrice = 2*points connected | ||
| + | parameter Real A=0.001; //Area m2 | ||
| + | parameter Real E=200e9; //Pa | ||
| + | Real G[N,N]; //global | ||
| + | Real Ginitial[N,N]; //global | ||
| + | Real Sol[N]; //global dispplacement | ||
| + | Real X[N]={0,0,-1035.2762,-3863.7033,0,0,-1035.2762,-3863.7033}; | ||
| + | Real R[N]; //global reaction force | ||
| + | Real SolMat[N,1]; | ||
| + | Real XMat[N,1]; | ||
| + | |||
| + | //boundary condition | ||
| + | Integer b1=1; | ||
| + | Integer b2=3; | ||
| + | |||
| + | //truss 1 | ||
| + | parameter Real X1=0; //degree between truss | ||
| + | Real k1=A*E/1; | ||
| + | Real K1[4,4]; //stiffness matrice | ||
| + | Integer p1a=1; | ||
| + | Integer p1b=2; | ||
| + | Real G1[N,N]; | ||
| + | |||
| + | //truss 2 | ||
| + | parameter Real X2=0; //degree between truss | ||
| + | Real k2=A*E/1; | ||
| + | Real K2[4,4]; //stiffness matrice | ||
| + | Integer p2a=2; | ||
| + | Integer p2b=3; | ||
| + | Real G2[N,N]; | ||
| + | |||
| + | //truss 3 | ||
| + | parameter Real X3=90; //degree between truss | ||
| + | Real k3=A*E/1.25; | ||
| + | Real K3[4,4]; //stiffness matrice | ||
| + | Integer p3a=2; | ||
| + | Integer p3b=4; | ||
| + | Real G3[N,N]; | ||
| + | |||
| + | //truss 4 | ||
| + | parameter Real X4=90+38.6598; //degree between truss | ||
| + | Real k4=A*E/1.6; | ||
| + | Real K4[4,4]; //stiffness matrice | ||
| + | Integer p4a=1; | ||
| + | Integer p4b=4; | ||
| + | Real G4[N,N]; | ||
| + | |||
| + | //truss 5 | ||
| + | parameter Real X5=90-38.6598; //degree between truss | ||
| + | Real k5=A*E/1.6; | ||
| + | Real K5[4,4]; //stiffness matrice | ||
| + | Integer p5a=3; | ||
| + | Integer p5b=4; | ||
| + | Real G5[N,N]; | ||
| + | |||
| + | /* | ||
| + | for each truss, please ensure pXa is lower then pXb (X represents truss element number) | ||
| + | */ | ||
| + | |||
| + | algorithm | ||
| + | |||
| + | //creating global matrice | ||
| + | K1:=Stiffness_Matrices(X1); | ||
| + | G1:=k1*Local_Global(K1,N,p1a,p1b); | ||
| + | |||
| + | K2:=Stiffness_Matrices(X2); | ||
| + | G2:=k2*Local_Global(K2,N,p2a,p2b); | ||
| + | |||
| + | K3:=Stiffness_Matrices(X3); | ||
| + | G3:=k3*Local_Global(K3,N,p3a,p3b); | ||
| + | |||
| + | K4:=Stiffness_Matrices(X4); | ||
| + | G4:=k4*Local_Global(K4,N,p4a,p4b); | ||
| + | |||
| + | K5:=Stiffness_Matrices(X5); | ||
| + | G5:=k5*Local_Global(K5,N,p5a,p5b); | ||
| + | |||
| + | G:=G1+G2+G3+G4+G5; | ||
| + | Ginitial:=G; | ||
| + | |||
| + | //implementing boundary condition | ||
| + | for i in 1:N loop | ||
| + | G[2*b1-1,i]:=0; | ||
| + | G[2*b1,i]:=0; | ||
| + | G[2*b2-1,i]:=0; | ||
| + | G[2*b2,i]:=0; | ||
| + | end for; | ||
| + | |||
| + | G[2*b1-1,2*b1-1]:=1; | ||
| + | G[2*b1,2*b1]:=1; | ||
| + | G[2*b2-1,2*b2-1]:=1; | ||
| + | G[2*b2,2*b2]:=1; | ||
| + | |||
| + | //solving displacement | ||
| + | Sol:=Gauss_Jordan(N,G,X); | ||
| + | |||
| + | //solving reaction force | ||
| + | SolMat:=matrix(Sol); | ||
| + | XMat:=matrix(X); | ||
| + | R:=Reaction_Trusses(N,Ginitial,SolMat,XMat); | ||
| + | |||
| + | end Trusses_Tugas3_MRayhanFatur; | ||
| + | |||
| + | '''Fungsi Panggil Yang Digunakan''' | ||
| + | {| class="wikitable" | ||
| + | |- | ||
| + | | style='border-style: none none solid solid;' | | ||
| + | ''Matrice Transformation'' | ||
| + | |||
| + | function Stiffness_Matrices | ||
| + | input Real A; | ||
| + | Real Y; | ||
| + | output Real X[4,4]; | ||
| + | Real float_error = 10e-10; | ||
| + | |||
| + | final constant Real pi=2*Modelica.Math.asin(1.0); | ||
| + | |||
| + | algorithm | ||
| + | |||
| + | Y:=A/180*pi; | ||
| + | |||
| + | X:=[(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y); | ||
| + | |||
| + | Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2; | ||
| + | |||
| + | -(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y); | ||
| + | |||
| + | -Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2]; | ||
| + | |||
| + | for i in 1:4 loop | ||
| + | for j in 1:4 loop | ||
| + | if abs(X[i,j]) <= float_error then | ||
| + | X[i,j] := 0; | ||
| + | end if; | ||
| + | end for; | ||
| + | end for; | ||
| + | |||
| + | end Stiffness_Matrices; | ||
| + | |||
| + | | style="width: 20cm;"| | ||
| + | ''Global Element Matrice'' | ||
| + | |||
| + | function Local_Global | ||
| + | input Real Y[4,4]; | ||
| + | input Integer B; | ||
| + | input Integer p1; | ||
| + | input Integer p2; | ||
| + | output Real G[B,B]; | ||
| + | |||
| + | algorithm | ||
| + | |||
| + | for i in 1:B loop | ||
| + | for j in 1:B loop | ||
| + | G[i,j]:=0; | ||
| + | end for; | ||
| + | end for; | ||
| + | |||
| + | G[2*p1,2*p1]:=Y[2,2]; | ||
| + | G[2*p1-1,2*p1-1]:=Y[1,1]; | ||
| + | G[2*p1,2*p1-1]:=Y[2,1]; | ||
| + | G[2*p1-1,2*p1]:=Y[1,2]; | ||
| + | |||
| + | G[2*p2,2*p2]:=Y[4,4]; | ||
| + | G[2*p2-1,2*p2-1]:=Y[3,3]; | ||
| + | G[2*p2,2*p2-1]:=Y[4,3]; | ||
| + | G[2*p2-1,2*p2]:=Y[3,4]; | ||
| + | |||
| + | G[2*p2,2*p1]:=Y[4,2]; | ||
| + | G[2*p2-1,2*p1-1]:=Y[3,1]; | ||
| + | G[2*p2,2*p1-1]:=Y[4,1]; | ||
| + | G[2*p2-1,2*p1]:=Y[3,2]; | ||
| + | |||
| + | G[2*p1,2*p2]:=Y[2,4]; | ||
| + | G[2*p1-1,2*p2-1]:=Y[1,3]; | ||
| + | G[2*p1,2*p2-1]:=Y[2,3]; | ||
| + | G[2*p1-1,2*p2]:=Y[1,4]; | ||
| + | |||
| + | end Local_Global; | ||
| + | |||
| + | | style="width: 20cm;"| | ||
| + | ''Reaction Matrice Equation'' | ||
| + | |||
| + | function Reaction_Trusses | ||
| + | input Integer N; | ||
| + | input Real A[N,N]; | ||
| + | input Real B[N,1]; | ||
| + | input Real C[N,1]; | ||
| + | Real X[N,1]; | ||
| + | output Real Sol[N]; | ||
| + | Real float_error = 10e-10; | ||
| + | |||
| + | algorithm | ||
| + | X:=A*B-C; | ||
| + | |||
| + | for i in 1:N loop | ||
| + | if abs(X[i,1]) <= float_error then | ||
| + | X[i,1] := 0; | ||
| + | end if; | ||
| + | end for; | ||
| + | |||
| + | for i in 1:N loop | ||
| + | Sol[i]:=X[i,1]; | ||
| + | end for; | ||
| + | |||
| + | end Reaction_Trusses; | ||
| + | |||
| + | |} | ||
| + | |||
| + | '''Gauss Jordan''' | ||
| + | {| class="wikitable" | ||
| + | |- | ||
| + | | style='border-style: none none solid solid;' | | ||
| + | |||
| + | function Gauss_Jordan | ||
| + | input Integer N; | ||
| + | input Real A[N,N]; | ||
| + | input Real B[N]; | ||
| + | output Real X[N]; | ||
| + | Real float_error = 10e-10; | ||
| + | |||
| + | algorithm | ||
| + | X:=Modelica.Math.Matrices.solve(A,B); | ||
| + | |||
| + | for i in 1:N loop | ||
| + | if abs(X[i]) <= float_error then | ||
| + | X[i] := 0; | ||
| + | end if; | ||
| + | end for; | ||
| + | |||
| + | end Gauss_Jordan; | ||
| + | |||
| + | |||
| + | '''Grafik''' | ||
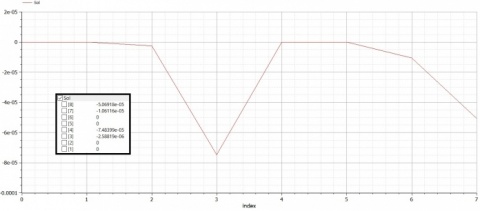
| + | [[File:Trusses_2 DisplacementGraph_Fatur.jpg|480px|thumb|left|Grafik Displacement]] | ||
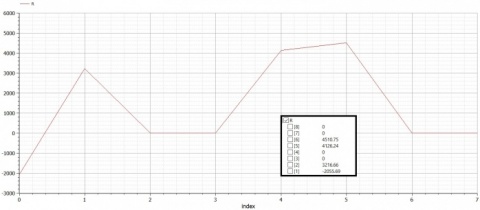
| + | [[File:Trusses_2 Reaction_Fatur.jpg|480px|thumb|right|Grafik Reaction Forces]] | ||
| + | |||
| + | |||
| + | |||
| + | '''KUIS''' | ||
| + | |||
| + | [[File:soal_m3.jpg|720px|center]] | ||
| + | |||
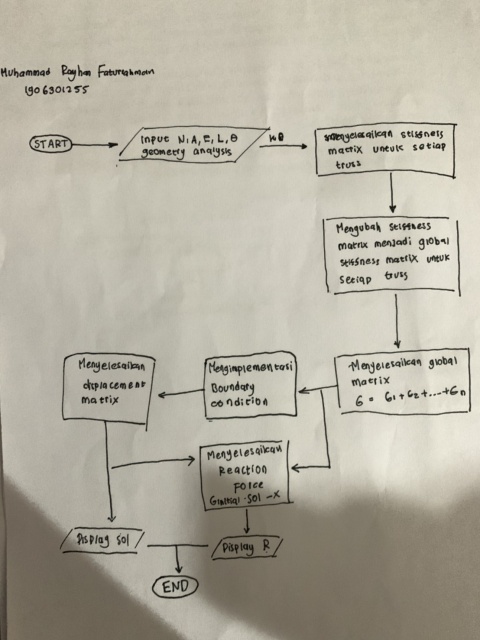
| + | Flowchart | ||
| + | |||
| + | [[File:flowcharttruss.jpg|480px|center]] | ||
| + | |||
| + | == Minggu Ke-4 == | ||
| + | |||
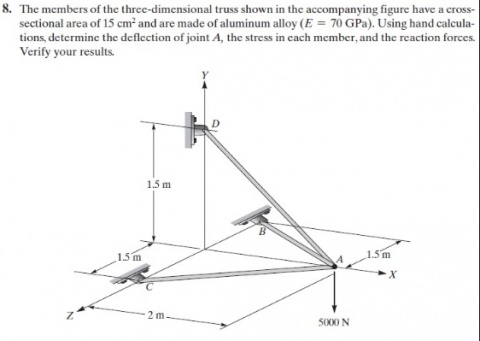
| + | Pada minggu ke-4 ini, perkuliahan terhalang oleh libur karena adanya Pilkada, sehingga Pak Dai memberikan tugas untuk mengerjakan soal di bawah ini: | ||
| + | |||
| + | |||
| + | [[File:1607498641008.jpg|480px|center]] | ||
| + | |||
| + | |||
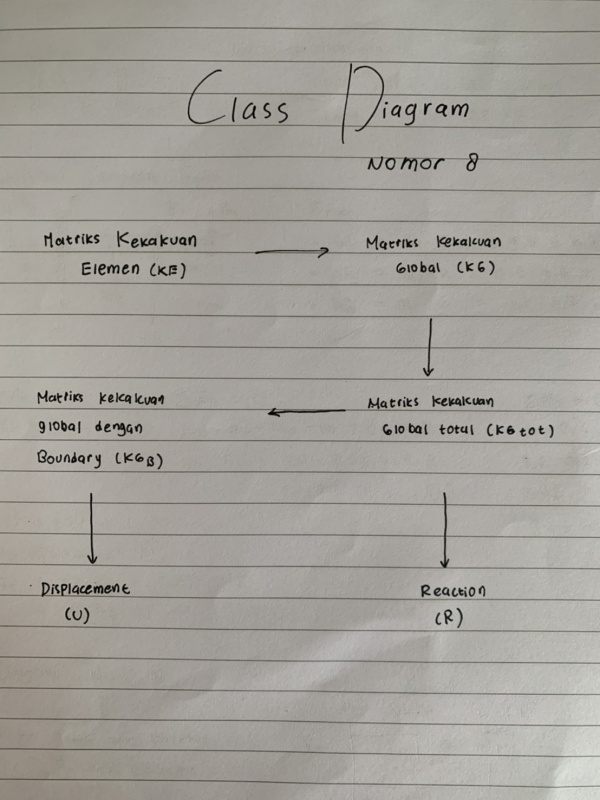
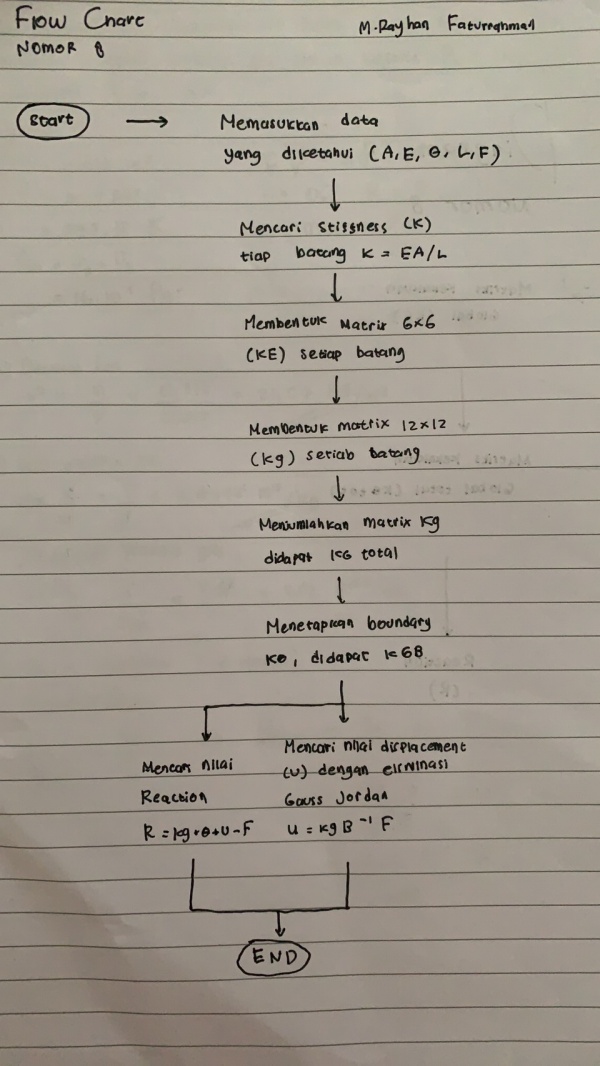
| + | Untuk memahami alur logika untuk mengerjakan soal di atas dengan metode numerik, saya mencoba membuat Class Diagram dan Flow Chart terkait soal berikut: | ||
| + | |||
| + | |||
| + | [[File:DC8.jpg|600px|Displacement]][[File:FC8.jpg|600px|Displacement]] | ||
| + | |||
| + | |||
| + | Dan berikut penjabaran dari flow chart di atas | ||
| + | |||
| + | |||
| + | [[File:FBD8.jpg|480px|center]] | ||
| + | |||
| + | |||
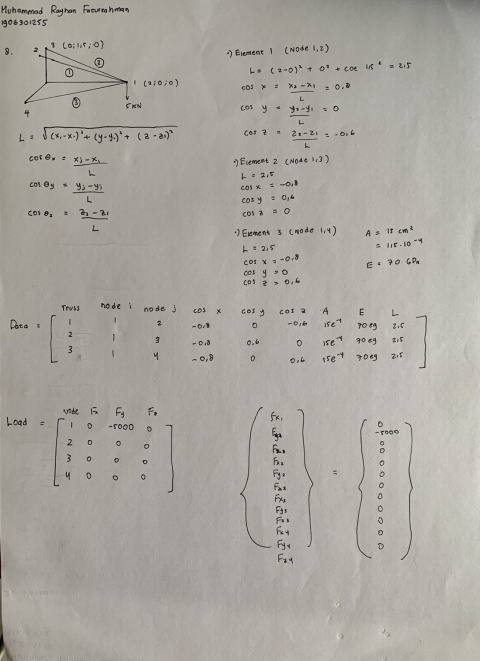
| + | Berikut adalah pemrograman OpenModellica saya | ||
| + | |||
| + | |||
| + | function StiffnessMatrixElement | ||
| + | input Real [:,9] inisiasi_mat; | ||
| + | output Real [size(inisiasi_mat,1),6,6] Ke_mat; | ||
| + | |||
| + | protected | ||
| + | Real cos_x; | ||
| + | Real cos_y; | ||
| + | Real cos_z; | ||
| + | Real [6] StiffTrig; | ||
| + | Real [6,6] StiffTrans; | ||
| + | Real [size(inisiasi_mat,1)] k_vec; | ||
| + | |||
| + | algorithm | ||
| + | k_vec := {(inisiasi_mat[i,7] * inisiasi_mat[i,8] / inisiasi_mat[i,9]) for i in 1:size(inisiasi_mat,1)}; | ||
| + | |||
| + | // Finding stiffness matrix of each element member | ||
| + | for i in 1:size(inisiasi_mat,1) loop | ||
| + | |||
| + | // Clearing the matrices | ||
| + | StiffTrig := zeros(6); | ||
| + | StiffTrans := zeros(6,6); | ||
| + | |||
| + | // Converting degrees to radians | ||
| + | cos_x := inisiasi_mat[i,4]; | ||
| + | cos_y := inisiasi_mat[i,5]; | ||
| + | cos_z := inisiasi_mat[i,6]; | ||
| + | |||
| + | // {cos^2, sin^2, sincos} | ||
| + | StiffTrig := {(cos_x)^2, | ||
| + | (cos_y)^2, | ||
| + | (cos_z)^2, | ||
| + | (cos_x*cos_y), | ||
| + | (cos_x*cos_z), | ||
| + | (cos_y*cos_z)}; | ||
| + | |||
| + | // Construct stiffness transformation matrix | ||
| + | StiffTrans := [ StiffTrig[1], StiffTrig[4], StiffTrig[5], -1*StiffTrig[1], -1*StiffTrig[4], -1*StiffTrig[5]; | ||
| + | StiffTrig[4], StiffTrig[2], StiffTrig[6], -1*StiffTrig[4], -1*StiffTrig[2], -1*StiffTrig[6]; | ||
| + | StiffTrig[5], StiffTrig[6], StiffTrig[3], -1*StiffTrig[5], -1*StiffTrig[6], -1*StiffTrig[3]; | ||
| + | -1*StiffTrig[1], -1*StiffTrig[4], -1*StiffTrig[5], StiffTrig[1], StiffTrig[4], StiffTrig[5]; | ||
| + | -1*StiffTrig[4], -1*StiffTrig[2], -1*StiffTrig[6], StiffTrig[4], StiffTrig[2], StiffTrig[6]; | ||
| + | -1*StiffTrig[5], -1*StiffTrig[6], -1*StiffTrig[3], StiffTrig[5], StiffTrig[6], StiffTrig[3]]; | ||
| + | |||
| + | // Multiply in stiffness constant of element, add final stiffness matrix to Ke_mat | ||
| + | for m in 1:6 loop | ||
| + | for n in 1:6 loop | ||
| + | Ke_mat[i,m,n] := k_vec[i] * StiffTrans[m,n]; | ||
| + | end for; | ||
| + | end for; | ||
| + | |||
| + | end for; | ||
| + | end StiffnessMatrixElement; | ||
| + | |||
| + | |||
| + | |} | ||
| + | |||
| + | {| class="wikitable" | ||
| + | |- | ||
| + | | style='border-style: none none solid solid;' | | ||
| + | |||
| + | function StiffnessMatrixGlobal | ||
| + | input Integer [:,2] n; | ||
| + | input Integer x; | ||
| + | input Integer y; | ||
| + | input Real [y,6,6] Ke_mat; | ||
| + | output Real [y,x,x] Kg_mat; | ||
| + | |||
| + | algorithm | ||
| + | for i in 1:y loop | ||
| + | for a in 1:x loop | ||
| + | for b in 1:x loop | ||
| + | Kg_mat[i,a,b]:=0; | ||
| + | end for; | ||
| + | end for; | ||
| + | end for; | ||
| + | |||
| + | for i in 1:y loop | ||
| + | Kg_mat[i,3*n[i,1],3*n[i,1]]:=Ke_mat[i,3,3]; | ||
| + | Kg_mat[i,3*n[i,1],3*n[i,1]-1]:=Ke_mat[i,3,2]; | ||
| + | Kg_mat[i,3*n[i,1],3*n[i,1]-2]:=Ke_mat[i,3,1]; | ||
| + | Kg_mat[i,3*n[i,1]-1,3*n[i,1]]:=Ke_mat[i,2,3]; | ||
| + | Kg_mat[i,3*n[i,1]-1,3*n[i,1]-1]:=Ke_mat[i,2,2]; | ||
| + | Kg_mat[i,3*n[i,1]-1,3*n[i,1]-2]:=Ke_mat[i,2,1]; | ||
| + | Kg_mat[i,3*n[i,1]-2,3*n[i,1]]:=Ke_mat[i,1,3]; | ||
| + | Kg_mat[i,3*n[i,1]-2,3*n[i,1]-1]:=Ke_mat[i,1,2]; | ||
| + | Kg_mat[i,3*n[i,1]-2,3*n[i,1]-2]:=Ke_mat[i,1,1]; | ||
| + | |||
| + | Kg_mat[i,3*n[i,2],3*n[i,2]]:=Ke_mat[i,6,6]; | ||
| + | Kg_mat[i,3*n[i,2],3*n[i,2]-1]:=Ke_mat[i,6,5]; | ||
| + | Kg_mat[i,3*n[i,2],3*n[i,2]-2]:=Ke_mat[i,6,4]; | ||
| + | Kg_mat[i,3*n[i,2]-1,3*n[i,2]]:=Ke_mat[i,5,6]; | ||
| + | Kg_mat[i,3*n[i,2]-1,3*n[i,2]-1]:=Ke_mat[i,5,5]; | ||
| + | Kg_mat[i,3*n[i,2]-1,3*n[i,2]-2]:=Ke_mat[i,5,4]; | ||
| + | Kg_mat[i,3*n[i,2]-2,3*n[i,2]]:=Ke_mat[i,4,6]; | ||
| + | Kg_mat[i,3*n[i,2]-2,3*n[i,2]-1]:=Ke_mat[i,4,5]; | ||
| + | Kg_mat[i,3*n[i,2]-2,3*n[i,2]-2]:=Ke_mat[i,4,4]; | ||
| + | |||
| + | Kg_mat[i,3*n[i,2],3*n[i,1]]:=Ke_mat[i,6,3]; | ||
| + | Kg_mat[i,3*n[i,2],3*n[i,1]-1]:=Ke_mat[i,6,2]; | ||
| + | Kg_mat[i,3*n[i,2],3*n[i,1]-2]:=Ke_mat[i,6,1]; | ||
| + | Kg_mat[i,3*n[i,2]-1,3*n[i,1]]:=Ke_mat[i,5,3]; | ||
| + | Kg_mat[i,3*n[i,2]-1,3*n[i,1]-1]:=Ke_mat[i,5,2]; | ||
| + | Kg_mat[i,3*n[i,2]-1,3*n[i,1]-2]:=Ke_mat[i,5,1]; | ||
| + | Kg_mat[i,3*n[i,2]-2,3*n[i,1]]:=Ke_mat[i,4,3]; | ||
| + | Kg_mat[i,3*n[i,2]-2,3*n[i,1]-1]:=Ke_mat[i,4,2]; | ||
| + | Kg_mat[i,3*n[i,2]-2,3*n[i,1]-2]:=Ke_mat[i,4,1]; | ||
| + | |||
| + | Kg_mat[i,3*n[i,1],3*n[i,2]]:=Ke_mat[i,3,6]; | ||
| + | Kg_mat[i,3*n[i,1],3*n[i,2]-1]:=Ke_mat[i,3,5]; | ||
| + | Kg_mat[i,3*n[i,1],3*n[i,2]-2]:=Ke_mat[i,3,4]; | ||
| + | Kg_mat[i,3*n[i,1]-1,3*n[i,2]]:=Ke_mat[i,2,6]; | ||
| + | Kg_mat[i,3*n[i,1]-1,3*n[i,2]-1]:=Ke_mat[i,2,5]; | ||
| + | Kg_mat[i,3*n[i,1]-1,3*n[i,2]-2]:=Ke_mat[i,2,4]; | ||
| + | Kg_mat[i,3*n[i,1]-2,3*n[i,2]]:=Ke_mat[i,1,6]; | ||
| + | Kg_mat[i,3*n[i,1]-2,3*n[i,2]-1]:=Ke_mat[i,1,5]; | ||
| + | Kg_mat[i,3*n[i,1]-2,3*n[i,2]-2]:=Ke_mat[i,1,4]; | ||
| + | end for; | ||
| + | end StiffnessMatrixGlobal; | ||
| + | |||
| + | {| class="wikitable" | ||
| + | |- | ||
| + | | style='border-style: none none solid solid;' | | ||
| + | |||
| + | function GaussJordan | ||
| + | input Integer x; | ||
| + | input Real [x,x] KgB_met; | ||
| + | input Real [x] load_met; | ||
| + | output Real [x] U_met; | ||
| + | |||
| + | protected | ||
| + | Real float_error = 10e-10; | ||
| + | |||
| + | algorithm | ||
| + | U_met:=Modelica.Math.Matrices.solve(KgB_met,load_met); | ||
| + | |||
| + | for i in 1:x loop | ||
| + | if abs(U_met[i]) <= float_error then | ||
| + | U_met[i] := 0; | ||
| + | end if; | ||
| + | end for; | ||
| + | |||
| + | end GaussJordan; | ||
| + | |||
| + | |||
| + | |} | ||
| + | |||
| + | |||
| + | {| class="wikitable" | ||
| + | |- | ||
| + | | style='border-style: none none solid solid;' | | ||
| + | |||
| + | function ReactionForce | ||
| + | input Integer x; | ||
| + | input Real [x,x] KgTot_met; | ||
| + | input Real [x] U_met; | ||
| + | input Real [x] load_met; | ||
| + | output Real [x] R_met; | ||
| + | |||
| + | algorithm | ||
| + | R_met := (KgTot_met*U_met)-load_met; | ||
| + | end ReactionForce; | ||
| + | |||
| + | |||
| + | |} | ||
| + | |||
| + | |||
| + | {| class="wikitable" | ||
| + | |- | ||
| + | | style='border-style: none none solid solid;' | | ||
| + | |||
| + | class TugasWeek4 | ||
| + | parameter Real [:,9] inisiasi = [1, 1, 2, -0.8, 0, -0.6, 15e-4, 70e9, 2.5; | ||
| + | 2, 1, 3, -0.8, -0.6, 0, 15e-4, 70e9, 2.5; | ||
| + | 3, 1, 4, -0.8, 0, 0.6, 15e-4, 70e9, 2.5]; | ||
| + | |||
| + | parameter Integer [:,2] node = [1, 2; | ||
| + | 1, 3; | ||
| + | 1, 4]; | ||
| + | |||
| + | parameter Integer y = size(node,1); | ||
| + | |||
| + | parameter Integer x = 3*(size(node_load,1)); | ||
| + | |||
| + | parameter Integer z = size(Boundary,1); | ||
| + | |||
| + | parameter Integer [:] Boundary = {2,3,4}; | ||
| + | |||
| + | parameter Real [:,4] node_load = [1, 0, -5000, 0; | ||
| + | 2, 0, 0, 0; | ||
| + | 3, 0, 0, 0; | ||
| + | 4, 0, 0, 0]; | ||
| + | |||
| + | parameter Real [x] load = {0,-5000, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0}; | ||
| + | |||
| + | Real [y] k; | ||
| + | |||
| + | Real [y,6,6] Ke; | ||
| + | |||
| + | Real [y,x,x] Kg; | ||
| + | |||
| + | Real [x,x] KgTot; | ||
| + | |||
| + | Real [x,x] KgB; | ||
| + | |||
| + | Real [x] U; | ||
| + | |||
| + | Real [x] R; | ||
| + | |||
| + | equation | ||
| + | k = {(inisiasi[i,7] * inisiasi[i,8] / inisiasi[i,9]) for i in 1:y}; | ||
| + | |||
| + | Ke = StiffnessMatrixElement(inisiasi); | ||
| + | |||
| + | Kg = StiffnessMatrixGlobal(node, x, y, Ke); | ||
| + | |||
| + | KgTot = SumStiffnessMatrixGlobal(x, y, Kg); | ||
| + | |||
| + | KgB = BoundaryStiffnessMatrixGlobal(x, z, KgTot, Boundary); | ||
| + | |||
| + | U = GaussJordan(x, KgB, load); | ||
| + | |||
| + | R = ReactionForce(x, KgTot, U, load); | ||
| + | |||
| + | end TugasWeek4; | ||
| + | |||
| + | |} | ||
| + | |||
| + | |||
| + | |||
| + | == Kuliah Minggu Ke-7 (23 Desember 2020) == | ||
| + | |||
| + | |||
| + | '''TUJUAN''' | ||
| + | |||
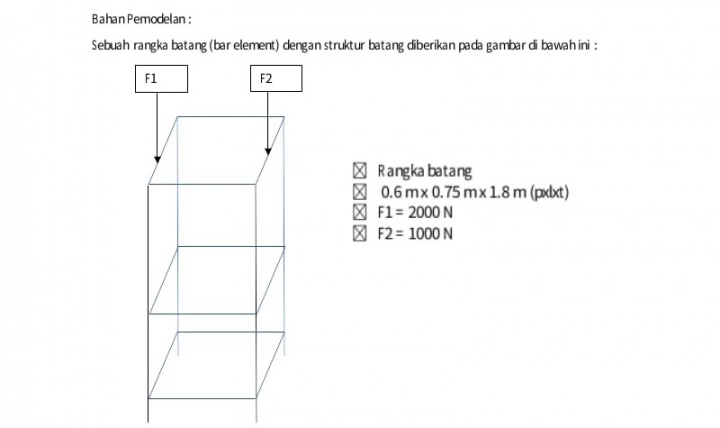
| + | Kuliah kali ini kita melaksanakan kuis mengenai optimasi 3D Truss menggunakan class algoritma yang sebelumnya telah disusun pada kuliah minggu lalu. 3D Truss yang dimaksud seperti ini. | ||
| + | |||
| + | |||
| + | [[File:TubesMetnumRayhan.jpg|720px|center]] | ||
| + | |||
| + | [[File:Soaltubes1.jpg|720px|center]] | ||
| + | |||
| + | |||
| + | Kuis ini juga sekaligus mendukung pengerjaan tugas besar, pada tugas besar Pak Dai meminta untuk menentukan jenis material, harga material, dan luas cross sectional area dari truss. Kami juga diminta untuk melakukan optimasi dan membentuk curve fitting pada variabel harga | ||
| + | |||
| + | '''PENGERJAAN''' | ||
| + | |||
| + | Diketahui: panjang= 0,6 m lebar= 0,75 m tinggi= 1,6 m F1= 2000 N F2= 1000 N | ||
| + | |||
| + | kemudian diasumsikan | ||
| + | |||
| + | -Beban akan terdistribusi hanya pada node (karena bersifat trusses). | ||
| + | |||
| + | -Safety factor minimal bernilai 2. | ||
| + | |||
| + | -Batas displacement 0,001 m sebelum buckling (pada truss paling atas). | ||
| + | |||
| + | -Ketinggian trusses pada tiap lantai sama yaitu 0,6 m. | ||
| + | |||
| + | |||
| + | berikutnya: Untuk jenis material yang sama: | ||
| + | |||
| + | -Mencari harga untuk 5 ukuran batang dengan material yang sama yaitu SS304. | ||
| + | |||
| + | -Mengitung nilai safety factor pada 5 ukuran batang dengan coding awal. | ||
| + | |||
| + | -Membuat rasio antara safety factor dengan harga total. | ||
| + | |||
| + | -Membuat persamaan antara rasio (safe/harga) dengan area dengan metode curve fitting. | ||
| + | |||
| + | -Melakukan optimasi menggunakan metode golden section. | ||
| + | |||
| + | |||
| + | Untuk area penampang yang sama: | ||
| + | |||
| + | -Mencari harga untuk 3 jenis material dengan area penampang yang sama yaitu 171 mm^2. | ||
| + | |||
| + | -Mengitung nilai safety factor pada 3 jenis batang dengan coding awal. | ||
| + | |||
| + | -Membuat rasio antara safety factor dengan harga total. | ||
| + | |||
| + | -Membuat persamaan antara rasio (safe/harga) dengan jenis material dengan metode curve fitting. | ||
| + | |||
| + | -Melakukan optimasi menggunakan metode golden section. | ||
| + | |||
| + | |||
| + | == Update Tugas Besar == | ||
| + | |||
| + | Untuk mencari reaction force, stress, dan safety factor, saya menggunakan script di bawah ini | ||
| + | |||
| + | model TugasBesar_Trusses_3d_Safety | ||
| + | //define initial variable | ||
| + | parameter Integer Points=size(P,1); //Number of Points | ||
| + | parameter Integer Trusses=size(C,1); //Number of Trusses | ||
| + | parameter Real Yield=215e6; //Yield Strength (Pa) | ||
| + | parameter Real Area=0.000171; //Area L Profile (Dimension=0.03, Thickness=0,003) (m2) | ||
| + | parameter Real Elas=193e9; //Elasticity SS 304 (Pa) | ||
| + | |||
| + | //define connection | ||
| + | parameter Integer C[:,2]=[1,5; | ||
| + | 2,6; | ||
| + | 3,7; | ||
| + | 4,8; | ||
| + | 5,6; //1st floor | ||
| + | 6,7; //1st floor | ||
| + | 7,8; //1st floor | ||
| + | 5,8; //1st floor | ||
| + | 5,9; | ||
| + | 6,10; | ||
| + | 7,11; | ||
| + | 8,12; | ||
| + | 9,10; //2nd floor | ||
| + | 10,11;//2nd floor | ||
| + | 11,12;//2nd floor | ||
| + | 9,12; //2nd floor | ||
| + | 9,13; | ||
| + | 10,14; | ||
| + | 11,15; | ||
| + | 12,16; | ||
| + | 13,14;//3rd floor | ||
| + | 14,15;//3rd floor | ||
| + | 15,16;//3rd floor | ||
| + | 13,16];//3rd floor | ||
| + | //define coordinates (please put orderly) | ||
| + | parameter Real P[:,6]=[0.3,-0.375,0,1,1,1; //1 | ||
| + | -0.3,-0.375,0,1,1,1; //2 | ||
| + | -0.3,0.375,0,1,1,1; //3 | ||
| + | 0.3,0.375,0,1,1,1; //4 | ||
| + | |||
| + | 0.3,-0.375,0.6,0,0,0; //5 | ||
| + | -0.3,-0.375,0.6,0,0,0; //6 | ||
| + | -0.3,0.375,0.6,0,0,0; //7 | ||
| + | 0.3,0.375,0.6,0,0,0; //8 | ||
| + | |||
| + | 0.3,-0.375,1.2,0,0,0; //9 | ||
| + | -0.3,-0.375,1.2,0,0,0; //10 | ||
| + | -0.3,0.375,1.2,0,0,0; //11 | ||
| + | 0.3,0.375,1.2,0,0,0; //12 | ||
| + | |||
| + | 0.3,-0.375,1.8,0,0,0; //13 | ||
| + | -0.3,-0.375,1.8,0,0,0; //14 | ||
| + | -0.3,0.375,1.8,0,0,0; //15 | ||
| + | 0.3,0.375,1.8,0,0,0]; //16 | ||
| + | //define external force (please put orderly) | ||
| + | parameter Real F[Points*3]={0,0,0, | ||
| + | 0,0,0, | ||
| + | 0,0,0, | ||
| + | 0,0,0, | ||
| + | 0,0,0, | ||
| + | 0,0,0, | ||
| + | 0,0,0, | ||
| + | 0,0,0, | ||
| + | 0,0,0, | ||
| + | 0,0,0, | ||
| + | 0,0,0, | ||
| + | 0,0,0, | ||
| + | 0,0,-500, | ||
| + | 0,0,-1000, | ||
| + | 0,0,-1000, | ||
| + | 0,0,-500}; | ||
| + | //solution | ||
| + | Real displacement[N], reaction[N]; | ||
| + | Real check[3]; | ||
| + | Real stress1[Trusses]; | ||
| + | Real safety[Trusses]; | ||
| + | Real dis[3]; | ||
| + | Real Str[3]; | ||
| + | protected | ||
| + | parameter Integer N=3*Points; | ||
| + | Real q1[3], q2[3], g[N,N], G[N,N], G_star[N,N], id[N,N]=identity(N), cx, cy, cz, L, X[3,3]; | ||
| + | Real err=10e-10, ers=10e-4; | ||
| + | algorithm | ||
| + | //Creating Global Matrix | ||
| + | G:=id; | ||
| + | for i in 1:Trusses loop | ||
| + | for j in 1:3 loop | ||
| + | q1[j]:=P[C[i,1],j]; | ||
| + | q2[j]:=P[C[i,2],j]; | ||
| + | end for; | ||
| + | //Solving Matrix | ||
| + | L:=Modelica.Math.Vectors.length(q2-q1); | ||
| + | cx:=(q2[1]-q1[1])/L; | ||
| + | cy:=(q2[2]-q1[2])/L; | ||
| + | cz:=(q2[3]-q1[3])/L; | ||
| + | X:=(Area*Elas/L)*[cx^2,cx*cy,cx*cz; | ||
| + | cy*cx,cy^2,cy*cz; | ||
| + | cz*cx,cz*cy,cz^2]; | ||
| + | //Transforming to global matrix | ||
| + | g:=zeros(N,N); | ||
| + | for m,n in 1:3 loop | ||
| + | g[3*(C[i,1]-1)+m,3*(C[i,1]-1)+n]:=X[m,n]; | ||
| + | g[3*(C[i,2]-1)+m,3*(C[i,2]-1)+n]:=X[m,n]; | ||
| + | g[3*(C[i,2]-1)+m,3*(C[i,1]-1)+n]:=-X[m,n]; | ||
| + | g[3*(C[i,1]-1)+m,3*(C[i,2]-1)+n]:=-X[m,n]; | ||
| + | end for; | ||
| + | G_star:=G+g; | ||
| + | G:=G_star; | ||
| + | end for; | ||
| + | //Implementing boundary | ||
| + | for x in 1:Points loop | ||
| + | if P[x,4] <> 0 then | ||
| + | for a in 1:Points*3 loop | ||
| + | G[(x*3)-2,a]:=0; | ||
| + | G[(x*3)-2,(x*3)-2]:=1; | ||
| + | end for; | ||
| + | end if; | ||
| + | if P[x,5] <> 0 then | ||
| + | for a in 1:Points*3 loop | ||
| + | G[(x*3)-1,a]:=0; | ||
| + | G[(x*3)-1,(x*3)-1]:=1; | ||
| + | end for; | ||
| + | end if; | ||
| + | if P[x,6] <> 0 then | ||
| + | for a in 1:Points*3 loop | ||
| + | G[x*3,a]:=0; | ||
| + | G[x*3,x*3]:=1; | ||
| + | end for; | ||
| + | end if; | ||
| + | end for; | ||
| + | //Solving displacement | ||
| + | displacement:=Modelica.Math.Matrices.solve(G,F); | ||
| + | //Solving reaction | ||
| + | reaction:=(G_star*displacement)-F; | ||
| + | //Eliminating float error | ||
| + | for i in 1:N loop | ||
| + | reaction[i]:=if abs(reaction[i])<=err then 0 else reaction[i]; | ||
| + | displacement[i]:=if abs(displacement[i])<=err then 0 else displacement[i]; | ||
| + | end for; | ||
| + | //Checking Force | ||
| + | check[1]:=sum({reaction[i] for i in (1:3:(N-2))})+sum({F[i] for i in (1:3:(N-2))}); | ||
| + | check[2]:=sum({reaction[i] for i in (2:3:(N-1))})+sum({F[i] for i in (2:3:(N-1))}); | ||
| + | check[3]:=sum({reaction[i] for i in (3:3:N)})+sum({F[i] for i in (3:3:N)}); | ||
| + | for i in 1:3 loop | ||
| + | check[i] := if abs(check[i])<=ers then 0 else check[i]; | ||
| + | end for; | ||
| + | //Calculating stress in each truss | ||
| + | for i in 1:Trusses loop | ||
| + | for j in 1:3 loop | ||
| + | q1[j]:=P[C[i,1],j]; | ||
| + | q2[j]:=P[C[i,2],j]; | ||
| + | dis[j]:=abs(displacement[3*(C[i,1]-1)+j]-displacement[3*(C[i,2]-1)+j]); | ||
| + | end for; | ||
| + | //Solving Matrix | ||
| + | L:=Modelica.Math.Vectors.length(q2-q1); | ||
| + | cx:=(q2[1]-q1[1])/L; | ||
| + | cy:=(q2[2]-q1[2])/L; | ||
| + | cz:=(q2[3]-q1[3])/L; | ||
| + | X:=(Elas/L)*[cx^2,cx*cy,cx*cz; | ||
| + | cy*cx,cy^2,cy*cz; | ||
| + | cz*cx,cz*cy,cz^2]; | ||
| + | Str:=(X*dis); | ||
| + | stress1[i]:=Modelica.Math.Vectors.length(Str); | ||
| + | end for; | ||
| + | //Safety factor | ||
| + | for i in 1:Trusses loop | ||
| + | if stress1[i]>0 then | ||
| + | safety[i]:=Yield/stress1[i]; | ||
| + | else | ||
| + | safety[i]:=0; | ||
| + | end if; | ||
| + | end for; | ||
| + | end TugasBesar_Trusses_3d_Safety; | ||
| + | |||
| + | |||
| + | dan didapati hasil berikut dari simulasi tersebut | ||
| + | |||
| + | didapatkan displacement seperti ini | ||
| + | |||
| + | [[File:displacement_rayhan.jpg|720px|center]] | ||
| + | |||
| + | kemudian reaction force sebagai berikut | ||
| + | |||
| + | [[File:reaction_tubes_rayhan.jpg|720px|center]] | ||
| + | |||
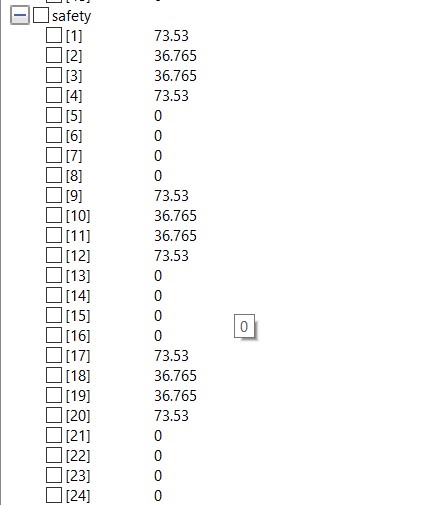
| + | safety factor | ||
| + | |||
| + | [[File:safety_rayhan.jpg|720px|center]] | ||
| + | |||
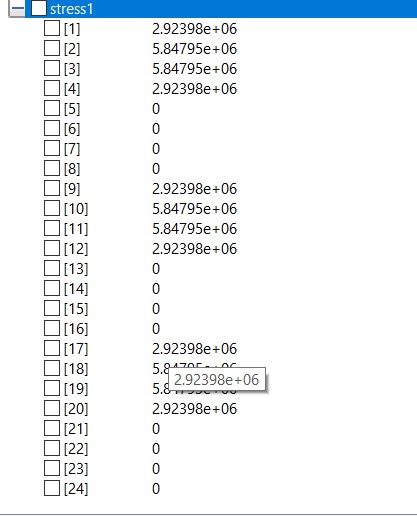
| + | stress | ||
| + | |||
| + | [[File:stress_tubes_rayhan.jpg|720px|center]] | ||
| + | |||
| + | |||
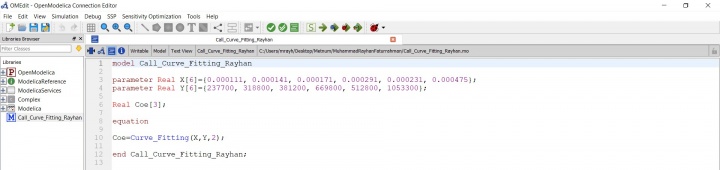
| + | '''Curve Fitting''' | ||
| + | |||
| + | Dalam mencari fitting cost/6m, saya menggunakan modelica untuk mencari koefisien persamaannya dengan metode curve fitting. Berikut adalah koding modelica yang saya gunakan untuk curve fitting. | ||
| + | |||
| + | |||
| + | [[File:kode_fittingcost_rayhan.jpg|720px|center]] | ||
| + | |||
| + | |||
| + | [[File:Fittingcost_rayhan.jpg|720px|center]] | ||
| + | |||
| + | == Jawaban UAS (13 Januari 2021) == | ||
| + | |||
| + | '''LEMBAR JAWABAN''' | ||
| + | |||
| + | [[File:uasrayhan1.jpg|720px|center]] | ||
| + | |||
| + | [[File:uasrayhan2.jpg|720px|center]] | ||
| + | |||
| + | [[File:uasrayhan3.jpg|720px|center]] | ||
| + | |||
| + | |||
| + | '''SCRIPT YANG DIGUNAKAN''' | ||
| + | |||
| + | model UAS_MuhammadRayhan | ||
| + | //define initial variable | ||
| + | parameter Integer Points = size(P, 1); | ||
| + | //Number of Points | ||
| + | parameter Integer Trusses = size(C, 1); | ||
| + | //Number of Trusses | ||
| + | parameter Real Yield = 196e6; | ||
| + | //Yield Strength (Pa) | ||
| + | parameter Real Area = 40; | ||
| + | //Area L Profile | ||
| + | parameter Real Elas = 30e6; | ||
| + | //define connection | ||
| + | parameter Integer C[:, 2] = [1, 2; 1, 3; 1, 4]; | ||
| + | //define coordinates (please put orderly) | ||
| + | parameter Real P[:, 6] = [0, 0, 0, 1, 1, 1; //1 | ||
| + | 6.5, 6.5, 36.5, 1, 1, 1; //2 | ||
| + | 6.5, 6.5, 36.5, 1, 1, 1; //3 | ||
| + | 6.5, 6.5, 36.5, 1, 1, 1]; //4 | ||
| + | //define external force (please put orderly) | ||
| + | parameter Real F[Points * 3] = {0,0,0,0,3708180,0,0,3708180,0,0,3708180,0}; | ||
| + | //solution | ||
| + | Real displacement[N], reaction[N]; | ||
| + | Real check[3]; | ||
| + | Real stress1[Trusses]; | ||
| + | Real safety[Trusses]; | ||
| + | Real dis[3]; | ||
| + | Real Str[3]; | ||
| + | protected | ||
| + | parameter Integer N = 3 * Points; | ||
| + | Real q1[3], q2[3], g[N, N], G[N, N], G_star[N, N], id[N, N] = identity(N), cx, cy, cz, L, X[3, 3]; | ||
| + | Real err = 10e-10, ers = 10e-4; | ||
| + | algorithm | ||
| + | //Creating Global Matrix | ||
| + | G := id; | ||
| + | for i in 1:Trusses loop | ||
| + | for j in 1:3 loop | ||
| + | q1[j] := P[C[i, 1], j]; | ||
| + | q2[j] := P[C[i, 2], j]; | ||
| + | end for; | ||
| + | L := Modelica.Math.Vectors.length(q2 - q1); | ||
| + | cx := (q2[1] - q1[1]) / L; | ||
| + | cy := (q2[2] - q1[2]) / L; | ||
| + | cz := (q2[3] - q1[3]) / L; | ||
| + | X := Area * Elas / L * [cx ^ 2, cx * cy, cx * cz; cy * cx, cy ^ 2, cy * cz; cz * cx, cz * cy, cz ^ 2]; | ||
| + | g := zeros(N, N); | ||
| + | for m, n in 1:3 loop | ||
| + | g[3 * (C[i, 1] - 1) + m, 3 * (C[i, 1] - 1) + n] := X[m, n]; | ||
| + | g[3 * (C[i, 2] - 1) + m, 3 * (C[i, 2] - 1) + n] := X[m, n]; | ||
| + | g[3 * (C[i, 2] - 1) + m, 3 * (C[i, 1] - 1) + n] := -X[m, n]; | ||
| + | g[3 * (C[i, 1] - 1) + m, 3 * (C[i, 2] - 1) + n] := -X[m, n]; | ||
| + | end for; | ||
| + | G_star := G + g; | ||
| + | G := G_star; | ||
| + | end for; | ||
| + | //Solving Matrix | ||
| + | //Transforming to global matrix | ||
| + | //Implementing boundary | ||
| + | for x in 1:Points loop | ||
| + | if P[x, 4] <> 0 then | ||
| + | for a in 1:Points * 3 loop | ||
| + | G[x * 3 - 2, a] := 0; | ||
| + | G[x * 3 - 2, x * 3 - 2] := 1; | ||
| + | end for; | ||
| + | end if; | ||
| + | if P[x, 5] <> 0 then | ||
| + | for a in 1:Points * 3 loop | ||
| + | G[x * 3 - 1, a] := 0; | ||
| + | G[x * 3 - 1, x * 3 - 1] := 1; | ||
| + | end for; | ||
| + | end if; | ||
| + | if P[x, 6] <> 0 then | ||
| + | for a in 1:Points * 3 loop | ||
| + | G[x * 3, a] := 0; | ||
| + | G[x * 3, x * 3] := 1; | ||
| + | end for; | ||
| + | end if; | ||
| + | end for; | ||
| + | //Solving displacement | ||
| + | displacement := Modelica.Math.Matrices.solve(G, F); | ||
| + | //Solving reaction | ||
| + | reaction := G_star * displacement - F; | ||
| + | //Eliminating float error | ||
| + | for i in 1:N loop | ||
| + | reaction[i] := if abs(reaction[i]) <= err then 0 else reaction[i]; | ||
| + | displacement[i] := if abs(displacement[i]) <= err then 0 else displacement[i]; | ||
| + | end for; | ||
| + | //Checking Force | ||
| + | check[1] := sum({reaction[i] for i in 1:3:N - 2}) + sum({F[i] for i in 1:3:N - 2}); | ||
| + | check[2] := sum({reaction[i] for i in 2:3:N - 1}) + sum({F[i] for i in 2:3:N - 1}); | ||
| + | check[3] := sum({reaction[i] for i in 3:3:N}) + sum({F[i] for i in 3:3:N}); | ||
| + | for i in 1:3 loop | ||
| + | check[i] := if abs(check[i]) <= ers then 0 else check[i]; | ||
| + | end for; | ||
| + | //Calculating stress in each truss | ||
| + | for i in 1:Trusses loop | ||
| + | for j in 1:3 loop | ||
| + | q1[j] := P[C[i, 1], j]; | ||
| + | q2[j] := P[C[i, 2], j]; | ||
| + | dis[j] := abs(displacement[3 * (C[i, 1] - 1) + j] - displacement[3 * (C[i, 2] - 1) + j]); | ||
| + | end for; | ||
| + | L := Modelica.Math.Vectors.length(q2 - q1); | ||
| + | cx := (q2[1] - q1[1]) / L; | ||
| + | cy := (q2[2] - q1[2]) / L; | ||
| + | cz := (q2[3] - q1[3]) / L; | ||
| + | X := Elas / L * [cx ^ 2, cx * cy, cx * cz; cy * cx, cy ^ 2, cy * cz; cz * cx, cz * cy, cz ^ 2]; | ||
| + | Str := X * dis; | ||
| + | stress1[i] := Modelica.Math.Vectors.length(Str); | ||
| + | end for; | ||
| + | //Solving Matrix | ||
| + | //Safety factor | ||
| + | for i in 1:Trusses loop | ||
| + | if stress1[i] > 0 then | ||
| + | safety[i] := Yield / stress1[i]; | ||
| + | else | ||
| + | safety[i] := 0; | ||
| + | end if; | ||
| + | end for; | ||
| + | end UAS_MuhammadRayhan; | ||
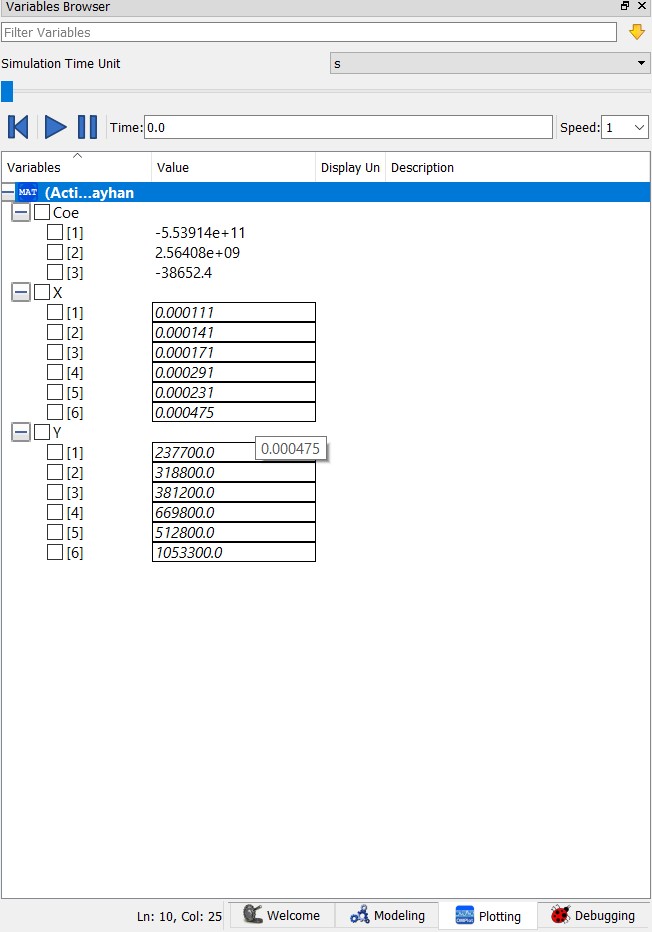
| + | '''OUTPUT PADA OpenModelica''' | ||
| − | + | [[File:hasiluasrayhan.jpg|720px|center]] | |
Latest revision as of 21:12, 14 January 2021
Contents
BIODATA
Nama : Muhammad Rayhan Faturrahman
NPM : 1906301255
Jurusan : Teknik Mesin
Angkatan : 2019
Hobi : Kulineran
Jargon : Selaras Dalam Dinamika
Selamat sore teman-teman, perkenalkan nama saya Fatur, teman-teman saya di Departemen Teknik Mesin biasa memanggil saya bob. Saya memilih jurusan Teknik Mesin karena terisnpirasi dari saudara saya yang seorang process engineer, saya sering memerhatikannya melakukan simulasi desain pabrik dan menurut saya itu merupakan pekerjaan yang seru sekaligus menantang, berangkat dari sana saya memilih untuk menjadi seorang engineer juga tapi pada disiplin yang berbeda dengan harapan nantinya kami dapat membuat perusahaan konsultan sendiri.
Komputasi
Saya memiliki ketertarikan pada dunia komputasi sejak awal memasuki perkuliahan teknik, namun masih muncul banyak pertanyaan "harus mulai belajar dari mana?" dan pertanyaan lain sebagainya, alasan inilah yang semata-mata menjadi pemicu saya untuk mencoba mengambil mata kuliah metode numerik di semester 3 ini.
Perkuliahan Minggu Pertama (Rabu, 11 November 2020)
Pada pertemuan pertama yang diisi oleh Bapak Dr. Ir. Ahmad Indra Siswantara. Mahasiswa dijelaskan mengenai esensi atau tujuan pembelajaran metode numerik, yaitu:
1. Memahami konsep-konsep dan prinsip-prinsip dasar dalam metode numerik seperti:Persamaan algoritma, aljabar, pencocokan kurva, persamaan diferensial parsial, dan lainnya
2. Mengerti dan mampu menerapkan pemahaman atau aplikasi terhadap konsep metode numerik
3. Mampu menerapkan metode numerik dalam hal terkait persoalan keteknikan
4. Mendapat added value (nilai tambah)/adab sehingga mahasiswa menjadi orang yang memiliki budi pekerti yang baik
Selain empat poin yang telah disebutkan, Mahasiswa juga mendapatkan pelajaran terkait penggunaan aplikasi OpenModelica. Dimana Mahasiswa dijelaskan dengan sangat sistematis mengenai penggunaan sederhana OpenModelica.
Untuk memenuhi tugas minggu pertama terkait pengantar aplikasi OpenModelica, saya telah membuat model matematis untuk menyelesaikan problem fisika dengan tema Hukum Kekekalan Momentum
LINK TUGAS : https://youtu.be/JjA5Otn82pY
Perkuliahan Minggu Ke-2 (18 November 2020)
Catatan Penting
Menjadi pribadi yang lebih baik dari hari kemarin, bahwa menjadi manusia haruslah terus berkembang setiap harinya, merupakan pesan moral sekaligus pelajaran hidup pembuka kuliah yang disampaikan Pak Dai pada perkuliahan kali ini.
Beberapa hal penting yang pahami pada perkuliahan kali ini adalah:
- Metode Numerik sangat mengassist perhitungan di bidang engineering, faktor cepat dan akurat memegang peranan penting dalam penggunaannya di lapangan.
- Efisiensi adalah alasan utama mengapa kita sebagai calon engineer semestinya memiliki keahlian metode numerik, terutama pemodelan/
- Berbicara mengenai pemodelan, Pak Dai sempat membahas mengenai mengapa pada perkuliahan metode numerik beliau memilih menggunakan OpenModellica dibanding bahasa pemrograman lain, seperti Fortran dan C, beliau menekankan bahwa Modelica merupakan bahasa pemodelan, hal ini menjadikannya tools yang mudah dioperasikan oleh kami yang berlatar belakang bukan dari Computer Science.
- Selain itu, Modelica adalah aplikasi yang bersifat Open-Source alias gratis, sehingga mahasiswa tidak perlu mengeluarkan biaya sepeserpun.
Tugas Minggu Ke-2
Pak Dai menugaskan kami untuk membuat sebuah class dan fungsi panggil. Disini saya akan mengerjakan sebuah soal Aljabar Linier dan mencari penyelesaiannya menggunakan perintah Modelica.Math.Matrices.solve
Perkuliahan Minggu Ke-3 (25 November 2020)
Pada perkuliahan ini Pak Dai menjelaskan tentang beberapa pengaplikasian metode numerik di dunia engineering, antara lain
- Computational Fluid Dynamic
- Metode Stokastik
- Finite Element Analysis
Sebelum membahas tentang aplikasi metode numerik pada permasalahan engineering, kita mereview tugas minggu ke-2 mengenai class dan function panggil, kemudian ternyata satu kelas masih kurang mencapai target Pak Dai, karena masih menggunakan function yang sudah disediakan oleh OpenModelica dan bukan membuat sendiri. Akhirnya cristo mencoba menjelaskan penyelesaian yang sudah dia buat seperti di bawah ini :
// Gauss-Jordan Algorithm // Transforms input matrix A into reduced row echelon form matrix B // Christopher S.E. November 2020 input Real [:,:] A; // An augmented matrix of m*n output Real [:,:] B; // Output matrix in reduced row echelon form // Local variables
protected Integer h = 1; // Initialize pivot row Integer k = 1; // Initialize pivot column Integer m = size(A,1); // Number of rows in matrix Integer n = size(A,2); // Number of columns in matrix Integer c = 0; // Index counter Integer max_row; // Row index of max number in pivot column
Real [:] pivot_column; // Vector containing pivot column data Real [:] pivot_row; // Vector containing backwards pivot row data Real [:,:] temp_array; // Stores matrix data when switching rows Real r; // Ratio value used for row operations
// Limit to handle floating point errors Real float_error = 10e-10;
algorithm
// Transfer input matrix A into variable B B := A;
while h <= m and k <= n loop
// Dealing with floating point errors
for i in 1:m loop
for j in 1:n loop
if abs(B[i,j]) <= float_error then
B[i,j] := 0;
end if;
end for;
end for;
// Finding the pivot
pivot_column := {B[i,h] for i in h:m};
// Get position index of lowest row with greatest pivot number
c:= h-1;
for element in pivot_column loop
c := c+1;
if abs(element) == max(abs(pivot_column)) then
max_row := c;
end if;
end for;
// No pivot in this column, move on to next column
if B[max_row, k] == 0 then
k := k+1;
else
// Swap Rows h <-> max_row
temp_array := B;
temp_array[h] := B[max_row];
temp_array[max_row] := B[h];
B:= temp_array;
// Divide pivot row by pivot number
B[h] := B[h]/B[h,k];
// For all rows below the pivot
for i in (h+1):m loop
// Store the ratio of the row to the pivot
r := B[i,k] / B[h,k];
// Set lower part of pivot column to zero
B[i,k] := 0;
// Operations on the remaining row elements
for j in (k+1):n loop
B[i,j] := B[i,j] - B[h,j] * r;
end for;
end for;
// Move on to next pivot row and column
h := h+1;
k := k+1;
end if;
end while;
// The matrix is now in row echelon form
// Set values of (h,k) to (m,n) h := m; k := n;
while h >= 1 and k >=1 loop
// Dealing with floating point errors
for i in 1:m loop
for j in 1:n loop
if abs(B[i,j]) <= float_error then
B[i,j] := 0;
end if;
end for;
end for;
// Finding the pivot
pivot_row := {B[h,i] for i in 1:k};
// Get position index k of pivot
c := 0;
for element in pivot_row loop
c := c+1;
if element <> 0 then
break;
end if;
end for;
k := c;
// No pivot in this row, move on to next row
if B[h, k] == 0 then
Bold text h := h-1;
else
// Perform row operations
for i in 1:(h-1) loop
r := B[i,k];
B[i] := B[i] - B[h] * r;
end for;
// Move on to next pivot row and column
h := h-1;
k := k-1;
end if;
end while;
// The matrix is now in reduced row echelon form
end GaussJordan;
Tugas Minggu Ke-3
Persamaan
class Trusses_Tugas3_MRayhanFatur
parameter Integer N=8; //Global matrice = 2*points connected
parameter Real A=0.001; //Area m2
parameter Real E=200e9; //Pa
Real G[N,N]; //global
Real Ginitial[N,N]; //global
Real Sol[N]; //global dispplacement
Real X[N]={0,0,-1035.2762,-3863.7033,0,0,-1035.2762,-3863.7033};
Real R[N]; //global reaction force
Real SolMat[N,1];
Real XMat[N,1];
//boundary condition
Integer b1=1;
Integer b2=3;
//truss 1
parameter Real X1=0; //degree between truss
Real k1=A*E/1;
Real K1[4,4]; //stiffness matrice
Integer p1a=1;
Integer p1b=2;
Real G1[N,N];
//truss 2
parameter Real X2=0; //degree between truss
Real k2=A*E/1;
Real K2[4,4]; //stiffness matrice
Integer p2a=2;
Integer p2b=3;
Real G2[N,N];
//truss 3
parameter Real X3=90; //degree between truss
Real k3=A*E/1.25;
Real K3[4,4]; //stiffness matrice
Integer p3a=2;
Integer p3b=4;
Real G3[N,N];
//truss 4
parameter Real X4=90+38.6598; //degree between truss
Real k4=A*E/1.6;
Real K4[4,4]; //stiffness matrice
Integer p4a=1;
Integer p4b=4;
Real G4[N,N];
//truss 5
parameter Real X5=90-38.6598; //degree between truss
Real k5=A*E/1.6;
Real K5[4,4]; //stiffness matrice
Integer p5a=3;
Integer p5b=4;
Real G5[N,N];
/*
for each truss, please ensure pXa is lower then pXb (X represents truss element number)
*/
algorithm
//creating global matrice
K1:=Stiffness_Matrices(X1);
G1:=k1*Local_Global(K1,N,p1a,p1b);
K2:=Stiffness_Matrices(X2);
G2:=k2*Local_Global(K2,N,p2a,p2b);
K3:=Stiffness_Matrices(X3);
G3:=k3*Local_Global(K3,N,p3a,p3b);
K4:=Stiffness_Matrices(X4);
G4:=k4*Local_Global(K4,N,p4a,p4b);
K5:=Stiffness_Matrices(X5);
G5:=k5*Local_Global(K5,N,p5a,p5b);
G:=G1+G2+G3+G4+G5;
Ginitial:=G;
//implementing boundary condition
for i in 1:N loop
G[2*b1-1,i]:=0;
G[2*b1,i]:=0;
G[2*b2-1,i]:=0;
G[2*b2,i]:=0;
end for;
G[2*b1-1,2*b1-1]:=1;
G[2*b1,2*b1]:=1;
G[2*b2-1,2*b2-1]:=1;
G[2*b2,2*b2]:=1;
//solving displacement
Sol:=Gauss_Jordan(N,G,X);
//solving reaction force
SolMat:=matrix(Sol);
XMat:=matrix(X);
R:=Reaction_Trusses(N,Ginitial,SolMat,XMat);
end Trusses_Tugas3_MRayhanFatur;
Fungsi Panggil Yang Digunakan
|
Matrice Transformation function Stiffness_Matrices
input Real A;
Real Y;
output Real X[4,4];
Real float_error = 10e-10;
final constant Real pi=2*Modelica.Math.asin(1.0);
algorithm
Y:=A/180*pi;
X:=[(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y);
Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2;
-(Modelica.Math.cos(Y))^2,-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.cos(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y);
-Modelica.Math.cos(Y)*Modelica.Math.sin(Y),-(Modelica.Math.sin(Y))^2,Modelica.Math.cos(Y)*Modelica.Math.sin(Y),(Modelica.Math.sin(Y))^2];
for i in 1:4 loop
for j in 1:4 loop
if abs(X[i,j]) <= float_error then
X[i,j] := 0;
end if;
end for;
end for;
end Stiffness_Matrices;
|
Global Element Matrice function Local_Global
input Real Y[4,4];
input Integer B;
input Integer p1;
input Integer p2;
output Real G[B,B];
algorithm
for i in 1:B loop
for j in 1:B loop
G[i,j]:=0;
end for;
end for;
G[2*p1,2*p1]:=Y[2,2];
G[2*p1-1,2*p1-1]:=Y[1,1];
G[2*p1,2*p1-1]:=Y[2,1];
G[2*p1-1,2*p1]:=Y[1,2];
G[2*p2,2*p2]:=Y[4,4];
G[2*p2-1,2*p2-1]:=Y[3,3];
G[2*p2,2*p2-1]:=Y[4,3];
G[2*p2-1,2*p2]:=Y[3,4];
G[2*p2,2*p1]:=Y[4,2];
G[2*p2-1,2*p1-1]:=Y[3,1];
G[2*p2,2*p1-1]:=Y[4,1];
G[2*p2-1,2*p1]:=Y[3,2];
G[2*p1,2*p2]:=Y[2,4];
G[2*p1-1,2*p2-1]:=Y[1,3];
G[2*p1,2*p2-1]:=Y[2,3];
G[2*p1-1,2*p2]:=Y[1,4];
end Local_Global;
|
Reaction Matrice Equation function Reaction_Trusses input Integer N; input Real A[N,N]; input Real B[N,1]; input Real C[N,1]; Real X[N,1]; output Real Sol[N]; Real float_error = 10e-10; algorithm X:=A*B-C; for i in 1:N loop if abs(X[i,1]) <= float_error then X[i,1] := 0; end if; end for; for i in 1:N loop Sol[i]:=X[i,1]; end for; end Reaction_Trusses; |
Gauss Jordan
|
function Gauss_Jordan input Integer N; input Real A[N,N]; input Real B[N]; output Real X[N]; Real float_error = 10e-10; algorithm X:=Modelica.Math.Matrices.solve(A,B); for i in 1:N loop if abs(X[i]) <= float_error then X[i] := 0; end if; end for; end Gauss_Jordan;
KUIS Flowchart Minggu Ke-4Pada minggu ke-4 ini, perkuliahan terhalang oleh libur karena adanya Pilkada, sehingga Pak Dai memberikan tugas untuk mengerjakan soal di bawah ini:
input Real [:,9] inisiasi_mat; output Real [size(inisiasi_mat,1),6,6] Ke_mat; protected Real cos_x; Real cos_y; Real cos_z; Real [6] StiffTrig; Real [6,6] StiffTrans; Real [size(inisiasi_mat,1)] k_vec; algorithm k_vec := {(inisiasi_mat[i,7] * inisiasi_mat[i,8] / inisiasi_mat[i,9]) for i in 1:size(inisiasi_mat,1)};
// Finding stiffness matrix of each element member for i in 1:size(inisiasi_mat,1) loop // Clearing the matrices StiffTrig := zeros(6); StiffTrans := zeros(6,6); // Converting degrees to radians cos_x := inisiasi_mat[i,4]; cos_y := inisiasi_mat[i,5]; cos_z := inisiasi_mat[i,6]; // {cos^2, sin^2, sincos}
StiffTrig := {(cos_x)^2,
(cos_y)^2,
(cos_z)^2,
(cos_x*cos_y),
(cos_x*cos_z),
(cos_y*cos_z)};
// Construct stiffness transformation matrix
StiffTrans := [ StiffTrig[1], StiffTrig[4], StiffTrig[5], -1*StiffTrig[1], -1*StiffTrig[4], -1*StiffTrig[5];
StiffTrig[4], StiffTrig[2], StiffTrig[6], -1*StiffTrig[4], -1*StiffTrig[2], -1*StiffTrig[6];
StiffTrig[5], StiffTrig[6], StiffTrig[3], -1*StiffTrig[5], -1*StiffTrig[6], -1*StiffTrig[3];
-1*StiffTrig[1], -1*StiffTrig[4], -1*StiffTrig[5], StiffTrig[1], StiffTrig[4], StiffTrig[5];
-1*StiffTrig[4], -1*StiffTrig[2], -1*StiffTrig[6], StiffTrig[4], StiffTrig[2], StiffTrig[6];
-1*StiffTrig[5], -1*StiffTrig[6], -1*StiffTrig[3], StiffTrig[5], StiffTrig[6], StiffTrig[3]];
// Multiply in stiffness constant of element, add final stiffness matrix to Ke_mat
for m in 1:6 loop
for n in 1:6 loop
Ke_mat[i,m,n] := k_vec[i] * StiffTrans[m,n];
end for;
end for;
end for; end StiffnessMatrixElement;
|
|
function StiffnessMatrixGlobal input Integer [:,2] n; input Integer x; input Integer y; input Real [y,6,6] Ke_mat; output Real [y,x,x] Kg_mat; algorithm for i in 1:y loop
for a in 1:x loop
for b in 1:x loop
Kg_mat[i,a,b]:=0;
end for;
end for;
end for;
for i in 1:y loop Kg_mat[i,3*n[i,1],3*n[i,1]]:=Ke_mat[i,3,3]; Kg_mat[i,3*n[i,1],3*n[i,1]-1]:=Ke_mat[i,3,2]; Kg_mat[i,3*n[i,1],3*n[i,1]-2]:=Ke_mat[i,3,1]; Kg_mat[i,3*n[i,1]-1,3*n[i,1]]:=Ke_mat[i,2,3]; Kg_mat[i,3*n[i,1]-1,3*n[i,1]-1]:=Ke_mat[i,2,2]; Kg_mat[i,3*n[i,1]-1,3*n[i,1]-2]:=Ke_mat[i,2,1]; Kg_mat[i,3*n[i,1]-2,3*n[i,1]]:=Ke_mat[i,1,3]; Kg_mat[i,3*n[i,1]-2,3*n[i,1]-1]:=Ke_mat[i,1,2]; Kg_mat[i,3*n[i,1]-2,3*n[i,1]-2]:=Ke_mat[i,1,1]; Kg_mat[i,3*n[i,2],3*n[i,2]]:=Ke_mat[i,6,6]; Kg_mat[i,3*n[i,2],3*n[i,2]-1]:=Ke_mat[i,6,5]; Kg_mat[i,3*n[i,2],3*n[i,2]-2]:=Ke_mat[i,6,4]; Kg_mat[i,3*n[i,2]-1,3*n[i,2]]:=Ke_mat[i,5,6]; Kg_mat[i,3*n[i,2]-1,3*n[i,2]-1]:=Ke_mat[i,5,5]; Kg_mat[i,3*n[i,2]-1,3*n[i,2]-2]:=Ke_mat[i,5,4]; Kg_mat[i,3*n[i,2]-2,3*n[i,2]]:=Ke_mat[i,4,6]; Kg_mat[i,3*n[i,2]-2,3*n[i,2]-1]:=Ke_mat[i,4,5]; Kg_mat[i,3*n[i,2]-2,3*n[i,2]-2]:=Ke_mat[i,4,4]; Kg_mat[i,3*n[i,2],3*n[i,1]]:=Ke_mat[i,6,3]; Kg_mat[i,3*n[i,2],3*n[i,1]-1]:=Ke_mat[i,6,2]; Kg_mat[i,3*n[i,2],3*n[i,1]-2]:=Ke_mat[i,6,1]; Kg_mat[i,3*n[i,2]-1,3*n[i,1]]:=Ke_mat[i,5,3]; Kg_mat[i,3*n[i,2]-1,3*n[i,1]-1]:=Ke_mat[i,5,2]; Kg_mat[i,3*n[i,2]-1,3*n[i,1]-2]:=Ke_mat[i,5,1]; Kg_mat[i,3*n[i,2]-2,3*n[i,1]]:=Ke_mat[i,4,3]; Kg_mat[i,3*n[i,2]-2,3*n[i,1]-1]:=Ke_mat[i,4,2]; Kg_mat[i,3*n[i,2]-2,3*n[i,1]-2]:=Ke_mat[i,4,1]; Kg_mat[i,3*n[i,1],3*n[i,2]]:=Ke_mat[i,3,6]; Kg_mat[i,3*n[i,1],3*n[i,2]-1]:=Ke_mat[i,3,5]; Kg_mat[i,3*n[i,1],3*n[i,2]-2]:=Ke_mat[i,3,4]; Kg_mat[i,3*n[i,1]-1,3*n[i,2]]:=Ke_mat[i,2,6]; Kg_mat[i,3*n[i,1]-1,3*n[i,2]-1]:=Ke_mat[i,2,5]; Kg_mat[i,3*n[i,1]-1,3*n[i,2]-2]:=Ke_mat[i,2,4]; Kg_mat[i,3*n[i,1]-2,3*n[i,2]]:=Ke_mat[i,1,6]; Kg_mat[i,3*n[i,1]-2,3*n[i,2]-1]:=Ke_mat[i,1,5]; Kg_mat[i,3*n[i,1]-2,3*n[i,2]-2]:=Ke_mat[i,1,4]; end for; end StiffnessMatrixGlobal;
Kuliah Minggu Ke-7 (23 Desember 2020)TUJUAN Kuliah kali ini kita melaksanakan kuis mengenai optimasi 3D Truss menggunakan class algoritma yang sebelumnya telah disusun pada kuliah minggu lalu. 3D Truss yang dimaksud seperti ini.
PENGERJAAN Diketahui: panjang= 0,6 m lebar= 0,75 m tinggi= 1,6 m F1= 2000 N F2= 1000 N kemudian diasumsikan -Beban akan terdistribusi hanya pada node (karena bersifat trusses). -Safety factor minimal bernilai 2. -Batas displacement 0,001 m sebelum buckling (pada truss paling atas). -Ketinggian trusses pada tiap lantai sama yaitu 0,6 m.
-Mencari harga untuk 5 ukuran batang dengan material yang sama yaitu SS304. -Mengitung nilai safety factor pada 5 ukuran batang dengan coding awal. -Membuat rasio antara safety factor dengan harga total. -Membuat persamaan antara rasio (safe/harga) dengan area dengan metode curve fitting. -Melakukan optimasi menggunakan metode golden section.
-Mencari harga untuk 3 jenis material dengan area penampang yang sama yaitu 171 mm^2. -Mengitung nilai safety factor pada 3 jenis batang dengan coding awal. -Membuat rasio antara safety factor dengan harga total. -Membuat persamaan antara rasio (safe/harga) dengan jenis material dengan metode curve fitting. -Melakukan optimasi menggunakan metode golden section.
Update Tugas BesarUntuk mencari reaction force, stress, dan safety factor, saya menggunakan script di bawah ini model TugasBesar_Trusses_3d_Safety //define initial variable parameter Integer Points=size(P,1); //Number of Points parameter Integer Trusses=size(C,1); //Number of Trusses parameter Real Yield=215e6; //Yield Strength (Pa) parameter Real Area=0.000171; //Area L Profile (Dimension=0.03, Thickness=0,003) (m2) parameter Real Elas=193e9; //Elasticity SS 304 (Pa) //define connection
parameter Integer C[:,2]=[1,5;
2,6;
3,7;
4,8;
5,6; //1st floor
6,7; //1st floor
7,8; //1st floor
5,8; //1st floor
5,9;
6,10;
7,11;
8,12;
9,10; //2nd floor
10,11;//2nd floor
11,12;//2nd floor
9,12; //2nd floor
9,13;
10,14;
11,15;
12,16;
13,14;//3rd floor
14,15;//3rd floor
15,16;//3rd floor
13,16];//3rd floor
//define coordinates (please put orderly)
parameter Real P[:,6]=[0.3,-0.375,0,1,1,1; //1
-0.3,-0.375,0,1,1,1; //2
-0.3,0.375,0,1,1,1; //3
0.3,0.375,0,1,1,1; //4
0.3,-0.375,0.6,0,0,0; //5
-0.3,-0.375,0.6,0,0,0; //6
-0.3,0.375,0.6,0,0,0; //7
0.3,0.375,0.6,0,0,0; //8
0.3,-0.375,1.2,0,0,0; //9
-0.3,-0.375,1.2,0,0,0; //10
-0.3,0.375,1.2,0,0,0; //11
0.3,0.375,1.2,0,0,0; //12
0.3,-0.375,1.8,0,0,0; //13
-0.3,-0.375,1.8,0,0,0; //14
-0.3,0.375,1.8,0,0,0; //15
0.3,0.375,1.8,0,0,0]; //16
//define external force (please put orderly)
parameter Real F[Points*3]={0,0,0,
0,0,0,
0,0,0,
0,0,0,
0,0,0,
0,0,0,
0,0,0,
0,0,0,
0,0,0,
0,0,0,
0,0,0,
0,0,0,
0,0,-500,
0,0,-1000,
0,0,-1000,
0,0,-500};
//solution
Real displacement[N], reaction[N];
Real check[3];
Real stress1[Trusses];
Real safety[Trusses];
Real dis[3];
Real Str[3];
protected
parameter Integer N=3*Points;
Real q1[3], q2[3], g[N,N], G[N,N], G_star[N,N], id[N,N]=identity(N), cx, cy, cz, L, X[3,3];
Real err=10e-10, ers=10e-4;
algorithm
//Creating Global Matrix
G:=id;
for i in 1:Trusses loop
for j in 1:3 loop
q1[j]:=P[C[i,1],j];
q2[j]:=P[C[i,2],j];
end for;
//Solving Matrix
L:=Modelica.Math.Vectors.length(q2-q1);
cx:=(q2[1]-q1[1])/L;
cy:=(q2[2]-q1[2])/L;
cz:=(q2[3]-q1[3])/L;
X:=(Area*Elas/L)*[cx^2,cx*cy,cx*cz;
cy*cx,cy^2,cy*cz;
cz*cx,cz*cy,cz^2];
//Transforming to global matrix
g:=zeros(N,N);
for m,n in 1:3 loop
g[3*(C[i,1]-1)+m,3*(C[i,1]-1)+n]:=X[m,n];
g[3*(C[i,2]-1)+m,3*(C[i,2]-1)+n]:=X[m,n];
g[3*(C[i,2]-1)+m,3*(C[i,1]-1)+n]:=-X[m,n];
g[3*(C[i,1]-1)+m,3*(C[i,2]-1)+n]:=-X[m,n];
end for;
G_star:=G+g;
G:=G_star;
end for;
//Implementing boundary
for x in 1:Points loop
if P[x,4] <> 0 then
for a in 1:Points*3 loop
G[(x*3)-2,a]:=0;
G[(x*3)-2,(x*3)-2]:=1;
end for;
end if;
if P[x,5] <> 0 then
for a in 1:Points*3 loop
G[(x*3)-1,a]:=0;
G[(x*3)-1,(x*3)-1]:=1;
end for;
end if;
if P[x,6] <> 0 then
for a in 1:Points*3 loop
G[x*3,a]:=0;
G[x*3,x*3]:=1;
end for;
end if;
end for;
//Solving displacement
displacement:=Modelica.Math.Matrices.solve(G,F);
//Solving reaction
reaction:=(G_star*displacement)-F;
//Eliminating float error
for i in 1:N loop
reaction[i]:=if abs(reaction[i])<=err then 0 else reaction[i];
displacement[i]:=if abs(displacement[i])<=err then 0 else displacement[i];
end for;
//Checking Force
check[1]:=sum({reaction[i] for i in (1:3:(N-2))})+sum({F[i] for i in (1:3:(N-2))});
check[2]:=sum({reaction[i] for i in (2:3:(N-1))})+sum({F[i] for i in (2:3:(N-1))});
check[3]:=sum({reaction[i] for i in (3:3:N)})+sum({F[i] for i in (3:3:N)});
for i in 1:3 loop
check[i] := if abs(check[i])<=ers then 0 else check[i];
end for;
//Calculating stress in each truss
for i in 1:Trusses loop
for j in 1:3 loop
q1[j]:=P[C[i,1],j];
q2[j]:=P[C[i,2],j];
dis[j]:=abs(displacement[3*(C[i,1]-1)+j]-displacement[3*(C[i,2]-1)+j]);
end for;
//Solving Matrix
L:=Modelica.Math.Vectors.length(q2-q1);
cx:=(q2[1]-q1[1])/L;
cy:=(q2[2]-q1[2])/L;
cz:=(q2[3]-q1[3])/L;
X:=(Elas/L)*[cx^2,cx*cy,cx*cz;
cy*cx,cy^2,cy*cz;
cz*cx,cz*cy,cz^2];
Str:=(X*dis);
stress1[i]:=Modelica.Math.Vectors.length(Str);
end for;
//Safety factor
for i in 1:Trusses loop
if stress1[i]>0 then
safety[i]:=Yield/stress1[i];
else
safety[i]:=0;
end if;
end for;
end TugasBesar_Trusses_3d_Safety;
didapatkan displacement seperti ini kemudian reaction force sebagai berikut safety factor stress
Dalam mencari fitting cost/6m, saya menggunakan modelica untuk mencari koefisien persamaannya dengan metode curve fitting. Berikut adalah koding modelica yang saya gunakan untuk curve fitting.
Jawaban UAS (13 Januari 2021)LEMBAR JAWABAN
model UAS_MuhammadRayhan
//define initial variable
parameter Integer Points = size(P, 1);
//Number of Points
parameter Integer Trusses = size(C, 1);
//Number of Trusses
parameter Real Yield = 196e6;
//Yield Strength (Pa)
parameter Real Area = 40;
//Area L Profile
parameter Real Elas = 30e6;
//define connection
parameter Integer C[:, 2] = [1, 2; 1, 3; 1, 4];
//define coordinates (please put orderly)
parameter Real P[:, 6] = [0, 0, 0, 1, 1, 1; //1
6.5, 6.5, 36.5, 1, 1, 1; //2
6.5, 6.5, 36.5, 1, 1, 1; //3
6.5, 6.5, 36.5, 1, 1, 1]; //4
//define external force (please put orderly)
parameter Real F[Points * 3] = {0,0,0,0,3708180,0,0,3708180,0,0,3708180,0};
//solution
Real displacement[N], reaction[N];
Real check[3];
Real stress1[Trusses];
Real safety[Trusses];
Real dis[3];
Real Str[3];
protected
parameter Integer N = 3 * Points;
Real q1[3], q2[3], g[N, N], G[N, N], G_star[N, N], id[N, N] = identity(N), cx, cy, cz, L, X[3, 3];
Real err = 10e-10, ers = 10e-4;
algorithm
//Creating Global Matrix
G := id;
for i in 1:Trusses loop
for j in 1:3 loop
q1[j] := P[C[i, 1], j];
q2[j] := P[C[i, 2], j];
end for;
L := Modelica.Math.Vectors.length(q2 - q1);
cx := (q2[1] - q1[1]) / L;
cy := (q2[2] - q1[2]) / L;
cz := (q2[3] - q1[3]) / L;
X := Area * Elas / L * [cx ^ 2, cx * cy, cx * cz; cy * cx, cy ^ 2, cy * cz; cz * cx, cz * cy, cz ^ 2];
g := zeros(N, N);
for m, n in 1:3 loop
g[3 * (C[i, 1] - 1) + m, 3 * (C[i, 1] - 1) + n] := X[m, n];
g[3 * (C[i, 2] - 1) + m, 3 * (C[i, 2] - 1) + n] := X[m, n];
g[3 * (C[i, 2] - 1) + m, 3 * (C[i, 1] - 1) + n] := -X[m, n];
g[3 * (C[i, 1] - 1) + m, 3 * (C[i, 2] - 1) + n] := -X[m, n];
end for;
G_star := G + g;
G := G_star;
end for;
//Solving Matrix
//Transforming to global matrix
//Implementing boundary
for x in 1:Points loop
if P[x, 4] <> 0 then
for a in 1:Points * 3 loop
G[x * 3 - 2, a] := 0;
G[x * 3 - 2, x * 3 - 2] := 1;
end for;
end if;
if P[x, 5] <> 0 then
for a in 1:Points * 3 loop
G[x * 3 - 1, a] := 0;
G[x * 3 - 1, x * 3 - 1] := 1;
end for;
end if;
if P[x, 6] <> 0 then
for a in 1:Points * 3 loop
G[x * 3, a] := 0;
G[x * 3, x * 3] := 1;
end for;
end if;
end for;
//Solving displacement
displacement := Modelica.Math.Matrices.solve(G, F);
//Solving reaction
reaction := G_star * displacement - F;
//Eliminating float error
for i in 1:N loop
reaction[i] := if abs(reaction[i]) <= err then 0 else reaction[i];
displacement[i] := if abs(displacement[i]) <= err then 0 else displacement[i];
end for;
//Checking Force
check[1] := sum({reaction[i] for i in 1:3:N - 2}) + sum({F[i] for i in 1:3:N - 2});
check[2] := sum({reaction[i] for i in 2:3:N - 1}) + sum({F[i] for i in 2:3:N - 1});
check[3] := sum({reaction[i] for i in 3:3:N}) + sum({F[i] for i in 3:3:N});
for i in 1:3 loop
check[i] := if abs(check[i]) <= ers then 0 else check[i];
end for;
//Calculating stress in each truss
for i in 1:Trusses loop
for j in 1:3 loop
q1[j] := P[C[i, 1], j];
q2[j] := P[C[i, 2], j];
dis[j] := abs(displacement[3 * (C[i, 1] - 1) + j] - displacement[3 * (C[i, 2] - 1) + j]);
end for;
L := Modelica.Math.Vectors.length(q2 - q1);
cx := (q2[1] - q1[1]) / L;
cy := (q2[2] - q1[2]) / L;
cz := (q2[3] - q1[3]) / L;
X := Elas / L * [cx ^ 2, cx * cy, cx * cz; cy * cx, cy ^ 2, cy * cz; cz * cx, cz * cy, cz ^ 2];
Str := X * dis;
stress1[i] := Modelica.Math.Vectors.length(Str);
end for;
//Solving Matrix
//Safety factor
for i in 1:Trusses loop
if stress1[i] > 0 then
safety[i] := Yield / stress1[i];
else
safety[i] := 0;
end if;
end for;
end UAS_MuhammadRayhan;
OUTPUT PADA OpenModelica |