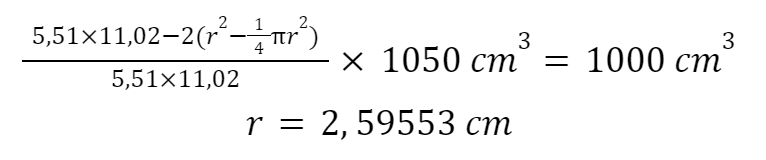Difference between revisions of "Alif Raynur Muhamad"
Alif.raynur (talk | contribs) |
Alif.raynur (talk | contribs) |
||
| Line 120: | Line 120: | ||
===Geometri End Cap=== | ===Geometri End Cap=== | ||
| + | |||
| + | [[File:ContohFilletEndCaps_Alif.png|500px|thumb|middle|Contoh sederhana filleting untuk end caps]] | ||
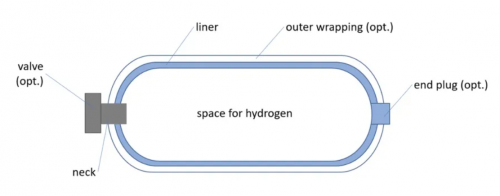
Tangki hidrogen dengan bentuk tabung murni saja akan meningkatkan risiko bahaya karena terdapat sudut yang terbentuk antara selimut dan alasnya pada kedua ujungnya, yang mana pada sudut tersebut terdapat konsentrasi stress (tegangan) yang terpusat sehingga dapat meningkatkan risiko kegagalan pada area sudut tersebut. Maka dari itu, dibentuklah end caps dengan metode filleting pada kedua ujung tangki agar stress yang disebabkan oleh tekanan dari gas hidrogen tersebut dapat lebih terdistribusi ke seluruh bagian tangki. | Tangki hidrogen dengan bentuk tabung murni saja akan meningkatkan risiko bahaya karena terdapat sudut yang terbentuk antara selimut dan alasnya pada kedua ujungnya, yang mana pada sudut tersebut terdapat konsentrasi stress (tegangan) yang terpusat sehingga dapat meningkatkan risiko kegagalan pada area sudut tersebut. Maka dari itu, dibentuklah end caps dengan metode filleting pada kedua ujung tangki agar stress yang disebabkan oleh tekanan dari gas hidrogen tersebut dapat lebih terdistribusi ke seluruh bagian tangki. | ||
| Line 126: | Line 128: | ||
[[File:EndCapsFilletRadius_Alif.png|center]] | [[File:EndCapsFilletRadius_Alif.png|center]] | ||
| + | |||
| + | Hasil perhitungan di atas menunjukkan bahwa radius fillet yang diperoleh untuk end caps pada ujung tangki adalah '''2,59553 cm'''. | ||
Revision as of 06:55, 5 June 2023
Contents
Introduction
Pagi Mesin!
Assalamu'alaikum Wr. Wb.
Halo semua! Perkenalkan, saya Alif Raynur Muhamad, akrab dipanggil Alif, dengan NPM 2106706930. Saya adalah mahasiswa semester 4 dengan Program Studi S1 Teknik Mesin angkatan 2021. Berikut adalah resume-resume saya selama pembelajaran di kelas Metode Numerik 01.
"Do your best, let God do the rest." Kalimat tersebut merupakan motto hidup saya agar selalu memaknai consciousness dalam setiap hal yang saya lakukan.
Factors & Processes Involved in Designing & Optimizing a Pressurized Hydrogen Storage
Specifications
- A hydrogen tank with 1 liter of volume & 8 bar of pressure
- The cost for the production is limited to IDR 500.000
Week 1 Progress (22/05 - 29/05)
- Before we dive deeper into the design & optimization phases, we must consciously understand the factors and processes involved in designing and optimizing the hydrogen storage first. ChatGPT helped me comprehensively to explain the factors and processes.
- Prompt: "Explain to me comprehensively the factors and processes in designing and optimizing a compact pressurized hydrogen storage with limited cost"
- Designing and optimizing a compact pressurized hydrogen storage system with limited cost involves considering several factors and processes. Here's a comprehensive overview of the key considerations:
- Storage Method
- Pressurized hydrogen storage involves storing hydrogen gas at high pressures. One common method is using high-pressure cylinders made of lightweight, strong materials such as carbon fiber composites or advanced metals like aluminum alloys. Other options include metal hydride storage or cryogenic liquid hydrogen storage, but we'll focus on pressurized storage for this explanation.
- Storage Capacity
- Determine the required storage capacity in terms of hydrogen mass or volume. This will depend on the application and the desired range or usage time before refueling. Consider factors such as vehicle range, energy demand, or the specific requirements of the system you're designing.
- Pressure Level
- Select an appropriate pressure level based on safety, storage efficiency, and system requirements. Higher pressures provide greater storage capacity but require stronger materials, leading to increased costs. Common pressure levels for pressurized hydrogen storage range from 350 bar (5,076 psi) to 700 bar (10,152 psi).
- Material Selection
- Choose materials that can withstand the desired pressure level while minimizing weight and cost. Carbon fiber composite materials are widely used due to their high strength-to-weight ratio, but they can be expensive. Aluminum alloys provide a good balance between strength, weight, and cost.
- Tank Design
- Optimize the tank design to maximize storage capacity while adhering to safety regulations and minimizing costs. Consider factors such as tank shape, volume, thickness, and the number of tanks required. Advanced design techniques like finite element analysis can help determine the optimal tank shape and thickness distribution.
- Safety Measures
- Ensure the storage system meets safety standards to prevent leaks, ruptures, or explosions. This involves using certified materials, incorporating pressure relief devices, and designing structural reinforcements to withstand potential accidents. Safety should always be a top priority in hydrogen storage design.
- Hydrogen Purity
- Consider the required hydrogen purity for your specific application. Depending on the end-use, such as fuel cells, certain contaminants like moisture or impurities can be detrimental. Incorporate purification systems if needed, which may increase the overall system cost.
- Refueling Considerations
- Design the storage system to facilitate efficient refueling processes. Consider aspects such as refueling time, connection mechanisms, and compatibility with refueling infrastructure. Faster and more accessible refueling can enhance the usability and practicality of hydrogen storage systems.
- Cost Optimization
- Minimize costs by considering factors such as material selection, manufacturing processes, and economies of scale. Explore different manufacturing techniques, such as filament winding or automated fiber placement, to optimize production costs. Additionally, identifying cost-effective suppliers and exploring potential cost-saving innovations can help reduce overall expenses.
- System Integration
- Ensure seamless integration of the hydrogen storage system with the overall application or infrastructure. Consider factors like system weight, space requirements, and compatibility with other components or systems.
- Testing and Certification
- Perform rigorous testing to validate the design, ensure safety, and obtain necessary certifications. Test the storage system under various conditions, such as temperature, pressure, and vibration, to evaluate its performance and reliability. Compliance with relevant safety standards and certifications is essential.
- Lifecycle Considerations
- Consider the environmental impact and overall lifecycle cost of the storage system. Evaluate factors such as recyclability, energy consumption during manufacturing, and end-of-life disposal options to ensure a sustainable and cost-effective solution.
- Optimizing a compact pressurized hydrogen storage system with limited cost requires a holistic approach, considering technical, safety, economic, and environmental aspects. It is crucial to balance these factors to achieve an efficient and affordable storage solution.
Final Report of Design & Optimization of Pressurized Hydrogen Storage
Sebagai mahasiswa Teknik Mesin yang merupakan calon-calon insinyur berkualitas, kita harus melakukan pendekatan secara menyeluruh pada segala faktor yang berpengaruh dalam mendesain suatu komponen, terutama efisiensi dan optimisasi yang urgensinya sangat penting. Melalui kelas Metode Numerik dengan tugas mendesain dan mengoptimalkan pressurized hydrogen storage ini, kita dapat belajar untuk berpola pikir layaknya seorang insinyur. Secara tidak langsung, hal ini juga melatih consciousness kita.
Terdapat 3 batasan (constraints) yang menjadi acuan dalam optimisasi desain tangki hidrogen ini, yaitu geometris (geometrical constraint), kekuatan material (strength constraint), dan biaya (budget constraint). Berdasarkan diskusi yang telah dilakukan di kelas bersama teman-teman pada pekan lalu, material yang akan digunakan pada pembuatan tangki hidrogen ini adalah AISI 316 austenitic stainless steel. Austenitic stainless steel juga menjadi salah satu pilihan utama untuk pabrikan-pabrikan pembuat industrial hydrogen storage. Hal ini menandakan juga bahwa austenitic stainless steel sudah teruji secara ketersediaan, durabilitas, kekuatan, machinability, dan sebagainya.
Batasan Geometris (Geometrical Constraint)
Geometri Dasar (Base)
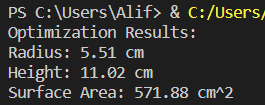
Ukuran menjadi batasan yang paling utama dalam mendesain tangki hidrogen ini. Optimisasi yang dilakukan adalah membuat surface area seminimal mungkin agar biaya material juga semakin minimum, tetapi tetap dengan volume 1 liter. Namun, karena nanti terdapat reduksi volume akibat end caps, batasan volume pada coding kali ini dibesarkan sedikit menjadi 1,050 liter atau 1050 cm^3. Pada optimisasi geometris ini, dilakukan coding menggunakan Python dengan library NumPy dan SciPy.
Berikut adalah code beserta hasilnya:
import numpy as np
from scipy.optimize import minimize
def objective(x):
# x[0] represents the radius, x[1] represents the height
radius = x[0]
height = x[1]
# Calculate the surface area of the cylindrical structure
surface_area = 2 * np.pi * radius * (radius + height)
return surface_area
def constraint(x):
# x[0] represents the radius, x[1] represents the height
radius = x[0]
height = x[1]
# Calculate the internal volume of the cylindrical structure
volume = np.pi * radius**2 * height
# Return the difference between the volume and the desired value (1050 cubic centimeters)
return volume - 1050
# Initial guess for the radius and height
x0 = [1.0, 10.0]
# Define the bounds for the variables (radius and height)
bounds = [(0, None), (0, None)]
# Define the constraint dictionary
constraint_dict = {'type': 'eq', 'fun': constraint}
# Use the minimize function to optimize the objective function subject to the constraint
result = minimize(objective, x0, method='SLSQP', bounds=bounds, constraints=constraint_dict)
# Print the optimized results
print("Optimization Results:")
print("Radius: {:.2f} cm".format(result.x[0]))
print("Height: {:.2f} cm".format(result.x[1]))
print("Surface Area: {:.2f} cm^2".format(result.fun))Berdasarkan hasil coding, dapat diketahui bahwa dengan luas area permukaan seminimal mungkin, ukuran tangki tabung yang optimal adalah dengan rasio tinggi:radius = 2:1, dalam hal ini tinggi tabung (h) adalah 11,02 cm dan radiusnya (r) adalah 5,51 cm. Dengan ukuran ini, luas area permukaan yang diperoleh adalah 571,88 cm^2.
Geometri End Cap
Tangki hidrogen dengan bentuk tabung murni saja akan meningkatkan risiko bahaya karena terdapat sudut yang terbentuk antara selimut dan alasnya pada kedua ujungnya, yang mana pada sudut tersebut terdapat konsentrasi stress (tegangan) yang terpusat sehingga dapat meningkatkan risiko kegagalan pada area sudut tersebut. Maka dari itu, dibentuklah end caps dengan metode filleting pada kedua ujung tangki agar stress yang disebabkan oleh tekanan dari gas hidrogen tersebut dapat lebih terdistribusi ke seluruh bagian tangki.
Namun, pembentukan end caps ini tentunya mengurangi volume dari tangki hidrogen. Oleh sebab itu, geometri dasar tangki dibuat dengan volume yang lebih banyak seperti di atas, yaitu 1050 cm^3 agar pengurangan volume akibat end caps ini tidak sampai kurang dari batasan 1 liter yang telah ditetapkan. Luas area proporsional dengan volume sehingga dapat dilakukan perhitungan perbandingan. Melalui perhitungan ini, dapat dicari radius dari fillet.
Hasil perhitungan di atas menunjukkan bahwa radius fillet yang diperoleh untuk end caps pada ujung tangki adalah 2,59553 cm.